Electricity is a branch of physics that deals with the study of phenomena associated with stationary or moving electric charges. 

Therefore, the various manifestations of electricity are the result of the accumulation or motion of electrons.

Electricity is classified into two types. They are Static Electricity and Current Electricity. (Scroll down to continue …)

Study Tools

Audio, Visual & Digital Content

ELECTRICITY

Static Electricity

Static Electricity is a branch of physics that deals with the study of phenomena associated with stationary electric charges.

Current Electricity.

Current Electricity is a branch of physics that deals with the study of phenomena associated with moving electric charges.

Electric Charge

Electric charge is a fundamental property of matter. 

Though we can’t say what is charge with certainty, we can study the properties and behaviour of charge. 

Charge is defined as the property associated with matter due to which it produces and experiences electrical and magnetic effects.

The electric charge is caused by the elementary particles, electrons and protons.

Protons possess positive charge, electrons possess negative charge and Neutrons do not possess any charge. 

Laws of Electric Charges:

Similar electric charges repel each other 

Dissimilar (opposite) electric charges attract each other.

Conductors And Insulators

Conductors are the materials in which electrons move freely. 

Example: All metals.

Insulators are the materials which do not have any free electrons to move. 

Example: Wood and plastic.

Electric Circuit:

The path of flow of current is known as electric circuit.

Electric Potential Energy 

Electric potential energy of a group of charges is defined as the amount of work done in bringing the charges to their respective positions in the system.

Electric Potential At A Point

The electric potential at a point, in an electric field, is defined as the amount of work done in moving a unit + ve charge from infinity to that point, without acceleration or without a change in K.E., against the electric force due to the electric field.

The potential at a point is given by the expression V = W/q

The S.I Unit of potential is mathematically written as 1 volt = 1 joule/1 coulomb.

Potential is a scalar quantity, therefore it is added algebraically.

For a positively charged body, potential is positive and for a negatively charged body potential is negative.

Electric current flows through a conductor only if  there is a potential difference across its ends.

Work done in moving a charge in the electric field of another charge is given by: 

W = Vq

More is the charge on a body, the more is its potential due to it.

Electric current flows through a conductor only if there is a potential difference across its ends.

Positive charge flows from a body at higher potential to a body at lower potential and negative charge flows from a body at a lower potential to a body at higher potential.

Potential difference 

The work done in moving a unit positive charge from one point to another is known as Potential Difference between those points.

Example

The work done in moving a unit positive charge from point A to another point B is known as Potential Difference between the  points A and B.

SI Unit: volt

The unit of potential difference is volt (V). 

Volt

In other words, Volt is defined as the potential difference between two points, if 1 Joule of work is done in moving 1 coulomb charge from one point to another.

 Potential difference between two points across a conductor is measured by using a voltmeter.

Voltmeter is always connected in parallel to the points across which potential difference is to be measured.

Battery:

Battery is an arrangement that creates a constant potential difference between its terminals.

Battery is defined as a combination of a number of cells in series.

Electric Current

The literary meaning of Electric Current is flow of electric charge.

Definition

Electric current is defined as the amount of charge passing a cross section of conductor per a unit time (second in SI Units).

Electric current is expressed mathematically in terms of rate of flow of charges as:

Electric Current =(Net Charge, Q)/(Time,t)

i =n.et , Where n = number of electrons, e = charge of one electron, t= time taken to flow,

Q = charge through the crosssection of the conductor.

The SI unit of electric current is Ampere (A).

• Direction of electric current is the same as the direction of positive charges But it is opposite to the direction of flow of negative charges.

Ohm’s Law

Experiment:

Potential difference, V between two points at a constant temperature is directly proportional to the current, I.

V ∝ I

⇒ V = lR

Where, R is a constant termed as Electric Resistance. 

The SI unit of resistance is ohm (Ω)

Q.1. State Ohm’s law. How can it be verified?

Answer: It states “Physical conditions’ remaining same, the current flowing through a conductor is directly proportional to the potential difference across its two ends”. 

i.e., V∞ I

or

V = IR, where, R is the constant of proportionality.

R is called the electrical resistance or resistance of the conductor.

Verification: 

V∞ I or V = IR, where the constant of proportionality R is called the electrical resistance or resistance of the conductor.

The following circuit diagram is used to verify Ohm’s law. 

Take a few cells; connect one cell across a nichrome wire AB, along with an ammeter and a voltmeter as shown in figure. Note the voltage and the current from the voltmeter and the

ammeter. 

Now, connect two cells and again note the voltage and the current. Repeat the procedure for three cells and four cells. Calculate the ratio for each set. 

You will find the ratio is nearly the same in all cases. If a graph of current against voltage is plotted, it will turn to be a straight line as shown in figure. This shows that the current is directly proportional to the potential difference.

Laws of Electric Resistance

     Or

Factors Affecting Resistance 

Resistance is directly proportional to length of conductor.

• Resistance is inversely proportional to the area of cross-section.
• Resistance is directly proportional to the temperature.
• Depends on the nature of the material. This is determined by the resistivity of material.

Laws of Electric Resistance

The resistance of any substance depends on the following factors,

Length of the substance.

Cross sectional area of the substance.

The nature of material of the substance.

Temperature of the substance.

There are mainly four (4) laws of resistance from which the resistivity or specific resistance of any substance can easily be determined.

The resistance of a substance is directly proportional to the length of the substance. Electric resistance, R of a substance is written as

Where L is the length of the substance.

The resistance of a substance is inversely proportional to the cross-sectional area of the substance. Electrical resistance R of a substance is 

Where A is the cross-sectional area of the substance.

Resistivity

Combining these two laws we get,

Where, ρ (rho) is the proportionality constant and known as resistivity or specific resistance of the material of the conductor or substance. 

Now if we put L = 1 and A = 1 in the equation, we get, R = ρ. 

That means resistance of a material of unit length having unit cross – sectional area is equal to its resistivity or specific resistance. 

Resistivity of a material can alternatively be defined as the electrical resistance between opposite faces of a cube of unit volume of that material.

Unit of Resistivity

The unit of resistivity can be easily determined form its equation

The unit of resistivity is Ω – m in the MKS system and Ω – cm in the CGS system and 1 Ω – m = 100 Ω – cm.

Resistivity

Resistivity is the property of the material. It does depend on the length and area of the conductor.

Resistance = (Resistivity) x  (Length of Conductor) / (Cross Sectional Area)

The SI unit of resistivity is ohm-metre.

• Resistivity of metals varies from 10^-8 to 10^-6.
• Resistivity of insulators varies from 10¹² to 10¹⁷
• Copper and aluminium are used in electrical transmission due to their low resistivity.

Net Resistance in Resistors In Series

When several resistors are joined in series, the resistance of the combination Rs equals the sum of their individual resistances, R₁, R₂, R₃

It is mathematically expressed as: R_S = R₁ + R₂ + R₃

Thus greater than any individual resistance.

Derivation of Net Resistance of Resistors In Series

When two or more resistors are joined in series, then their total resistance is given by the formula: 

⇒ R_S = R₁ + R₂ + R₃

The current will remain the same through all resistors. 

Total voltage is given by: V = V₁ + V₂ + V₃

Voltage across each resistor is given as: V₁ = IR₁, V₂ = IR₂, V₃ = IR₃ 

⇒ V = V₁ + V₂ + V₃

But Total Voltage V = I × R, Here I = Current in electric circuit and R = Net Resistance in the circuit.

⇒ IR = IR₁ +  IR₂ +  IR₃  ⇒ IR = I(R₁ +  R₂ +  R₃) ⇒ R = R₁ +  R₂ +  R₃

Resistors In Parallel

The reciprocal of the equivalent resistance of a group of resistances joined in parallel is equal to the sum of the reciprocals of the individual resistances.

(V/Rp) = (V/R₁) + (V/R₂) + (V/R₃)

Derivation of Net Resistance of Resistors In Parallel

In this case, voltage is the same across each resistor and is equal to applied voltage. 

Total current is given as:

I = I₁ + I₂ + I₃ 

It is observed that the total current I, is equal to the sum of the separate currents through each branch of the combination.

I = I₁ + I₂ + I₃  ————– (i)

Let Rp be the equivalent resistance of the parallel combination of resistors. 

By applying Ohm’s law to the parallel combination of resistors, we have: I = V/Rp ————– (ii)

On applying Ohm’s law to each resistor, we have

I₁= V /R₁; I₂= V /R₂; and I₃= V /R₃ —————– (iii)

From Eqs. (ii) to (iii), we have

(V/Rp) = (V/R₁) + (V/R₂) + (V/R₃)

⇒ V(1/Rp) = V[(1/R₁) + (1/R₂) + (1/R₃)] 

⇒ (1/Rp) = [(1/R₁) + (1/R₂) + (1/R₃)] ————– ()

Thus, we may conclude that the reciprocal of the equivalent resistance of a group of resistances joined in parallel is equal to the sum of the reciprocals of the individual resistances.

Advantages of Parallel Combination over Series Combination:

If one component fails in series, then the complete circuit is broken and no component can work properly. Different appliances need different current, this can be met through parallel.

Heating effects of Electric Current

When charge Q moves against the potential difference V in time t, the amount of work is given by-

Joule’s Law of Heating

• Heat produced in a resistor is directly proportional to square root of current.
• It is also directly proportional to resistance for a given current.
• Also, directly proportional to time

⇒ H = l² Rt

Filament of an electric bulb is made up of tungsten because it has a very high melting point and also does not oxidise readily at a high temperature.

Electric fuse is a safety device to protect the electrical appliance from short circuits.

Electric Power

The rate at which electric energy is dissipated or consumed in an electric current. The SI unit of power is Watt.

⇒ P = Vl

⇒ P = l² R = V²/R

The commercial unit of electric energy is kilowatt hour (KWh).

Formulae:

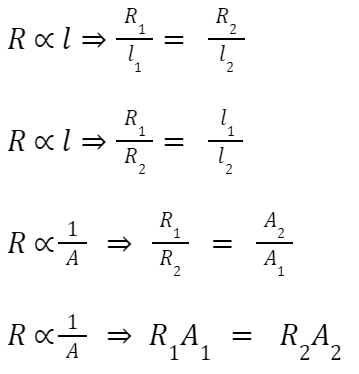
Cylindrical Conductor: