Your cart is currently empty!
Tag: Chapter Level
Chapter level course material
MENSURATION | Study
ALGEBRA | Study
KNOWING OUR NUMBERS | Study
BASIC GEOMETRICAL IDEAS | Study
UNDERSTANDING ELEMENTARY SHAPES | Study
CBSE 10 | Science – Study – Free
MENSURATION | Study
Pre-Requisires
Test & Enrich
English Version Mensuration | Speed Notes
Notes For Quick Recap
Perimeter is the length of the boundary of the geometric shape.
In other words the distance covered along theboundary forming aclosed figure whenyou go round the figure once.
(a) Perimeter of arectangle = 2 × (length + breadth) (b) Perimeter of a square = 4 × length ofits side. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
Mensuration
Perimeter is the length of the boundary of the geometric shape.
In other words the distance covered along theboundary forming aclosed figure whenyou go round the figure once.
(a) Perimeter of arectangle = 2 × (length + breadth)
(b) Perimeter of a square = 4 × length ofits side
(c) Perimeter of anequilateral triangle =3 × length of a side
(d) Perimeter of a regular pentagon has five equal sides = 5 × length of a sides Figures in which all sides and angles are equal are called regular closed figures.
The amount of surface enclosed by a closed figure is called its area. To calculate the area of a figure using a squared paper, the following conventions are adopted :
(a) Ignore portions ofthe area thatare less thanhalf a square.
(b) If more than half a square is in a region. Count it as one square
(c) If exactly half the square is counted, take its area as
Area of a rectangle = length × breadth Area of a square = side × side
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments
ALGEBRA | Study
Pre-Requisires
Test & Enrich
English Version Algebra | Speed Notes
Notes For Quick Recap
Algebra: A generalization of arithmetic in which letters representing numbers are combined according to the rules of arithmetic.
We looked at patterns of making letters and other shapes using matchsticks.
We learnt how to write the general relation between the number of matchsticks required for repeating a given shape. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
The number of times a given shape is repeated varies;
it takes on values 1,2,3, It is a variable, denoted by some letter like n.
A variable takes on different values, its value is not fixed.
The length of a square can have any value.
It is a variable.
But the number of angles of a triangle has a fixed value 3.
It is not a variable.
We may use any letter a, b, c … x, y, z etc., to show a variable.
A variable allows us to express relations in any practical situation.
Variables are numbers, although their valueis not fixed.
We can do the operations ofaddition, subtraction, multiplication and division on them just as in the case of fixed numbers.
Using different operations we can form expressions with variables like x –3, x +3, 2n, 5m, 3p, 2y + 3, 3l – 5, etc.
Variables allow us to express many common rules in both geometry and arithmetic in a general way.
For example, the rule that the sum of two numbers remains the same if the order in which thenumbers are taken is reversed canbe
expressed as a + b = b +a.
Here, the variables a and b stand for any number, 1, 32, 1000– 7, – 20, etc.
An equation is a condition on a variable.
It is expressed by saying that an expression with avariable is equal to a fixed number, e.g. x– 3 =10.
An equation has twosides, LHS and RHS, between them is the equal (=) sign.
Solution of an Equation: The value ofthe variable inan equation which satisfies the equation.
For getting thesolution of anequation, one method is the trial and error method.
In this method, we give somevalue to the variable and check whether it satisfies the equation.
We go on giving this way different values to the variable until we find the right which satisfies the equation.
The LHS of an equation is equal to its RHS only for a definite value of the variable in the equation.
We say that this definite value of the variable satisfies the equation.
This value itself is called the solution of the equation.
For getting the solution of an equation, one method is the trial and error method.
In this method, we give some value to the variable and check whether it satisfies the equation.
We goon giving this way different values to the variable` until we find the right value which satisfies the equation.
Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments
KNOWING OUR NUMBERS | Study
Pre-Requisires
Test & Enrich
English Version Whole Numbers | Speed Notes
Notes For Quick Recap
Whole Numbers The numbers 1,2, 3, ……which we use for counting are known as natural numbers. If you add 1 to a natural number, we get its successor. If you subtract 1 from a natural number, you get its predecessor. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
Every natural number has a successor. Every natural number except 1 has a predecessor.
Whole Numbers
Whole numbers are formed by adding zero to the collection of natural numbers. Hence, the set of whole numbers includes 0, 1, 2, 3, and so on.
Key Properties of Whole Numbers:
- Successors and Predecessors:
- Every whole number has a successor. For example:
- The successor of 0 is 1.
- The successor of 1 is 2.
- The successor of 2 is 3.
- Every whole number except zero has a predecessor. For example:
- The predecessor of 1 is 0.
- The predecessor of 2 is 1.
- The predecessor of 3 is 2.
- Every whole number has a successor. For example:
- Relationship with Natural Numbers:
- All natural numbers (1, 2, 3, …) are whole numbers, but not all whole numbers are natural numbers since whole numbers include 0.
- Number Line Representation:
Whole Number Line - To visualize whole numbers, we can draw a number line starting from 0:
- Mark points at equal intervals to the right: 0, 1, 2, 3, …
- This number line allows us to carry out operations:
- Addition: Moving to the right (e.g., 1 + 2 = 3).
- Subtraction: Moving to the left (e.g., 3 – 1 = 2).
- Multiplication: Making equal jumps (e.g., 2 × 3 means jumping twice the distance of 2, reaching 6).
- Division: Although division can be tricky, it involves partitioning. For example, 6 ÷ 2 means splitting 6 into 2 equal parts, resulting in 3.
Closure Properties:
- Adding two whole numbers always results in a whole number:
- Examples:
- 2 + 3 = 5
- 0 + 4 = 4
- 1 + 1 = 2
- Examples:
- Multiplying two whole numbers also results in a whole number:
- Examples:
- 2 × 3 = 6
- 0 × 5 = 0
- 1 × 4 = 4
- Examples:
- Whole numbers are closed under subtraction only if the result is non-negative:
- Examples:
- 2 – 1 = 1
- 5 – 3 = 2
- 3 – 3 = 0
- Yet, if the result is negative, they are not closed under subtraction:
- Example: 2 – 3 = -1 (not a whole number).
- So, the whole numbers are not not closed under subtraction.
- Examples:
- Division by whole numbers is defined only when the divisor is not zero, and the result is a whole number:
- Examples:
- 6 ÷ 2 = 3
- 8 ÷ 4 = 2
- 0 ÷ 5 = 0
- Division by zero is undefined (e.g., 5 ÷ 0).
- So, the whole numbers are not not closed under division.
- Examples:
Identity Elements:
- Zero acts as the identity for addition:
- Example: 5 + 0 = 5.
- The whole number 1 acts as the identity for multiplication:
- Example: 3 × 1 = 3.
Commutative and Associative Properties:
- Addition is commutative:
- Examples:
- 2 + 3 = 3 + 2
- 1 + 4 = 4 + 1
- 0 + 5 = 5 + 0
- Examples:
- Multiplication is also commutative:
- Examples:
- 2 × 3 = 3 × 2
- 1 × 4 = 4 × 1
- 0 × 5 = 5 × 0
- Examples:
- Both addition and multiplication are associative:
- Examples for addition:
- (1 + 2) + 3 = 1 + (2 + 3)
- (0 + 4) + 1 = 0 + (4 + 1)
- (2 + 2) + 2 = 2 + (2 + 2)
- Examples for multiplication:
- (1 × 2) × 3 = 1 × (2 × 3)
- (0 × 4) × 1 = 0 × (4 × 1)
- (2 × 2) × 2 = 2 × (2 × 2)
- Examples for addition:
Distributive Property:
- Multiplication distributes over addition:
- Example: 2 × (3 + 4) = 2 × 3 + 2 × 4.
Understanding these properties helps simplify calculations. It enhances our grasp of numerical patterns. These patterns are not only interesting but also practical for mental math.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments
- Successors and Predecessors:
PLAYING WITH NUMBERS | Study
Pre-Requisires
Test & Enrich
English Version PLAYING WITH NUMBERS | Speed Notes
Notes For Quick Recap
We have discussed multiples, divisors, factors and have seenhow to identify factors and multiples. We have discussed and discovered thefollowing: (a) A factor of a number is an exactdivisor of thatnumber. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
(b) Every number is a factor of itself. 1 is a factor ofevery number.
(c) Every factor ofa number isless than or equal tothe given number.
(d) Every number isa multiple ofeach of itsfactors.
(e) Every multiple ofa given number is greater thanor equal tothat number.
(f) Every number is a multiple of itself.
We have learnt that – (a) The number otherthan 1, withonly factors namely 1 and thenumber itself, isa prime number. Numbers that have more than two factors are called composite numbers. Number 1is neither prime nor composite.
(b) The number 2is the smallest prime number andis even. Every prime number other than 2 isodd.
(c) Two numbers withonly 1 asa common factor are called co-prime numbers.
(d) If a number is divisible byanother number thenit is divisible by each of the factors of that number.
(e) A number divisible by two co-prime numbers is divisible by their product also.
We have discussed how we can find just by looking at a number, whether it is divisible by small numbers 2,3,4,5,8,9 and 11.
We have explored the relationship between digits of thenumbers and theirdivisibility by different numbers.
(a) Divisibility by 2,5and 10 canbe seen byjust the lastdigit.
(b) Divisibility by 3and 9 ischecked by finding the sum ofall digits.
(c) Divisibility by 4 and 8is checked bythe last 2and 3 digits respectively.
(d) Divisibility of11 is checked by comparing thesum of digits at odd andeven places.
We have discovered that if twonumbers are divisible by a number then their sum and difference are also divisible by that number.
We have learnt that – (a) The Highest Common Factor (HCF) of two ormore given numbers is the highest of their common factors.
(b) The Lowest Common Multiple (LCM) of two ormore given numbers is the lowest of their common multiples.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments
BASIC GEOMETRICAL IDEAS | Study
Pre-Requisires
Test & Enrich
English Version Basic Geometrical Ideas | Speed Notes
Notes For Quick Recap
Geometry
Geometry is a branch of mathematics concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is called a 🎉geometer. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
Basic Geometrical Ideas | Speed Notes
Notes For Quick Recap
Geometry
Geometry is a branch of mathematics concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is called a 🎉geometer.
Space
Space: space refers to a set of points that form a particular type of structure.
Plane
A plane is a flat surface that extends infinitely in all directions
Point
Point is an exact position or location in space with no dimensions.
Mathematically, a point is defined as a circle with zero radius.
Since it is not possible its is represented by a very small dot.
A point is usually represented by a capital letter.
In mathematical terms, pont is a cirlce with no radius. It does mean that a very very small circle.
A point determines a location. It is usually denoted by a capital letter.
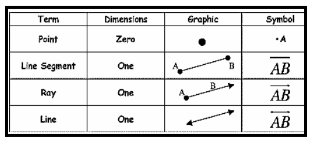
Lines And Its Types
Ray
A Ray is a straight path that stars at a point and extends infinitely in one direction.
Note: A ray is a portion of line starting at a point and extends in one direction endlessly. A ray has only one endpoint (Initial point).
Line or Straight Line
A line is a straight path that extends infinitely in two opposite directions. It can be treated as a combination of two rays starting from the same point but extending in the opposite directions.
Note: A line has no end points.
Line Segment:
A line segment is the part of a line between two points. (Segment means part).
The length of a line segment is the shortest length between two end points.
The line segment has two end points. Note: A line Segment has two endpoints. (both Initial and end points). (Scroll down to continue …).
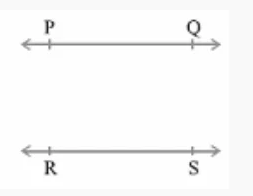
Intersecting Lines and Non-intersecting Lines
Intersecting And Parallel Lines
Parallel Lines
The lines which never cross each other at any point are called Parallel lines or Non-intersecting lines.
In other words, lines that are always the same distance apart from each other and that never meet are called Parallel lines Or Non-intersecting lines.
The perpendicular length between two lines is the distance between parallel lines.
Note: Parallel lines do not have any common point.
Intersecting Lines
The lines which cross (meet) each other at a point are called Intersecting Lines or non-parallel lines.
Intersecting lines meet at only one point.
Angle
An angle is made up of two rays starting from a common end point.
An angle leads to three divisions of a region:
On the angle, the interior of the angle and the exterior of the angle.
Curve
In geometry, a curve is a line or shape that is drawn smoothly and continuously in a plane with bends or turns.
In other words, Curve is a drawing (straight or non-straight) made without lifting the pencil may be called a curve.
Mathematicians define a curve as any shape that can be drawn without lifting the pen.
In Mathematics, A curve is a continuous and smooth line that is defined by a mathematical function or parametric equations.
Note: In this sense, a line is also a curve.
Types of Curves
Simple Or Open And Closed Curves
Cureves are two types based on intersection (crossing). They are (i) Simple or Open curve (ii) Closed curve.
Simple Curve
Simple or open curve is a curve that does not cross (intersect) itself.
Closed Curve
Closed curve is a curve that crosses (intersects) itself.
Concave And Convex Curves
Curves are of two types. They are concave curve and convex curve.
Concave Curve:
A curve is concave is a curve that curves inward, resembling a cave.
Examples:
– The interior of a circle.
– The graph of a concave function like y = -x2.
Convex Curves:
A curve is convex if it curves outward.
Examples:
– The exterior of a circle.
– The graph of a convex function like y = x2.
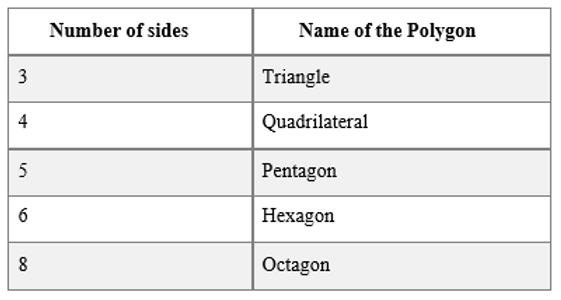
Polygon
A polygon is a simple closed figure formed by the line segments.
Types of Polygons
Polygons are classified into two types on the basis of interior angles: as (i) Convex polygon (ii) Concave polygon.
(a) Concave Polygon:
A concave polygon is a simple polygon that has at least one interior angle, that is greater than 1800 and less than 3600 (Reflex angle).
And at Least one diagonal lies outside of the closed figure.
Atlest one diagonal lies outside of the polygon.
b. Convex Polygon:
A convex polygon is a simple polygon that has at least no interior angle that is greater than 1800 and less than 3600 (Reflex angle).
And no diagonal lies outside of the closed figure.
In this case, the angles are either acute or obtuse (angle < 180 o).
Regular And Irregular Polygon
On the basis of sides, there are two types of polygons as Regular Polygon and Irregular Polygon
(a) Regular Polygon:
A convex polygon is called a regular polygon, if all its sides and angles are equal as shown in the following figures.
Each angle of a regular polygon of n-sides =
Part of Polygon
(i) Sides Of The Polygon
The line segments of a polygone are called sides of the polygon.
(ii) Adjacent Sides Of Polygon
Adjacent sides of a polygon are thesides of a polygon with a common end point.
(iii) Vertex Of Polygon
Vertext of a polygon is a point at which a pair of sides meet.
(iv) Adjacent Vertices
Adjacent vertices of polygon are the end points of the same side of the polygon.
(v) diagonal
Diagonal of a polygone is a line segment that joins the non-adjacent vertices of the polygon.
Triangle
A triangle is a three-sided polygon.
In other terms triangle is a three sided closed figure.
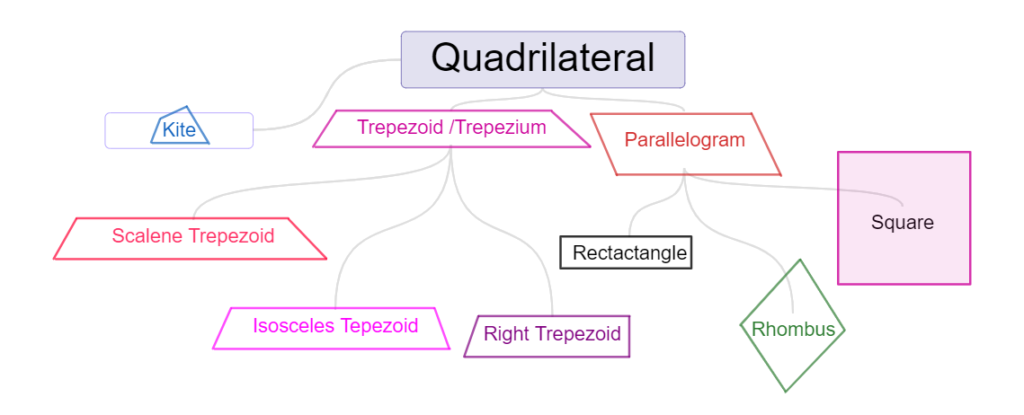
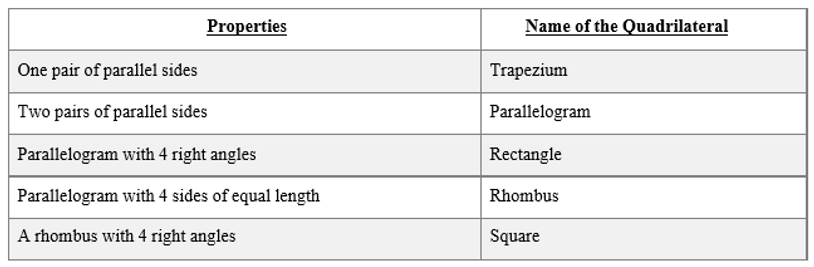
Quadrilateral
A quadrilateral is a four-sided polygon. (It shouldbe named cyclically).
In any
similar relations exist for the other three angles.
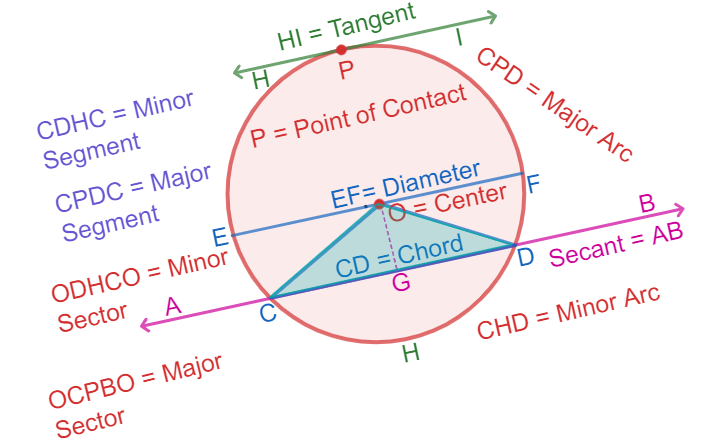
Circle And Its Parts
A circle is the path of a point moving at the same distance from a fixed point.
Centre Of Circle
Centre Of Circle is a point that is equidistant from any point on the boundary of the circle.
In other words the centre of the circles is a centre point of the circle.
Radius Of Circle
Radius of circle is the distance between the centre of the circle and any point on the boundary of the circle.
Circumference Of Circle
Circumference of circle is the length of the boundary of the circle.
Chord Of Circle
A chord of a circle is a line segment joining any two points on the circle.
A diameter is a chord passing throughthe Centre of the circle.
Sector Of Circle
A sector is the region in the interior of a circle enclosed by an arc on one side and a pair of radii on the other two sides.
Segment Of Circle
A segment of a circle is a region in the interior of the circle enclosed by an arc and a chord.
The diameter of a circle divides it into two semi-circles.
The diameter of a circle divides it into two semi-circles.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments
UNDERSTANDING ELEMENTARY SHAPES | Study
Pre-Requisires
Test & Enrich
English Version Understanding Elementary Shapes |Speed Notes
Notes For Quick Recap
The distance betweenthe end pointsof a line segment is its length. A graduatedruler and the divider are useful to compare lengthsof line segments. When a hand of a clock moves from one position to another position we have an examplefor an angle. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
One full turn of the hand is 1 revolution.
A right angle is ¼ revolution and a straight angle is ½ a revolution. We use a protractor to measure the size of an angle in degrees.
The measure of a right angle is 90° and hence that of a straight angle is 180°.
An angle is acute if its measure is smaller than that of a right angle and is obtuseif its measure is greaterthan that of a right angle and less than a straightangle.
A reflex angle is largerthan a straight angle.
Two intersecting lines are perpendicular if the anglebetween them is 90°.
The perpendicular bisector of a line segmentis a perpendicular to the line segmentthat divides it into two equal parts.
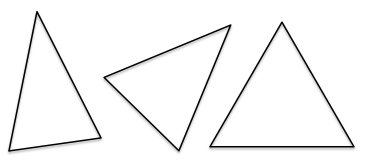
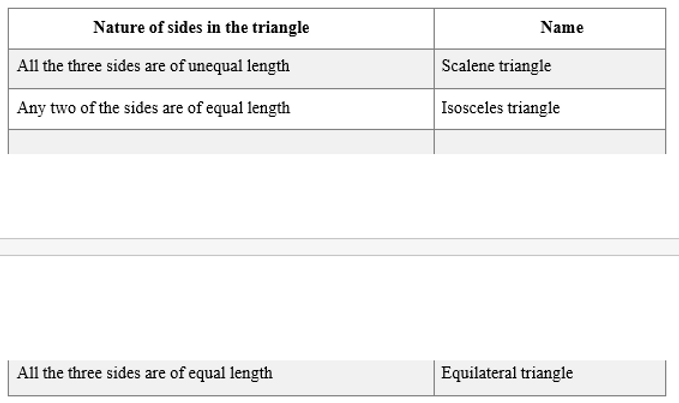
Triangles can be classified as follows based on their angles:
Triangles can be classified as follows based on the lengths of their sides:
Polygons are namedbased on theirsides.
Quadrilaterals are furtherclassified with reference to their properties.
·We see aroundus many three dimensional shapes.Cubes, cuboids, spheres,
cylinders, cones,prisms and pyramidsare some of them.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments
CBSE 10 | Science – Study – Free
Educational Tools | Full Course
Study Tools
Audio, Visual & Digital Content
Assessment Tools
Assign, Assess & Analyse
