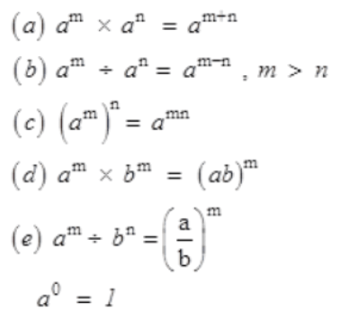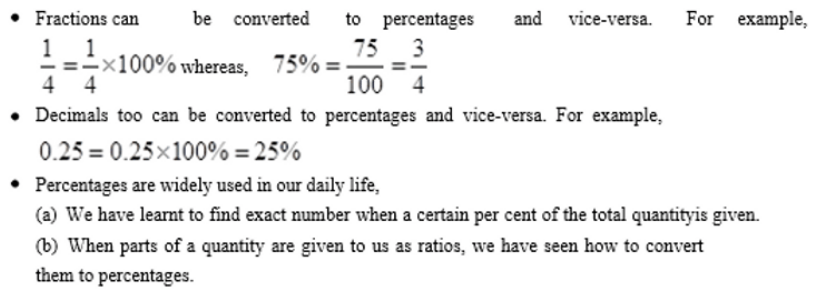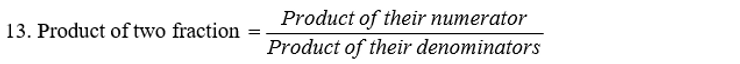Your cart is currently empty!
Tag: Class 7
Motion and Time | Study
Electric Current and its Effects | Study
Light | Study
Forests: Our Lifeline | Study
Wastewater Story | Study
CBSE 7 | Science – Study – Free
Nutrition in Plants | Study
Nutrition in Animals | Study
Heat | Study
Acids, Bases and Salts | Study
Physical and Chemical Changes | Study
Respiration in Organisms | Study
Transportation in Animals and Plants | Study
Reproduction in Plants | Study
Visualising Solid Shapes | Study
Symmetry | Study
Rational Numbers | Study
Perimeter and Area | Study
Exponents and Powers | Study
The Triangle And Its Properties | Study
Comparing Quantities | Study
Integers | Study
Fractions and Decimals | Study
Data Handling | Study

Motion and Time | Study
Pre-Requisires
Test & Enrich
English Version Motion and Time | Speed Notes
Notes For Quick Recap
Motion: The action or process of moving or being moved.
The distance moved by an object in a unit time is called its speed.
Speed of objects help us to decide which one is moving faster than the other.
The speed of an object is the distance travelled divided by the time taken to cover that distance.
Its basic unit is mere per second (m/s). (Scroll down to continue …).
Study Tools
Audio, Visual & Digital Content
Periodic events are used for the measurement of time.
Periodic motion of a pendulum has been used to make clocks and watches.
Motion of objects can be presented in pictorial form by their distance-time graphs.
The distance-time graph for the motion of an object moving with a constant speed is a straight line.
Uniform Motion ANd Non – Uniform Motion:
- Uniform motion: An object moving with a constant speed is said to be in uniform motion. The average speed is the same as the actual speed.
- Non-Uniform motion: If the speed of a moving object keeps changing, the object is said to be in non-uniform motion.
Speed: It is the distance covered by an object in a unit time.
Speed = Total distance/ Total Time
Basic unit of speed is m/s.
Distance-Time Graph: Motion of objects can be presented in pictorial form by their distance-time graphs.
The distance-time graph for the motion of an object moving with a constant speed is a straight line.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

Electric Current and its Effects | Study
Pre-Requisires
Test & Enrich
English Version Electric Current | Speed Notes
Notes For Quick Recap
Flow of electrons through a conductor.
It is convenient to represent electric components by symbols.
Using these, an electric circuit can be represented by a circuit diagram.
When an electric current flows through a wire, the wire gets heated.
It is the heating effect of current. This effect has many applications. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
Wires made from some special materials melt quickly and break when large electric currents are passed through them.
These materials are used for making electric fuses which prevent fires and damage to electric appliances.
When an electric current flows through a wire, it behaves like a magnet.
Electric Circuit: A complete pathway of the flow of electric current.
Component of Electric Circuit: Component of electric circuit are following :
Cell: Provides energy for the current to flow.
Bulb: Lights ups when an electric current flows through it.
Switch: Keeps the circuit off or on.
Connecting wires: Help to conduct the electric current and complete the circuit.
1.Heating Effect: The wire gets hot when an electric current passes through it.
This is the heating effect of the electric current.
Electric heater contains a coil of wire called element which becomes red hot when current passes through it.
The amount of heat produced in a wire depends on its material, length and thickness.
Fuse: It is a safety device which prevents damage to electric circuit.
It is made by inserting a short wire into porcelain or insulating material.
MCB: Stands for Miniature Circuit Breakers. These are switches which automatically turn off when current in a circuit exceeds the safe limit.
Effects of Electric Current:
Electric Current and its Effects
2. Magnetic Effect: When electric current passes through a wire, it behaves like a magnet. This is the magnetic effect of the electric current. This was first observed by Hans Christian Oersted.
A current carrying coil of an insulated wire wrapped around a piece of iron is called an electromagnet.
Electromagnet: An electromagnet is a coil of wire wound on a soft iron core, used to separate magnetic material from the junk. Doctors use tiny electromagnets to take out small pieces of magnetic material that have accidentally fallen in the eye.
Many toys also have electromagnets inside them.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

Light | Study
Pre-Requisires
Test & Enrich
English Version Light | Speed Notes
Notes For Quick Recap
Light: It is the natural agent that stimulates sight and makes things visible.
Light travels along straight line.
Any polished or a shining surface acts as a mirror.
An image which can be obtained on a screen is called a real image. (Scroll down to continue …).
Study Tools
Audio, Visual & Digital Content
It is formed by light rays that actually pass through the screen.
An image which cannot be obtained on a screen is called a virtual image.
It is formed by light rays that seem to pass through the screen.
The image formed by a plane mirror is erect.
It is virtual and is of the same size as the object.
The image is at the same distance behind the mirror as the object is in front of it.
In an image formed by a mirror, the left side of the object is seen on the right side in the image, and right side of the object appears to be on the left side in the image.
A concave mirror can form a real and inverted image.
When the object is placed very close to the mirror, the image formed is virtual, erect and magnified.
A Convex mirror is the mirror that curves out; the reflecting surface is convex.
Image formed is virtual, upright and diminished. Image formed by a convex mirror is erect, virtual and smaller in size than the object.
A Concave lens is the lens that is thinner at the center than at the edges.
It is a diverging lens.
Image formed is virtual, erect and diminished.
A convex lens can form real and inverted image.
When the object is placed very close to the lens, the image formed is virtual, erect and magnified.
When used to see objects magnified, the convex lens is called a magnifying glass.
White light is composed of seven colors.
Properties of Light:
1. Rectilinear Propagation of Light: It is the property of light by which it travels in a straight line in any direction.
The direction of path in which light make a ray.
2. Reflection of Light: It is the bouncing back of light after striking the surface of an object.
Shiny smooth surfaces reflect almost all the light.
3. Dispersion: It is the phenomenon of splitting of white light into its seven colors. White
light is mixture of: Violet, Indigo, Blue, Green, Yellow, Orange and Red (VIBGYOR) colors.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

Forests: Our Lifeline | Study
Pre-Requisires
Test & Enrich
English Version Forests: Our Lifeline | Speed Notes
Notes For Quick Recap
Forest: Large area of land thickly covered with trees, bushes, etc.
We get various products from the forests around us.
Forest is a system comprising various plants, animals and micro-organisms.
In a forest, trees from the uppermost layer, followed by shrubs, the herbs to
the lowest layer of vegetation. (Scroll down to continue …).
Study Tools
Audio, Visual & Digital Content
Different layers of vegetation provide food and shelter for animals, birds and insects.
The various components of the forest are interdependent on one another.
The forest keeps on growing and changing, and can regenerate.
In the forest, there is interaction between soil, water, air and living organisms.
Forests protect the soil from erosion.
Soil helps forests to grow and regenerate.
Deforestation: Cutting down of trees is known as deforestation.
Importance of Forests:
Forests:Provide timber,
Purify air,
Provide shelter,
Prevent soil,
Absorbs noise.
Independence of Plants and Animals in Forest:
Plants and animals depends on each other to remain alive.All organisms interact with each other and their physical environment to derive and survive.
Effects of deforestation:
Amount of carbon dioxide in air will increase, resulting in the increase of earth’s temperature. (Global Warming) Animals will not get food and shelter.Soil will not hold water, which will cause floods.
Endanger lives and environment.
Conservation of Forests:
Do not allow overgrazing.Promote afforestation.
Protect wildlife.
Food Chain:
Interdependence between producers and consumers studied in form of various linkage that appears as a chain or Interdependence of
organisms which shows who eats whom.Food Web: A system of interdependent food chains used to represent various relationships in organisms.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

Wastewater Story | Study
Pre-Requisires
Test & Enrich
English Version Wastewater Story | Speed Notes
Notes For Quick Recap
Wastewater: Black-brown water which is rich in lather , mixed with oil that goes down the drains from skins, showers, toilets, laundries is called wastewater.
sewage: Wastewater is generated in homes, industries, agricultural fields and in other human activities. This is called sewage. (Scroll down to continue …).
Study Tools
Audio, Visual & Digital Content
Sewage is a liquid waste which causes water and soil pollution.
Wastewater is treated in a sewage treatment plant.
Treatment plants reduce pollutants in wastewater to a level where nature can take care of it.
Where underground sewerage systems and refuse disposal systems are not available, the low cost on-site sanitation system can be adopted.
By-products of wastewater treatment are sludge and bio gas.
Open drain system is a breeding place for flies, mosquitoes and organisms which cause diseases.
We should not defecate in the open. It is possible to have safe disposal of excreta by low cost methods.
Sewage Treatment:
Aeration: Air is bubbled through the wastewater while it is continuously stirred.
Filtration: Aerated water passes through a deep filter of layered sand, fine gravel and medium gravel.
Chlorination: Chlorine is added and mixed to the filtered water until water is clear.
Wastewater Treatment Plant (WWTP):
Wastewater passes through screens to remove large objects.
To go to a grit and sand removal tank at low speed.
Water is allowed to settle in large tank.
Floating solids are removed with skimmer.
Settled solids (sludge) are removed with scraper.
Clear water is called clarified water.
Water is then decomposed by anaerobic bacteria in a tank and air is passed.
Microbes settled at bottom as activated sludge and water from top is removed.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

CBSE 7 | Science – Study – Free
Educational Tools | Full Course
Study Tools
Audio, Visual & Digital Content
Assessment Tools
Assign, Assess & Analyse
Connnect With Us
Enrich our growing community
[ultimatemember form_id=”26482″]

Nutrition in Plants | Study
Pre-Requisires
Test & Enrich
English Version Speed Notes
Notes For Quick Recap
Nutrition: It is the mode of taking food by an organism and its utilization by the body.
Nutrients: The components of food that provide nourishment to the body.
All organisms take food and utilise it to get energy for the growth and maintenance of their bodies. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
Autotrophs: Autotrophs are the green plants which synthesise their food themselves by the process of photosynthesis.
Photosynthesis: the process of preparation of own food by the Green plants with the help of chlorophyll (found in green plants), carbon dioxide and water taken from the environment in presence of sunlight is known as photosynthesis.
Plants use simple chemical substances like carbon dioxide, water and minerals for the synthesis of food.
Chlorophyll and sunlight are the essential requirements for photosynthesis.
Complex chemical substances such as carbohydrates are the products of photosynthesis.
Solar energy is stored in the form of food in the leaves with the help of chlorophyll.
Oxygen is produced during photosynthesis.
Oxygen released in photosynthesis is utilised by living organisms for their survival.
Fungi derive nutrition from dead, decaying matter.
They are saprotrophs.
Plants like Cuscuta are parasites.
They take food from the host plant.
A few plants and all animals are dependent on others for their nutrition and are called heterotrophs.
Parasitic: Organisms that live on the body of other organisms. All parasitic plants feed on other plants as either:
Partial Parasites: Obtain some of their nutrition from the host,
Example: Painted cup
Total Parasites: Dependent completely on the host for nutrition.
Example: Mistletoe.
Nutrition in plants
Saprophytic: Organisms that obtain nutrition from dead and decaying plant and animal matter.
Mushrooms, moulds and certain types of fungi and bacteria.
Insectivorous Plants: Green plants which obtain their nourishment partly from soil and atmosphere and partly from small insects.
Example: pitcher plant, bladderwort, and venus fly trap.
Symbiosis: Mode of nutrition in which two different individuals associate with each other to fulfil their requirement of food.
Lichens found on tree trunks is the association between algae and fungus.
Algae obtains water from fungus and it in turn obtains food from algae.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

Nutrition in Animals | Study
Pre-Requisires
Test & Enrich
English Version Nutrition in Animals | Speed Notes
Notes For Quick Recap
Classification based on Eating Habits:
Herbivorous: Animals that eat plants or plant products.
Example: Cow, sheep, goat, deer, elephant, kangaroo, giraffe, etc.
Carnivorous: Animals that eat only flesh of other animals. They never eat plants.
Examples: Tiger, lizard, lion, etc.
Omnivorous: Animals consume plants as well as other animals as their food.
Examples: Bear, dog, human being, etc.
Parasites: Organisms that obtain their food from other animals either by living inside (endoparasites) or outside (ectoparasites) their body.
Examples: Tapeworm and roundworm (inside body), tick and lice (outside body).
Scavengers: Animals which feed on the remains of dead animals preyed by predators. Example: vulture, crows, jackal, etc. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
The main digestive glands which secrete digestive juices are:
- the salivary glands,
- the liver and
(iii) the pancreas.
The human digestive system consists of the alimentary canal and secretory glands.
It consists of:
- buccal cavity,
- oesophagus,
- stomach,
- small intestine,
- large intestine ending in rectum
- anus.
Animal nutrition includes nutrient requirement, mode of intake of food and its utilisation in the body.
The stomach wall and the wall of the small intestine also secrete digestive juices.
The modes of feeding vary in different organisms.
Nutrition is a complex process involving:
- ingestion,
- digestion,
- absorption,
- assimilation and
- egestion.
Digestion of carbohydrates, like starch, begins in the buccal cavity.
The digestion of protein starts in the stomach.
Bile secreted from the liver, the pancreatic juice from the pancreas and the digestive juice from the intestinal wall complete the digestion of all components of food in the small intestine.
The digested food is absorbed in the blood vessels from the small intestine.
The absorbed substances are transported to different parts of the body.
Water and some salts are absorbed from the undigested food in the large intestine.
The undigested and unabsorbed residues are expelled out of the body as faeces through the anus.
The grazing animals like cows, buffaloes and deer are known as ruminants.
They quickly ingest, swallow their leafy food and store it in the rumen.
Later, the food returns to the mouth and the animal chews it peacefully.
Amoeba ingests its food with the help of its false feet or pseudopodia.
The food is digested in the food vacuole.
It pushes out finger-like pseudopodia which engulf the prey.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

Heat | Study
Pre-Requisires
Test & Enrich
English Version Heat | Speed Notes
Notes For Quick Recap
Heat: It is a form of energy, which makes any object hot or cold.
The materials which allow heat to pass through them easily are conductors of heat.
The materials which do not allow heat to pass through them easily are called insulators.
Temperature: The degree of hotness of an object is called temperature.
Heat is the cause of temperature.
Our sense of touch is not reliable to measure the temperature. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
Heat:
It is a form of energy, which makes any object hot or cold.
Temperature: The degree of hotness of an object is called temperature. Our sense of touch is not reliable to measure the temperature.
Thermometer is a device used for measuring temperatures.
Heat is the cause of temperature.
Clinical thermometer is used to measure our body temperature.
Transfer of Heat: Heat flows from a hotter object to a colder object until both objects reach the same temperature.
The heat flows from a body at a higher temperature to a body at a lower temperature.
There are three ways in which heat can flow from one object to another.
These are conduction, convection and radiation.
Conduction: It is the process by which heat is transferred from the hotter end to the colder and end of an object.
Convection: It is the flow of heat through a fluid from places of higher temperature to places of lower temperature by movement of the fluid itself.
Radiation: It is the mode of transfer of heat in which energy is directly transferred from one place to another.
It does not need any material medium.
Dark-coloured objects absorb radiation better than the light-coloured objects.
That is the reason we feel more comfortable in light-coloured clothes in the summer.
Woollen clothes keep us warm during winter.
It is so because wool is a poor conductor of heat and it has air trapped in between the fibres.
Thermometer:
Thermometer is a device used for measuring temperatures.
Clinical thermometer:
Clinical thermometer is used to measure our body temperature.
A thermometer used to measure the temperature of our body is called a clinical thermometer.
For other purposes, we use the laboratory thermometers.
The range of these thermometers is usually from –10°C to 110°C.
The normal temperature of the human body is 37°C.
It consists of a long, narrow, uniform glass tube with a bulb containing mercury at one end.
There is a kink near the bulb.
The range of clinical thermometer is from 35°C to 42°C. (Or from 94°F to 108°F).
Laboratory Thermometer:
Laboratory Thermometer: It is a thermometer used to measure the temperature of objects other than our body.
It consists of a column of mercury enclosed in a glass casing.
The column is continuous without any kink.
It measures a range of temperature from -10˚C to 110˚C.
Sea Breeze:
Sea Breeze: Durign the day, the land heats up faster than the sea.
Warm air above the land rises and cold air from sea takes its place.
Warm air from the land moves towards the sea to compele the cycle.
This produces a sea breeze from the sea to the land.
Land Breeze:
Land Breeze: At night the land cools faster than sea.
The warm air above the sea rises.
This warm air is replaced by colder air from the land producing a land breeze.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

Acids, Bases and Salts | Study
Pre-Requisires
Test & Enrich
English Version Acids, Bases and Salts | Speed Notes
Notes For Quick Recap
There are three types of Substances: Acids, Bases and Salts
Acids: Acids are sour in taste. They are corrosive in nature.
A concentrated acid cuts through clothes and eats away the wool.
If it falls on the skin, it can cause burns.
They are good conductors of electricity, as they allow the passage of electric current through them. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
Types of Acids:
(i) Mineral Acids: These are acids prepared from minerals present in the earth’s crust.
(ii) Organic Acids: These are acids produced by plants and animals (except hydrochloric acid).
- Weak Acids: These do not dissociate completely in solution.
- Examples: tartaric acid, lactic acid.
- Strong Acids: These dissociate completely in solution. Example: nitric acid, sulphuric acid.
Neutralization: It is the reaction between an acid and a base which results in formation of salt and water.
Acid + Base ———-> Salt + Water
Example: HCl + NaOH ———-> NaCl + H2O
Neutralisation in Everyday Life:
Indigestion: Too much acid in stomach causes indigestion. It is neutralized by taking an antacid like milk of magnesia.
Ant sting: When an ant bites, it injects formic acid into the skin. The effect is neutralized by rubbing moist baking soda (sodium hydrogen carbonate) or calamine (containing zinc carbonate).
(iii) Soil treatment: When the soil is too acidic, it is neutralized by treating with
quicklime (calcium oxide) or slaked lime (calcium hydroxide).
Bases: Bases are bitter in taste and soapy to touch.
Types of Bases:
- Weak Bases: These naturally produce less hydroxide ions in solution. Example: magnesium hydroxide, ammonium hydroxide.
- Strong Bases: These produce more number of hydroxide ions on dissolving in water. Example: Sodium hydroxide(NaOH), Potassium hydroxide (KOH)
Substances which are neither acidic nor basic are called neutral.
An acid and a base neutralise each other and form a salt. A salt may be acidic, basic or neutral in nature.
Solutions of substances that show different colour in acidic, basic and neutral solutions are called indicators.
Indicators: It is special chemical that changes its colour to indicate the presence of a chemical substance.
It is used to confirm the presence of an acid, a base or a neutral solution.
Classification of Indicators:
Natural Indicators:
- Litmus: It is extracted from lichens. It is available in the form of strips of paper or in the form of a solution.· Acid turns blue litmus red. Bases turn red litmus blue.
- Turmeric: It remains yellow in neutral and acidic solutions but turns red in alkaline solutions.
- China rose: It turns acidic solutions to dark pink (magenta) and basic solution to green.
- Red cabbage: It turns acidic solutions to red and basic solutions to blue.
Other Indicators:
- Methyl Orange: It gives pinkish red colour with acidic solutions and yellow colour with bases.
- Phenolphthalein: It is an acid-base indicator. It is colourless in acidic solutions but turns pink in alkali solutions.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

Physical and Chemical Changes | Study
Pre-Requisires
Test & Enrich
English Version Physical And Chemical Changes | Speed Notes
Notes For Quick Recap
Changes can be of two types, physical and chemical.
Physical changes are changes in the physical properties of substances.
Due to physical chages new substances are not formed.
Physical changes may be reversible.
Examples: crushing a can, glowing of an electric bulb, tearing of paper, mixing of sand and water. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
Chemical Changes are changes in which the composition and chemical properties of the substance get changed.
In chemical changes new substances are produced. The most of the chemical changes are irreversible and permanent.
Note: Some chemical changes are reversible, known as reversible chemical changes.
Example: burning of a candle, formation of curd from milk, ripening of fruits.
Some Chemical Reactions in daily life:
Rusting of Iron: Rusting is the process in which iron turns into iron oxide.
It happens when iron comes into contact with water and oxygen. The process is a type of corrosion that occurs easily under natural conditions.
Prevention of Rusting:
- By Painting
- By Oiling and greasing
- By Chromium plating
- By Galvanizing
- By Alloying
Cooking of food: Cooking causes breakdown of complex molecules of carbohydrates, fats and proteins into smaller molecules.
It is regarded as a decomposition reaction.
Cooked food is easier to digest than uncooked food.
3. Decay of Organic Substances: Microorganisms like fungi and bacteria produce enzymes which break down complex organic compounds into smaller substances.
It is also regarded as a decomposition reaction.
Some substances can be obtained in pure state from their solutions by crystallization.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

Respiration in Organisms | Study
Pre-Requisires
Test & Enrich
English Version Respiration in Organisms | Speed Notes
Notes For Quick Recap
Respiration is essential for survival of living organisms.
It releases energy from the food.
The oxygen we inhale is used to breakdown glucose into carbon dioxide and water.
Energy is released in this process.
The breakdown of glucose occurs in the cells of an organism (cellular respiration). (ScrollScroll down to continue…)
Study Tools
Audio, Visual & Digital Content
During heavy exercise when the supply of oxygen to our muscle cells is insufficient, food breakdown is by anaerobic respiration (without oxygen)
Types of Respiration:
External respiration also known as breathing refers to a process of inhaling oxygen from the air into the lungs and expelling carbon dioxide from the lungs to the air.
Exchange of gases both in and out of the blood occurs simultaneously.
Internal Respiration: Process in which food is broken down in body cells.
Internal respiration is further classified into two types as aerobic respiration and anaerobic respiration
(a) Aerobic Respiration: Aerobic respiration takes place in the presence of oxygen. Carbon dioxide and water are the end products of aerobic respiration. respiration happens in most of the organisms.
(b) Anaerobic Respiration: Anaerobic respiration takes place in the absence of oxygen.
Anaerobic respiration usually happens in most of the microbes.
Alcohol and carbon dioxide are formed at the end of anaerobic respiration.
In some cases, lactic acid is formed at the end of anaerobic respiration.
Respiration in Plants: Leaves have pores called stomata for gaseous exchange by diffusion.
Stems have openings called lenticels for gaseous exchange by diffusion.
Roots have stomatal pores for gaseous exchange of oxygen dissolved in soil water.
Respiration in Animals: Respiration in animals vary according to their character like:
Earthworm: Earthworms respire through their skin.
Insect: Insects respire through entire body surface.
Fish: Fishes respire through their gills.
Frogs: Frogs respire through their thin, moist and smooth skin when in water and by lungs when on the land.
Respiration in Humans: Inhaled air passes through nostrils into nasal cavity and then into lungs through windpipe.
Breathing is a part of the process of respiration during which an organism takes in the oxygen-rich air and gives out air rich in carbon dioxide.
The respiratory organs for the exchange of gases vary in different organisms.
During inhalation, our lungs expand and then come back to the original state as the air moves out during exhalation.
Increased physical activity enhances the rate of breathing.
In animals like cow, buffalo, dog and cat the respiratory organs and the process of breathing are similar to those in humans.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

Transportation in Animals and Plants | Study
Pre-Requisires
Test & Enrich
English Version Transportation in Animals and Plants | Speed Notes
Notes For Quick Recap
Transportation in animals:
In most of the animals the blood that circulates in the body distributes food and oxygen to different cells of the body.
It also carries waste products from different parts of the body for excretion.
Circulatory system consists of the heart and blood vessels.
In humans, blood flows through arteries and veins and the heart acts as a pumping organ. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
Blood is the fluid which flows in blood vessels.
It transport substance like digested food from the small intestine to the other parts of the body.
Blood consists of plasma, RBC, WBC and platelets.
Blood is red due to the presence of a red pigment, haemoglobin which binds with oxygen and transport oxygen to various parts.
Heart rate:The human heart beats about 70 to 80 times per minute in an adult person. This is called heart rate.
Arteries carry blood from the heart to all parts of the body.
Veins carry blood from all parts of the body back to the heart.
excretion: Removal of waste products from the body is called excretion.
Excretory system of humans consists of two kidneys, two ureters, a urinary bladder, and urethra.
Salts and urea are removed along with water as sweat.
Fish excrete waste substances such as ammonia which directly dissolve in water.
Birds, insects and lizard excrete uric acid in semi-solid form.
Transportation in plants :
Water and mineral nutrients are absorbed by roots from the soil.
xylem: Nutrients are transported along with water to the entire plant via the vascular tissue called xylem.
phloem: The vascular tissue for the transport of food to the various parts of the plant is phloem.
A lot of water is lost by plants in the form of vapour through stomata during transpiration.
Transpiration generates a force which pulls up water absorbed by the roots from the soil, to reach the stem and leaves.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

Reproduction in Plants | Study
Pre-Requisires
Test & Enrich
English Version Reproduction in Plants | Speed Notes
Notes For Quick Recap
All organisms multiply or reproduce offspring of their own kind.
In plants there are two modes of reproduction, namely (a) Asexual reproduction and (b) Sexual reproduction.
There are several methods of asexual reproduction such as fragmentation, budding, spore formation and vegetative propagation.
Sexual reproduction involves the fusion of male and female gametes. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
In vegetative propagation new plants are produced from different vegetative parts such as leaves, stems and roots.
Flower is the reproductive part of a plant.
A flower may be unisexual with either the male or the female reproductive parts.
A bisexual flower has both the male and the female reproductive parts.
The male gametes are found inside the pollen grains and female gametes are found in the ovule.
Pollination is the process of transfer of pollen grains from the anther of one flower to the stigma of the same or another flower.
Pollination is of two types, self-pollination and cross-pollination.
In self-pollination, pollen grains are transferred from the anther to the stigma of the same flower.
In cross-pollination, pollen grains are transferred from the anther of one flower to the stigma of another flower of the same kind.
Pollination takes place in plants with the help of wind, water and insects.
The fusion of male and female gametes is called fertilization.
Fertilized egg is called zygote.
Zygote develops into an embryo.
Fruit is the mature ovary whereas ovule develops into a seed, which contains the developing embryo.
Seed dispersal is aided by wind, water and animals.
Seed dispersal helps the plants to:
- prevent overcrowding,
- avoid competition for sunlight, water and minerals
- invade new habitats.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

Visualising Solid Shapes | Study
Pre-Requisires
Test & Enrich
English Version Visualising SolidShapes | Speed Notes
Notes For Quick Recap
The circle, thesquare, the rectangle, the quadrilateral and the triangle are examples of plane figures; the cube, the cuboid, the sphere, the cylinder, the cone and the pyramid areexamples of solid shapes. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
Plane figures areof two-dimensions (2-D) and the solid shapes are of three- dimensions (3-D). The corners of a solid shape are called its vertices; theline segments ofits skeleton areits edges; and itsflat surfaces areits faces. A net is a skeleton-outline of a solid that can be folded to make it. The same solid can haveseveral types ofnets. Solid shapes can be drawn on a flat surface (like paper) realistically. We call this 2-D representation of a 3-Dsolid. Two types ofsketches of asolid are possible: (a) An oblique sketch does nothave proportional lengths. Still it conveys all important aspects of the appearance of the solid. (b) An isometric sketch is drawn on an isometric dot paper, a sample of which isgiven at theend of thisbook. In an isometric sketch of the solidthe measurements kept proportional. Visualising solidshapesis a veryuseful skill. Youshould be ableto see ‘hidden’ parts of thesolid shape. Different sections of a solid can be viewed in many ways: (a) One way is to viewby cutting or slicing the shape, whichwould result in the cross- section of thesolid. (b) Another way isby observing a 2-D shadow of a 3-Dshape. (c) A third wayis to lookat the shapefrom different angles; the front-view, theside- view and thetop view canprovide a lotof information aboutthe shape observed.
19. When a grouping symbol preceded by ‘ sign is removed or inserted, thenthe sign of eachterm of thecorresponding expression ischanged (from ‘ + ‘ to ‘−’ and from‘− ‘ to + ‘).
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

Symmetry | Study
Pre-Requisires
Test & Enrich
English Version Congruence of Triangles | Speed Notes
Notes For Quick Recap
Congruence: The relation of two objects being congruent is called congruence. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
Chapter – 7
Congruence of Triangles

SSS Congruence of two triangles: Under a given correspondence, two triangles are congruent if the three sides of the one are equal to the three corresponding sides of the other.
SAS Congruenceof two triangles: Under a given correspondence, two triangles are
congruent if two sides and the angleincluded between them in one of the triangles are equal to the corresponding sides and the angle included between them of the other triangle.
ASA Congruence of two triangles: Under a given correspondence, two triangles are congruent if two anglesand the side included betweenthem in one of the triangles are equal to the corresponding angles and the side included between them of the other triangle.
RHS Congruence of two right-angled triangles: Under a given correspondence, two right-angled triangles are congruent if the hypotenuse and a leg of one of the triangles are equal to the hypotenuse and the corresponding leg of the other triangle.
There is no such thing as AAA Congruence of two triangles: Two triangles with equal corresponding angles need not be congruent. In such a correspondence, one of them can be an enlarged copy of the other.
(They would be congruent only if they are exact copies of one another).
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

Rational Numbers | Study
Pre-Requisires
Test & Enrich
English Version Rational Numbers | Speed Notes
Notes For Quick Recap
Rational Number: A number that can be expressed in the form (Scroll down to continue …)
Audio, Visual & Digital Content



14. Every positive rational number is greater than zero.
15. Every negative rational number is less than zero.
16. The rational numbers can be represented on the number line.
Study Tools
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

Perimeter and Area | Study
Pre-Requisires
Test & Enrich
English Version Perimeter and Area | Speed Notes
Notes For Quick Recap
Perimeter is the distance around a closed figure whereasarea is the part of plane occupied by the closedfigure.
Area is the measure of the part of plane or regionenclosed by it. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
We have learnt how to find perimeter and area of a squareand rectangle earlierclass.
They are:
(a) Perimeter of a square = 4 × side
(b) Perimeter of a rectangle = 2 × (length + breadth)
(c) Area of a square = side × side
(d) Area of a rectangle = length × breadth Areaof a parallelogram = base × height

Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

Exponents and Powers | Study
Pre-Requisires
Test & Enrich
English Version Exponents and Powers Exponents | Speed Notes
Notes For Quick Recap
Exponents are used to express large numbers in shorter form to make them easy to read, understand, compare and operate upon. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
Expressing Large Numbers in the Standard Form: Any number can be expressed as a decimal number between 1.0 and 10.0 (including 1.0) multiplied by a power of 10. Such form of a number is called its standard form or scientific motion. Very large numbers are difficult to read, understand, compare and operate upon. To make all these easier, we use exponents, converting many of the large numbers in a shorter form. The following are exponential forms of some numbers?

Here, 10, 3 and 2 are the bases, whereas 4, 5 and 7 are their respective exponents. We also say, 10,000 is the 4th power of 10, 243 is the 5th power of 3, etc. Numbers in exponential form obey certain laws, which are: For any non-zero integers a and b and whole numbers m and n,

(g) (–1) even number = 1 (–1) odd number = – 1

Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

The Triangle And Its Properties | Study
Pre-Requisires
Test & Enrich
English Version Speed Notes
Notes For Quick Recap
Triangle
A closed plane figure bounded by three linesegments. The six elements of a triangle are its three angles and thethree sides. The line segment joining a vertex of a triangle to the mid point of its opposite side is called a medianof the triangle. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
Notes For Quick Recap
A closed plane figure bounded by three linesegments. The six elements of a triangle are its three angles and thethree sides. The line segment joining a vertex of a triangle to the mid point of its opposite side is called a medianof the triangle. (Scroll down to continue …)
Triangle:
A closed plane figure bounded by three line segments is called Triangle.
The six elements of a triangle are its three angles and the three sides. The line segment joining a vertex of a triangle to the midpoint of its
Median:
The opposite side is called the median of the triangle.
A triangle has three medians.
Altitude of the triangle:
The perpendicular line segment from vertex of a triangle to its opposite sides is called an altitude of the triangle.
A triangle has3 altitudes.
Type of triangle based onSides: Equilateral:
A triangle is said to be equilateral, if each one of its sides has the same length. In An equilateral triangle, each angle measures 60°.
Isosceles Triangle:
A triangle is said to be isosceles, if atleast any two of its sides are of same length. The non-equal side of an isosceles triangle is called its base; the base angles of an isosceles triangle have equal measure.
Scalene Triangle:
A triangle having all sides of different lengths. It has no two angles equal.
Property of the lengths of sides of a triangle:
The sum of the lengths of any two sides of a triangle is greater than the length of the third side. The difference between the lengths of any two sides is smaller than the length of the third side. This property is useful to know if it is possible to draw a triangle when the lengths of the three sides are known.
Types of Triangle based on Angles:
(i) Right Angled Triangle:
A triangle one of whose angles measures
(ii) Obtuse Angled Triangle:
A triangle one of whose angles measures more than
(iii) Acute Angled Triangle:
A triangle each of whose angles measures less than In a right angled triangle, the side opposite to the right angle is called the hypotenuse and the other two sides are called its legs.
Pythagoras property:
In a right-angled triangle, the square on the hypotenuse = the sum of the squares on its legs.If a triangle is not right-angled, this property does not hold good. Thisproperty is useful to decide whether a given triangle is right-angled
or not.
Exterior angle of a triangle:
An exterior angle of a triangle is formed, when a side of a triangle is produced. At each vertex, you have two ways of forming an exterior angle.
A property of exterior angles:
The measure of any exterior angle of a triangle is equal to the sum of the measures of its interior opposite angles.
The angle sum property of a triangle:
The total measure of the three angles of a triangle is 180°.
Property of the Lengths of Sides of a Triangle:
The sum of the lengths of any two sides of a triangle is always greater than the length of the third side. The difference of the lengths of any two sides of a triangle is always smaller than the length of the third side.
Important Formulas – TheTriangles and its Properties
1. A triangle is a figure made up by three line segments joining, in pairs, three non-collinear points. That is, if A, B, C are three non-collinear points, the figure formed by three line segments AB,BC and CA is called a triangle with vertices A, B, C.
2. The three line segments forming a triangle are called the sides of the triangle.
3. The three sides and three angles of a triangle are together called the six parts or elements of the triangle.
4. A triangle whose two sides are equal, is called an isosceles triangle.
5. A triangle whose all sides are equal, is called an equilateral triangle.
6. A triangle whose no two sides are equal, is called a scalene triangle.
7. A triangle whose all the angles are acute is called an acute triangle.
8. A triangle whose one of the angles is a right angle is called a right triangle.
9. A triangle whose one of the angles is an obtuse angle is called an obtuse triangle.
10. The interior of a triangle is made up of all such points P of the plane, as are enclosed by the triangle.
11. The exterior of a triangle is that part of the plane which consists of those points Q, which are neither on the triangle nor in its interior.
12. The interior of a triangle together with the triangle itself is called the triangular region.
13. The sum of the angles of a triangle is two right angles or 180°.
14. If a side of a triangle is produced, the exterior angle so formed is equal to the sum of the interior opposite angles.
15. In any triangle, an exterior angle is greater than either of the interior opposite angles.
16. The sum of any two sides of a triangle is greater than the third side.
17. In a right triangle, if a, b are the lengths of the sides and c that of the hypotenuse, then
18. If the sides of a triangle are of lengths a, b and c such that
then the triangle is right-angled and the side of length c is the hypotenuse.
19. Three positive numbers a, b, c in this order are said to form a Pythagorean triplet, if
Triplets (3, 4, 5) (5, 12,13), (8, 15, 17), (7,24, 25) and (12, 35,37) are somePythagorean triples.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

Comparing Quantities | Study
Pre-Requisires
Test & Enrich
English Version Speed Notes
Notes For Quick Recap
Comparing Quantities: Weare often requiredto compare two quantities, in our dailylife. They may be heights, weights, salaries, marks etc. To compare two quantities, their units must be the same.
We are often required to compare two quantities in our daily life. They may be heights, weights,salaries, marks etc. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
While comparing heights of two persons with heights150 cm and 75 cm, we write it as the ratio 150 : 75 or 2 : 1.
Ratio: A ratio compares two quantities using a particular operation.
Percentage: Percentage are numerators of fractions with denominator 100. Percent is represent by the symbol% and means hundredth too.
Two ratios can be compared by converting them to like fractions. If the two fractions are equal,we say the two given ratios are equivalent.
If two ratios are equivalent then the four quantities are said to be in proportion. For example, the ratios 8 : 2 and 16 : 4 are equivalent therefore 8, 2, 16 and 4 are in proportion.
A way of comparing quantities is percentage. Percentages are numerators of fractions with denominator 100. Per cent means per hundred. For example 82% marks means
82 marks out of hundred.

Percentages are widely used in our daily life,
(a) We have learnt to find exact number when a certain per cent of the total quantity is given.
(b) When parts of a quantityare given to us as ratios, we have seen how to convert
them to percentages.
(c) The increase or decrease in a certainquantity can also be expressed as percentage.
(d) The profit or loss incurredin a certain transaction can be expressedin terms of percentages.
(e) While computing intereston an amount borrowed, the rate of interest is given in terms of per cents. For example, ` 800 borrowed for 3 years at 12% per annum. Simple Interest:Principal means the borrowed money.
The extra money paid by borrower for using borrowedmoney for given time is called interest(I).
The period for which the money is borrowed is called ‘TimePeriod’ (T).
Rate of interestis generally given in percentper year.
Interest, I = PTR/100
Total money paid by the borrower to the lenderis called the amount.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

Integers | Study
Pre-Requisires
Test & Enrich
English Version Integers | Speed Notes
Notes For Quick Recap
Integers are a bigger collection of numbers which is formed by whole numbers and their negatives. You have studied inthe earlier class, about the representation of integers onthe number lineand their addition and subtraction. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
We now study theproperties satisfied by addition andsubtraction.
(a) Integers are closed for addition and subtraction both. That is, a + b and a – b are again integers, where a andb are anyintegers.
(b) Addition is commutative forintegers, i.e., a + b = b + a for allintegers a andb.
(c) Addition is associative for integers, i.e., (a + b) + c = a + (b + c) for all integers a, b and c.
(d) Integer 0 is the identity under addition. That is, a + 0 = 0 + a = a for every integer a. We studied, how integers could be multiplied, andfound that product of a positive and a negative integer is a negative integer, whereas the product of two negative integers isa positive integer. For example, –2 × 7 = –14 and –3 × – 8 =24.
Product of even number of negative integers is positive, whereas the product of odd number of negative integers is negative. Integers showsome properties under multiplication.
(a) Integers are closed under multiplication. Thatis, a × b isan integer forany two integers a and b.
(b) Multiplication is commutative for integers. Thatis, a × b = b × a forany integers a and b.
(c) The integer 1 is theidentity under multiplication, i.e., 1 × a = a × 1 = a forany integer a.
(d) Multiplication is associative for integers, i.e.,(a × b) × c = a × (b × c) for anythree integers a,b and c.
Under addition and multiplication, integers show a property called distributive property.
That is, a× (b +c) = a × b+ a × c forany three integers a, b andc.
The properties of commutativity, associativity under addition and multiplication, and the distributive property help us to make our calculations easier. We alsolearn how to divide integers. We found that,
(a) When a positive integer is divided by a negative integer, the quotient obtained is a negative integer and vice-versa. (b) Division of a negative integer by another negative integer gives a positive integer as quotient. For any integer a,we have
1) The numbers. . . , —4,—3, —1, 0, 1, 2,3, 4, etc.are integers.
2) 1, 2, 3, 4, 5. . . . are positive integers and —1,-2, —3,.. are negative integers.
3) 0 isan integer which is neither positive nornegative.
4). On an integer number line, all numbers to the right of 0 arepositive integers andall numbers tothe left of0 are negative integers.
5) 0 is less than everypositive integer and greater than everynegative integer.
6) Every positive integer is greater than every negative integer.
7) Two integers thatare at thesame distance from 0, but onopposite sides of it are called opposite numbers.
8. The greater the number, the lesser is its opposite.
9. The sumof an integer and its opposite is zero.
10. The absolute valueof an integer is the numerical value of theinteger without regard to its sign.
The absolute value of an integer a isdenoted by |a| and is given by a,if a is positive or 0 a = -a,if a is negative
11. The sum oftwo integers of the same sign is an integer of the same sign whose absolute value is equal to the sum of the absolute values of the given integers.
12. The sum of two integers of opposite signs is an integer whose absolute value is the difference of the absolute values of addend and whose sign isthe sign ofthe addend having greater absolute value.
13. To subtract an integer b from another integer a, we change the sign ofb and addit to a. Thus, a − b = a + (−b)
14. All properties of operations onwhole numbers aresatisfied by theseoperations on integers.
15. If aand b are two integers, then(a − b) is alsoan integer.
16. −a and aare negative oradditive inverses of each other.
17. To find theproduct of twointegers, we multiply theirabsolute values andgive the result a plus signif both thenumbers have the same sign or a minussign otherwise.
18. To find thequotient of oneinteger divided by another non-zero integer, we divide their absolute values and give the result a plus sign if both the numbers have the same sign or a minus signotherwise.
19. All the properties applicable to wholenumbers are applicable to integers in addition, the subtraction operation has the closure property.
20. Any integer whenmultiplied or divided by 1 gives itself and whenmultiplied or divided by-1 gives its opposite.
21. When expression hasdifferent types ofoperations, some operations haveto be performed before the others. That is, each operation has its own precedence. The order in which operations are performed is division, multiplication, addition and finally subtraction (DMAS).
22. Brackets are usedin an expression when we wanta set of operations to be performed before the others.
23. While simplifying anexpression containing brackets, the operations within the innermost set of brackets are performed first and then those brackets are removed followed by the ones immediately after them tillall the brackets are removed.
24. While simplifying arithmetic expressions involving various brackets and operations, we use BODMAS rule.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

Fractions and Decimals | Study
Pre-Requisires
Test & Enrich
English Version Speed Notes
Notes For Quick Recap

(Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
Fractions:

4. A fraction whose numerator is less than the denominator is called a proper fraction.
5. A fraction whose numerator is more than or equal to the denominator is called animproper fraction.
6. A combination of a whole number and a proper fraction is called a mixed fraction.
7. To get a fractionequivalent to a given fraction,we multiply (or divide) its numerator and denominator by the same non-zero number.
8. Fractions having the same denominators are called like fractions. Otherwise, they are calledunlike fractions.
9. A fraction is said to be in its lowest termsif its numerator and denominator have no commonfactor other than 1.
10. To compare fractions, we use the followingsteps:
Step I Find the LCMof the denominators of the given fractions.
Step II Converteach fraction to itsequivalent fraction with denominator equal to the LCM obtained in step I.
Step Ill Arrangethe fractions in ascending or descending order byarranging numerators in ascending or descending order.
11. To convert unlike fractions into like fractions, we use the following steps:Step I Find the LCM of the denominators of the given fractions.
Step II Convert each of the given fractions into an equivalent fraction having denominator equal to the LCM obtained in step I.
12. To add (or subtract)fractions, we may use the following steps:Step I Obtain the fractionsand their denominators.
Step II Find the LCMof the denominators.
Step III Convert each fraction into an equivalent fraction having its denominator equal to the LCM obtainedin step II.
Step IV Add (or subtract) like fractions obtained in Step Ill.
Step III Convert each fraction into an equivalent fraction having its denominator equal to the LCM obtainedin step II.
Step IV Add (or subtract) like fractions obtained in Step Ill.

14. Two fractions are said to be reciprocal of each other, if their product is 1. The reciprocal of a non zero fraction a/b is b/a.
15. The divisionof a fraction a/b by a non-zero fraction c/d is the product of a/b with the
reciprocal of c/d.
Decimals:
1. Decimals are an extension of our number system.
2. Decimals are fractionswhose denominators are 10, 100, 1000 etc.
3. A decimal has two parts, namely, the whole numberpart and decimal part.
4. The number of digits containedin the decimal part of a decimal number is known as the numberof decimal places.
5. Decimals having the same number of places are called like decimals, otherwise they are knownas unlike decimals.
6. We have, 0.1 = 0.10 = 0.100 etc, 0.5 = 0.50 = 0.500 etc and so on. That is by annexing zeros on the right side of the extreme right digit of the decimalpart of a number does not alterthe value of the number.
7. Unlike decimals may be converted into like decimals by annexing the requisite numberof zeros on the right side of the extreme right digit in the decimal part.
8. Decimal numbers may be convertedby using the following steps.Step I Obtain the decimalnumbers
Step II Compare the whole partsof the numbers. The number with greater whole part will be greater. If the whole parts are equal, go to next step.
Step Ill Compare the extreme left digits of the decimal parts of two numbers. The number with greater extreme left digit will be greater. If the extreme left digits of decimal parts are equal,then compare the next digits and so on.
9. A decimal can be converted into a fractionby using the following steps:Step I: Obtain the decimal.
Step II: Take the numerator as the number obtained by removing the decimal point from the given decimal.
Step III: Take the denominator as the number obtainedby inserting as many zeros with 1 (e.g.10, 100 or 1000 etc.)as there are number of places in the decimal part.
10. Fractions can be converted into decimals by using the following steps:
Step I: Obtain the fractionand convert it into an equivalent fraction with denominator 10 or 100 or 1000 if it is not so.
Step II: Write its numeratorand mark decimal point after one place or two places or threeplaces from right towards left if the denominator is 10 or 100 or 1000 respectively. If the numerator is short of digits, insert zeros at the left of the numerator.
11. Decimals can be added or subtracted by using the following steps:Step I: Convert the given decimals to like decimals.
Step II: Write the decimals in columns with their decimal pointsdirectly below each other so that tenthscome under tenths, hundredths come and hundredths and so on.
Step III: Addor subtract as we add or subtract whole numbers.
Step IV: Place the decimal point, in the answer, directly below the other decimal points.
12. In order to multiply a decimal by 10, 100, 1000 etc., we use the following rules:
Rule I: On multiplying a decimal by 10, the decimalpoint is shiftedto the right by one place.
Rule II: On multiplying a decimal by 100, the decimal point is shiftedto the right by two places.
Rule III: On multiplying a decimal by 1000, the decimal point is shiftedto the right by threeplaces, and so on.
13. A decimal can be multiplied by a whole number by using following steps:
Step I: Multiply the decimal without the decimalpoint by the given whole number.
Step II: Mark the decimal point in the product to have as many placesof decimal as are there in the given decimal.
14. To multiply a decimal by another decimal, we follow following steps:
Step I: Multiply the two decimalswithout decimal point just like whole numbers.
Step II: Insert the decimal point in the product by countingas many places from the right to left as the sum of the number of decimalplaces of the given decimals.
15. A decimal can be dividedby 10, 100, 1000 etc by using the followingrules:
Rule I When a decimal is divided by 10, the decimal point is shifted to the left by one place.
Rule II When a decimal is divided by 100, the decimal point is shifted to the left by two places.
Rule III When a decimal is divided by 1000, the decimal point is shiftedto the left by threeplaces.
16. A decimal can be divided by a whole number by using the following steps:Step I: Check the whole number part of the dividend.
Step II: If the wholenumber part of the dividend is less than the divisor,then place a 0 in the onesplace in the quotient. Otherwise, go to step Ill.
Step III: Divide the whole number part of the dividend.
Step IV: Place the decimal point to the right of ones place in the quotient obtained in step I.
Step V: Divide the decimal part of the dividend by the divisor. If the digits of the dividend are exhausted, then place zeros to the right of dividendand remainder each time and continue the process.
17. A decimal can be divided by a decimal by using the following steps:
Step 1 Multiple the dividend and divisor by 10 or 100 or 1000 etc. to convert the divisor into a whole number.
Step II Divide the new dividendby the whole number obtainedin step I.
Extra:


Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments

Data Handling | Study
Pre-Requisires
Test & Enrich
English Version Data Handling | Speed Notes
Notes For Quick Recap
The collection, recording and presentation of data help us organiseour experiences and draw inferences from them.
Before collecting data we need to know what we would use it for.
The data that is collected needs to be organised in a propertable, so that it becomeseasy to understand and interpret. (Scroll down to continue …).
Study Tools
Audio, Visual & Digital Content
Average is a numberthat represents or shows the central tendencyof a group of observations or data.
Arithmetic mean is one of the representative values of data.
Mean = sum of all observations/ Number of observations.
Mode is another form of central tendency or representative value.
The mode of a set of observations is the observation that occurs most often.
If each of the value in a data is occurring one time, then all are mode.
Sometimes we also say that this data has no mode since none of them is occurring frequently.
Median is also a form of representative value.
It refers to the value which lies in the middle of the data with half of the observations above it and the other half below it.

.
A bar graph is a representation of numbers using bars of uniform widths.
Double bar graphshelp to comparetwo collections of data at a glance.
Double bar graphshelp to comparetwo collections of data at a glance.
There are situations in our life, that are certain to happen, some that are impossible and some that may or may not happen.
The situation that may or may not happen has a chanceof happening.
Probability: A branch of mathematics that is capable of calculating the chance or likelihood of an event taking place (in percentage terms).
If you have 10 likelihoods and you want to calculate the probability of 1 event taking place,it is said that its probability is 1/10 or event has a 10% probability of taking place.
Events that have many possibilities can have probability between 0 and 1.
Important Formulae – Data Handling
1. A trial is anaction which results in one or several outcomes. 2. An experiment in whichthe result ofa trial cannot be predicted inadvance is called a random experiment.
3. An event associated to a random experiment is thecollection of someoutcomes of theexperiment.
4. An event associated witha random experiment is said tohappen if anyone of theoutcomes satisfying thedefinition of theevent is anoutcome of theexperiment when it is performed.
5. The Empirical probability ofhappening of an event E is defined as: P(E)= Number of trials in which the event happened/ Total number of trials.
Hindi Version Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments