Tag: Class 9

Pre-Requisires

Test & Enrich

Basics Of Geometry

Triangles

English Version

Quadrilaterals | Speed Notes

Notes For Quick Recap

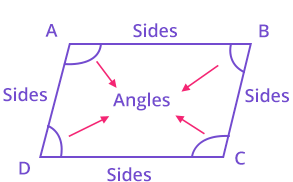
Quadrilateral

Any closed polygon with four sides, four angles and four vertices are called Quadrilateral. It could be regular or irregular. (Sroll down to continute …)

Study Tools

Audio, Visual & Digital Content

Revision Notes – CBSE 09 Math – Quadrilaterals

Quadrilaterals

A simple closed figure bounded by four line segments is called a quadrilateral, it has four sides i.e., AB, BC, CD and AD and four vertices as A, B, Can d D and the sum of all angles of a quadrilateral is 360.

Characteristics of a quadrilateral

Angle Sum Property of a Quadrilateral:

Quadrilateral is a four sided closed figure.

Sum of all angles of a quadrilateral is 360°.

Parts Of Quadrilaterals

Types Of Quadrilaterals

Classification of quadrilaterals

Quadrilaterals are broadly classified into three categories as:

(i) Kite

(ii) Trapezium

(ii) Parallelogram

Kite:

A kite is a quadrilateral with two distinct pairs of adjacent sides that are equal in length. It resembles a flying kite in shape.

🪁 Types of Kites:

There are several types of kites based on their properties:

Square Kites:

All four sides are equal in length, making it both a kite and a square.

Rhombus Kites:

All four sides are equal in length, and opposite angles are equal, making it both a kite and a rhombus.

Right Kites:

One pair of opposite angles is a right angle (90 degrees).

Equilateral Kites:

Two pairs of adjacent sides are equal, and the diagonals are perpendicular bisectors of each other.

(i) Kite has no parallel sides

(ii) Kite has a pair of equal adjacent sides.

(ii) It is not a parallelogram

Characteristics Of Kite:

Perimeter Of Kite

Area Of Kite

Trapezium:

Trapezium is a quadrilateral with the following characteristics:

(i) One pair of opposite sides is parallel to each other.

(ii) The other pair of opposite sides may not be parallel to each other.

Characteristics Of Trapezium

(i) Sum of all angles of a quadrilateral is 360°.

(ii) One pair of opposite sides is parallel to each other.

(iii) The other pair of opposite sides need not be parallel to each other.

Types Of Trapezium:

Quadrilaterals are broadly classified into two categories as:

(i) Isosceles Trapezium.

(ii) Scalene Trapezium.

(i) Right Trapezium.

Isosceles Trapezium:

Isosceles Trapezium is a quadrilateral with the following characteristics:

(i) One pair of opposite sides is parallel to each other.

(ii) The other pair of opposite sides are equal.

(iii) The other pair of opposite sides need not be parallel to each other.

Isosceles Trapezium is a trapezium with the following characteristics:

(i) One pair of opposite sides is parallel to each other.

(ii) The other pair of opposite sides are equal.

(iii) The other pair of opposite sides need not be parallel to each other.

Characteristics Of Isosceles Trapezium

(i) Sum of all angles of a quadrilateral is 360°.

(ii) One pair of opposite sides is parallel to each other.

(iii) The other pair of opposite sides are equal.

(iv) The other pair of opposite sides need not be parallel to each other.

Scalene Trapezium:

Scalene trapezium: Classified by the length of the legs or the measurement of their angles.

Characteristics Of Scalene Trapezium

Right Trapezium:

Right trapezium: Has one pair of parallel sides and one pair of right angles.

Characteristics Of Right Trapezium

Perimeter Of Trapezium

Area Of Trapezium

Parallelogram:

A parallelogram is a quadrilateral (a four-sided polygon) in which both pairs of opposite sides are parallel and equal in length.

In other words, Parallelogram is a quadrilateral with the following characteristics:

Characteristics of a parallelogram

Parallelogram is a quadrilateral with the following characteristics:

(i) Two pairs of opposite sides are parallel to each other.

(ii) Two pairs of opposite sides are equal in length.

(iii) Sum of all angles of a Parallelogram is 360°.

(iv) Two pairs of opposite sides are parallel to each other.

(v) Two pairs of opposite sides are equal in length.

(vi) Two pairs of opposite angles are equal.

(vii) Diagonals bisect each other.

(viii) Diagonals need not be equal to each other.

(ix) Diagonals divide it into two congruent triangles.

Types Of Parallelogram

Parallelograms are broadly classified into three categories as:

(i) Rectangle (ii) Rhombus (iii) Square

Perimeter Of Parallelogram:

Perimeter of a Parallelogram is the length of the boundary of the Parallelogram.

Perimeter of a Parallelogram = 2(length + width)

Area Of Parallelogram

Measure of the space enclosed by the boundary of a Parallelogram is called its area.

Area of a Parallelogram = Base x Height

Rectangle:

A rectangle is a Parallelogram with four right angles.

In other words, A rectangle is a quadrilateral (a four-sided polygon) in which both pairs of opposite sides are parallel and equal in length.and has four right angles.

Characteristics Of Rectangle

Rectangle is a quadrilateral with the following characteristics:

(i) Two pairs of opposite sides are parallel to each other.

(ii) Two pairs of opposite sides are equal in length.

(iii) All four angles are right angles. (each angle is 90 ^o).Sum of all angles of a quadrilateral is 360°.

(iv) Two pairs of opposite sides are parallel to each other.

(v) Two pairs of opposite sides are equal in length.

(vi) All four angles are right angles. (each angle is 90 ^o).

(vii) Diagonals bisect each other.

(viii) Diagonals are equal to each other.

(ix) Diagonals of a rectangle divide it into two congruent triangles.

Conclusions:

Every Rectangle is a Parallelogram. But Every Parallelogram need not be a Rectangle.

Condition for a rhombus to be a square:

If all four angles of a parallelogram are right angles. (each angle is 90 ^o), the parallelogram becomes a Rectangle.

Perimeter Of Rectangle

Perimeter of a rectangle is the length of the boundary of the rectangle.

Perimeter of a rectangle = 2(length + width)

Area Of Rectangle

Measure of the space enclosed by the boundary of a rectangle is called its area.

Area of a rectangle = length x width

Rhombus:

Rhombus is a Parallelogram with four equal sides.

In other words, A rectangle is a quadrilateral (a four-sided polygon) in which both pairs of opposite sides are parallel and equal in length.and also has four equal sides.

Characteristics Of Rhombus

Rhombus is a quadrilateral with the following characteristics:

(i) Two pairs of opposite sides are parallel to each other.

(ii) All four sides are equal in length.

(iii) Sum of all angles of a quadrilateral is 360°.

(iv) Two pairs of opposite sides are parallel to each other.

(v) All four sides are equal in length.

(vi) Two pairs of opposite angles are equal.

(vii) Diagonals bisect each other.

(viii) Diagonals need not be equal to each other.

(ix) Diagonals divide a Rhombus into two congruent triangles.

Conclusions:

Every Rhombus is a Parallelogram. But Every Parallelogram need not be a Rhombus.

Condition for a rhombus to be a square:

If all four angles of the Rhombus are right angles, the Rhombus becomes a square.

If all the sides of a parallelogram are equal, the parallelogram becomes a Rhombus.

Perimeter Of Rhombus

Perimeter of a Rhombus is the length of the boundary of the Rhombus.

Perimeter of Rhombus = 2(length + width)

Area Of Rhombus

Measure of the space enclosed by the boundary of a rhombus is called its area.

Area of Rhombus = ½(Diagonal 1 + Diagonal 2 x height)

Area of Rhombus = Base x Height

Square:

Characteristics Of Square

Square is a quadrilateral with the following characteristics:

(i) Two pairs of opposite sides are parallel to each other.

(ii) All four sides are equal in length.

(iii) All four angles are right angles. (each angle is 90 ^o).

(iv) Sum of all angles of a quadrilateral is 360°.

(v) Diagonals bisect each other.

(vi) Diagonals need not be equal to each other.

(vii) Diagonals divide a Rhombus into two congruent triangles.

Conclusions:

Every square is a Rhombus. But Every Rhombus need not be a square.

Condition for a rhombus to be a square:

If all the angles of a rhombus are right angles (equal to 90^o), the rhombus becomes a square.

Condition for a parallelogram to be a square:

(i) If all the angles of a parallelogram are right angles (equal to 90^o), and all the sides of a parallelogram are equal in length, the parallelogram becomes a square.

Note: Every Square is a rectangle. But Every Rectangle need not be a square.

Condition for a Rectangle to be a square:

If all the sides of a Rectangle are equal in length, the Rectangle becomes a square.

Perimeter Of Square

Perimeter of a Square is the length of the boundary of the square.

Perimeter of square = 4(side)

Area Of Square

Measure of the space enclosed by the boundary of a Square is called its area.

Area of square = side²

Practical Geometry Of Quadrilaterals:

Angle Sum Property of a Quadrilateral

The sum of the four angles of a quadrilateral is 360°

If we draw a diagonal in the quadrilateral, it divides it into two triangles.

And we know the angle sum property of a triangle i.e. the sum of all the three angles of a triangle is 180°.

The sum of angles of ∆ADC = 180°.

The sum of angles of ∆ABC = 180°.

By adding both we get ∠A + ∠B + ∠C + ∠D = 360°

Hence, the sum of the four angles of a quadrilateral is 360°.

Example

Find ∠A and ∠D, if BC∥ AD and ∠B = 52° and ∠C = 60° in the quadrilateral ABCD.

Solution:

Given BC ∥ AD, so ∠A and ∠B are consecutive interior angles.

So ∠A + ∠B = 180° (Sum of consecutive interior angles is 180°).

∠B = 52°

∠A = 180°- 52° = 128°

∠A + ∠B + ∠C + ∠D = 360° (Sum of the four angles of a quadrilateral is 360°).

∠C = 60°

128° + 52° + 60° + ∠D = 360°

∠D = 120°

∴ ∠A = 128° and ∠D = 120 °.

Types of Quadrilaterals

Remark: A square, Rectangle and Rhombus are also a parallelogram.

Properties of a Parallelogram

Theorem 1: When we divide a parallelogram into two parts diagonally then it divides it into two congruent triangles.

∆ABD ≅ ∆CDB

Theorem 2: In a parallelogram, opposite sides will always be equal.

Theorem 3: A quadrilateral will be a parallelogram if each pair of its opposite sides will be equal.

Here, AD = BC and AB = DC

Then ABCD is a parallelogram.

Theorem 4: In a parallelogram, opposite angles are equal.

In ABCD, ∠A = ∠C and ∠B = ∠D

Theorem 5: In a quadrilateral, if each pair of opposite angles is equal, then it is said to be a parallelogram. This is the reverse of Theorem 4.

Theorem 6: The diagonals of a parallelogram bisect each other.

Here, AC and BD are the diagonals of the parallelogram ABCD.

So the bisect each other at the centre.

DE = EB and AE = EC

Theorem 7: When the diagonals of the given quadrilateral bisect each other, then it is a parallelogram.

This is the reverse of the theorem 6.

The Mid-point Theorem

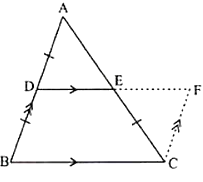
1. If a line segment joins the midpoints of the two sides of the triangle then it will be parallel to the third side of the triangle.

If AB = BC and CD = DE then BD ∥ AE.

2. If a line starts from the midpoint of one line and that line is parallel to the third line then it will intersect the midpoint of the third line.

If D is the midpoint of AB and DE∥ BC then E is the midpoint of AC.

Example

Prove that C is the midpoint of BF if ABFE is a trapezium and AB ∥ EF.D is the midpoint of AE and EF∥ DC.

Solution:

Let BE cut DC at a point G.

Now in ∆AEB, D is the midpoint of AE and DG ∥ AB.

By midpoint theorem, G is the midpoint of EB.

Again in ∆BEF, G is the midpoint of BE and GC∥ EF.

So, by midpoint theorem C is the midpoint of BF.

Hence proved.

Hindi Version

Dig Deep

Topic Level Resources

Sub – Topics

Select A Topic

Topic:

Chapters Index

Select Another Chapter

Assessments

Personalised Assessments

November 17, 2023

FORCE AND LAWS OF MOTION | Study
Assess
Interact
Pre-Requisires
Test & Enrich
Pre – Requisite

English Version
Speed Notes
Notes For Quick Recap
Force : Push or pull is called Force.
Example:
We push or pull to open a door. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
Effects of Force
Force can change the shape and size of an object.
Force can move a stationary object.
Force can change the speed of a body.
Force can stop a moving body.
Force can change the direction of a moving object.
Net or Resultant Force:
Resultant Force or Net Force acts on a body if two or more forces act on it at the same time. Resultant Force or Net Force on a body is defined as the net effective force due to the multiple forces acting on it simultaneously.
Based on Net force, Forces are classified into two types as:
(A) Balanced forces
(B) Unbalanced forces
(A) Balanced Forces
• If the resultant of applied forces is equal to zero, the forces are called balanced forces.
• Balanced forces do not cause any change in state of an object.
• Balanced forces can change the shape and size of an object.
For example, when forces are applied from both sides over a balloon, the size and shape of the balloon is changed.
(B) Unbalanced Forces
• If the resultant of applied forces are greater than zero, the forces are called unbalanced forces.
• Unbalanced forces can do the following :
* Move a stationary object
* Increase the speed of a moving object
* Decrease the speed of a moving object
* Stop a moving object
* Change the shape and size of an object
Laws of Motion :
Galileo Galilei :
Galileo Galilei was the first to say that objects move with a constant speed when no forces act on them.
That is, if there is no unbalanced force acting on the object, the object moves forever with a constant speed without changing its direction.
In other words, if an object is moving on a frictionless path and no other force is acting upon it, the object moves forever with a constant speed without changing its direction.
Galileo’s Experiment:
Galileo’s thought experiment considered rolling balls on inclined planes in the absence of friction or other resistant forces.
Galileo arranged two inclined planes opposite to each other as shown.
He rolls down the ball from the first inclined plane to climb the second inclined plane.
Galileo observations:
Galileo observed that:
The ball rolling down the first inclined plane comes to rest after climbing a certain height on the second inclined plane.
The speed acquired by the ball moving down a plane from a height is sufficient to enable it to reach the same height when climbing up another plane at a different inclination .
As the angle decreases, the body should travel a greater distance.
From these observations, Galileo hypothesized as:
if the force acting on the ball is only gravitational force, the height reached by the ball must be equal to the height from which it was rolled.
When the inclinations of the two planes are the same, the distance travelled by the sphere while rolling down is equal to the distance travelled by it while climbing up.
Now, if the inclination of the second plane is decreased slowly, then the sphere needs to travel over longer distances to reach the same height.
If the second plane is made horizontal, then the sphere must travel forever trying to reach the required height.
This is the case when there is no unbalanced force acting on it.
From his experiments Galileo proposed that the body could travel indefinitely far as , contrary to the Aristotelian notion of the natural tendency of an object to remain at rest unless acted upon by an external force.
Therefore, Galileo can be credited with introducing the concept of inertia, later exploited by Newton.
However, in reality, frictional forces bring the sphere to rest after it travels over a finite distance.
After further study, Newton, in his first law of motion, stated that all objects resist a change in their natural state of motion.
This tendency of resisting any change in the natural state of motion is called “inertia”.
Newton’s Laws of Motion:
Newton studied the ideas of Galileo and gave the three laws of motion. These laws are popular as Newton’s laws of motion.
Newton’s First Law of Motion (Law of Inertia):
Any object remains in the state of rest or in the state of uniform motion along a straight line, until it is compelled to change its state by applying an external force.
Newton’s First Law of Motion in Everyday Life:
(a) A person standing in a bus falls backward when the bus starts suddenly.
This happens because the person and bus both are at rest while the bus is not moving, but as the bus starts moving, an external force is acted by the bus on the legs of the person. This external force moves legs along with the bus. But the rest of his body has the tendency to remain in rest known as inertia of rest. Because of this, the person falls backward; if he is not alert.
(b) A person standing in a moving bus falls forward if the driver applies brakes suddenly. This happens because when the bus is moving, the person standing in it is also in motion along with the bus. But when the driver applies brakes the speed of the bus decreases suddenly or the bus comes to a state of rest suddenly, in this condition the legs of the person which are in contact with the bus come to rest while the rest of his body have the tendency to remain in motion. Because this person falls forward if he is not alert.
(c) Before hanging the wet clothes over the laundry line, usually many jerks are given to the clothes to get them dried quickly. Because of jerks, droplets of water from the pores of the cloth fall on the ground and the reduced amount of water
in clothes dries them quickly. This happens because when suddenly clothes are made in motion by giving jerks, the water droplets in it have the tendency to remain in rest and they are separated from clothes and fall on the ground.
(d) When the pile of coins on the carrom-board is hit by a striker, the coin only at the bottom moves away leaving the rest of the pile of coins at the same place. This happens because when the pile is struck with a striker, the coin at
the bottom comes in motion while rest of the coin in the pile has the tendency to remain in the rest and they vertically falls the carrom-board
and remain at the same place.
Momentum
Momentum of an object at state of rest is zero :
Let an object with mass ‘m’ be at rest.
Since, object is at rest, its velocity, v = 0
We know that
Momentum, p is equal to the product of mass, m and velocity, v = 0
⇒ p = m × 0 = 0
Thus, the momentum of an object in the rest i.e., non-moving, is equal to zero.
Unit of momentum :
SI unit of mass = kg
SI unit of velocity = meter per second i.e., m/s
We know that Momentum (p) = m × v
⇒ p = kg × m/s
Or ⇒ p = kg m/s
Therefore, SI unit of momentum = kg m/s
Impulse and Impulsive Force
If a cricketer catches a ball he moves his hand back while catching the ball. He does this to reduce the impact, due to the force of the ball on his hand. An object in motion has momentum. Momentum is defined as the product of mass and velocity of an object.
The momentum of the object at the starting of the time interval is called the initial momentum and the momentum of the object at the end of the time interval is called the final momentum. The rate of change of momentum of an object is directly proportional to the applied force.
Newton’s second law quantifies the force on an object. The magnitude of force is given by the equation,
F = ma, where ‘m’ is the mass of the object and ‘a’ is its acceleration. The CGS unit of force is dyne and the SI unit is newton (N).
A large amount of force acting on an object for a short interval of time is called impulse or impulsive force. Numerically impulse is the product of force and time. Impulse of an object is equal to the change in momentum of the object.
Impulse and Impulsive Force
The momentum of an object is the product of its mass and velocity. The force acting on a body causes a change in its momentum. In fact, according to Newton’s second law of motion, the rate of change in the momentum of a body is equal to the net external force acting on it.
Another useful quantity that we come across is “impulse”. “Impulse” is the product of the net external force acting on a body and the time for which the force is acted.
If a force “F” acts on a body for “t” seconds, then Impulse I = Ft.
In fact, this is also equal to the change in the momentum of the body. It means that due to the application of force, if the momentum of a body changes from “P” to “P ‘ ”, then impulse,I = P ‘ – P.
For the same change in momentum, a small force can be made to act for a long period of time, or a large force can be made to act for a short period of time. A fielder in a cricket match uses the first method while catching the ball. He pulls his hand down along with the ball to decrease the impact of the ball on his hands.
In a cricket match, when a batsman hits a ball for a six, he applies a large force on the ball for a very short duration. Such large forces acting for a short time and producing a definite change in momentum are called “impulsive forces”.
Newton’s Second Law of Motion
Newton’s Second Law of Motion states that, the rate of change in momentum of an object is proportional to applied unbalanced force in the direction of force.
Mathematical expression:
State and derive newton’s second law of Motion
Statement: Newton’s second law of motion states that the rate of change of momentum of an object is Proportional to the applied unbalanced force in the direction of force.
Derivation of Newton’s second law of motion:
Suppose an object of mass, m is moving along a straight line with an initial velocity, u.
It is uniformly accelerated to velocity, v in time, t by the application of a constant force, F throughout the time t.
⇒Initial momentum of the object, p₁= mu
⇒Final momentum, p₂ = mv
⇒Change in momentum = p₂ – p₁
⇒The change in momentum = mv – mu
⇒The change in momentum = m × (v – u)
⇒The rate of change of momentum = m(v -u)t
⇒ m (v -u)t
According to Newton’s Second Law of Motion,
Applied force α Rate of change in motion
⇒ F m (v -u)t
F=km (v -u)t = kma —————————- (i)
Here, k is a constant of proportionality and
(v -u)t is the rate of change of velocity, which equals acceleration, a.
The SI units of mass and acceleration are kg and m s-²respectively.
The unit of force is so chosen that the value of the constant, K becomes one For this.
One unit of force is defined as the amount that produces acceleration
of 1 m s^-2 in an object of 1 kg mass.
That is,
1 unit of force = k × (1 kg) × (1 m s^-2).
Thus, the value of k becomes 1. From Eq. (iii)
F = ma ————————————-
The unit of force is kg m s^-2 or newton, with the symbol N.
Newton’s Third Law of Motion
To every action there is an equal and opposite reaction.
Applications:
(i) Walking is enabled by 3rd law.
(ii) A boat moves back when we deboard it.
(iii) A gun recoils.
Rowing of a boat.
Law of Conservation of Momentum
Law of conservation of momentum states that, if two or more bodies collide, the sum of the initial momentum is equal to the sum of the final momentum.
Or
Law of conservation of momentum states that the sum (total) of the individual momentums of the colliding bodies just before the collision is equal to the sum (total) of the individual momentums of the colliding bodies after the collision
Derivation of Law of Conservation of Momentum From Newton’s Third Law of Motion.
Answer:
For a system of bodies ( two or more bodies ), the total vector sum of momenta of all the bodies due to the mutual action and reaction remain unchanged as long as no external force is acted on the system.
Consider two bodies A and B of the masses m₁, m₂moving with the initial velocities u₁, u₂respectively.
For a system, let, these masses collide and their velocities after collision are v₁, v₂respectively.
If ‘A’ applies a F on B for a time, t;
‘B’ applies a force –F on A for time t [according to Newton’s third law of motion].
Then,
\[\]
Therefore, the sum of momentum before impact is equal to the sum of the momenta after the impact represents the law of conservation of momentum.
Hindi Version
Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments
Assess
November 5, 2023
GRAVITATION | Study
Assess
Interact
Pre-Requisires
Test & Enrich
Pre – Requisite

English Version
Speed Notes
Notes For Quick Recap
Study Tools
Audio, Visual & Digital Content

Hindi Version
Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments
Assess
November 5, 2023
WORK AND ENERGY | Study
Assess
Interact
Pre-Requisires
Test & Enrich
Pre – Requisite

English Version
Speed Notes
Notes For Quick Recap
Study Tools
Audio, Visual & Digital Content

Hindi Version
Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments
Assess
November 5, 2023
SOUND | Study
Assess
Interact
Pre-Requisires
Test & Enrich
Pre – Requisite

English Version
Speed Notes
Notes For Quick Recap
Study Tools
Audio, Visual & Digital Content

Hindi Version
Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments
Assess
November 5, 2023
IMPROVEMENT IN FOOD RESOURCES | Study
Assess
Interact
Pre-Requisires
Test & Enrich
Pre – Requisite

English Version
Speed Notes
Notes For Quick Recap
Study Tools
Audio, Visual & Digital Content

Hindi Version
Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments
Assess
November 5, 2023

MOTION | Study

Pre-Requisires

Test & Enrich

Rest And Motion | Speed Notes

Notes For Quick Recap

Rest And Motion

Motion: An object is said to be in motion when its position changes with respect to the reference point with time.

Rest: An object is said to be at rest when its position does not change with respect to a reference point with time.

Reference Point:

A specific point with respect to which we describe the location of an object is called a reference point.

A body can be at rest as well as in motion at the same time with respect to two different reference points.

Therefore, Rest and Motion are relative terms not absolute terms.

Rest and Motion are relative tems. Since a body may be at rest relative to one object and simultaneously it may be in motion relative to another object.

Example: a passenger sitting in a moving vehicle is at rest with respect to his fellow passenger but he is in motion with respect to a place outside the bus.

(Scroll down to continue …)

Study Tools

Audio, Visual & Digital Content

Distance and Displacement

Distance: The total length of path covered by an object is said to be the distance travelled by it.
Displacement: Gap between the initial and final positions of an object is said to be its displacement. Or
The length of a line segment that joins the initial and final positions of an object is known as the displacement.

Difference Between Displacement and Displacement

Distance	Displacement
Distance is defined as the total length of the path travelled by an object to go from one point to another.	Displacement is defined as the length of the line segment that joins the initial and final positions of an object.
Since distance has only magnitude and its direction cannot be specified always, it is a scalar quantity.	Since displacement has magnitude and it is specified in a direction from initial position to final position, it is a vector quantity.
Distance can only have positive values.	Displacement can have both positive and negative values.
Distance depends on the length of the path travelled.	Displacement depends only on the initial and final point regardless of the path travelled.

Difference Between Displacement and Displacement

Speed And Velocity

Speed:

Speed or Average Speed: The distance travelled by an object in unit time is referred to as speed.
Its S.I unit is m/s.
In general speed refers to average speed.
For non-uniform motion, the average speed of an object is obtained by dividing the total distance travelled by an object with the total time taken.

Instantaneous Speed:
Speed of a body at an instant, that is at a very short span is known as Instantaneous Speed.
For a uniform motion, the average speed of an object is equal to its instantaneous speed throughout the path.

Velocity

Velocity Or Average Velocity:

In case of a uniform motion in a straight path, the average velocity is equal to its instantaneous velocity throughout its path.

Velocity (average velocity) of an object is equal to the instantaneous velocity of an object.

Differences Between Speed And Velocity

SPEED	VELOCITY
It is defined as the distance covered by a body per unit time. In other words, it is the rate of change of distance.	It is defined as the Net Displacement of a body per unit time In other words, it defined as the rate of change of net displacement.
It is a scalar quantity.	It is a vector quantity.
It can never be negative or zero.	It can be negative,zero or positive.
	Velocity is directed speed.
Speed may or may not be equal to velocity.	A body may possess different velocities at different positions, but the same speed.
For a moving body speed never decreases with time for a moving body.	For a moving body velocity can decrease or increase.
In case of a moving body, speed never become zero.	In case of a moving body, Velocity can be zero.
Speed in SI is measured in ms^-1	Velocity in SI, is measured in ms^-1

Differences Between Speed and Velocity

Uniform And Non-Uniform motion

Uniform motion or non accelerated motion: When an object covers equal distances in equal intervals of time, it is said to be in uniform motion. Uniform motion is a non-accelerated motion.
Non-uniform motion or accelerated motion: Motions where objects cover unequal distances in equal intervals of time. Uniform motion is an accelerated motion.

Acceleration

Acceleration: Change in the velocity of an object per unit time.

Graphical representation of motions

(i) Distance-time graph

For a distance-time graph, time is taken on x-axis and distance is taken on the y-axis.

[Note: All independent quantities are taken along the x-axis and dependent quantities are taken along the y-axis.]

(ii) Velocity-time graph

Equation of motion by graphical methods

Derivation Of Equations Of Motion

Equations of motion can be derived by two methods. They are (i) Graphical Method. (ii) Algebraic Method

Derivation of The Equations of Motion By Algebraic Method:

(a) Velocity-time relation:

Derivation of S = ut + ½ at²

(ii) The equation for position-time relation:

motion-cbse-notes-class-9-science-9

Derivation of v² – u² = 2as

(iii) Equation for position-velocity relation:

Conclusions From a Distance – Time Graph

Uniform Circular Motion

When a body moves in a circular path with uniform speed, its motion is called uniform circular motion.

Hindi Version

Dig Deep

Topic Level Resources

Sub – Topics

Select A Topic

Topic:

Chapters Index

Select Another Chapter

Assessments

Personalised Assessments

TISSUES | Study
Assess
Interact
Pre-Requisires
Test & Enrich
Pre – Requisite

English Version
Tissues | Speed Notes
Notes For Quick Recap
Are plants and Animals made of same types of tissues?
Plants are stationary, and hence are provided with some tissues made up of dead cells, which provide mechanical strength. They have to withstand unfavourable conditions like strong winds, storms, floods etc. Animals on other hand move around in search of food, mates, shelter. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
They consume more energy as compared to plants. Most of the tissues they contain are living.
Cell growth in animas is more uniform.
The structural organisation of organs and organ systems is far more specialized and localised in complex animals than even in very complex plants.
Plant tissues:
Meristematic Tissue: The growth of plants occurs only in certain specific regions. This is because the dividing tissue
also known as meristematic tissue is the region where they are present, meristematic tissues are classified as apical, lateral and intercalary. Apical meristem is present at the apical or growing tips of stems and roots. Apical meristem
increases the length of the plant. Lateral meristem is present in the radial portion of the stem or root. Lateral meristem increases the girth of the plant.
Intercalary meristem occurs at the base of the leaves or at the internodes. Intercalary meristem increases the length of the internode. Permanent Tissue Old meristematic cells lose the capacity to divide and transform into permanent tissues.
This process of taking up a permanent shape, size, and function is called differentiation. These are cells which have lost their capacity to divide but are specified to provide strength, flexibility and elasticity to the plant. These tissues can be further classified into simple permanent, complex permanent and special tissues. Simple permanent can be categorized into parenchyma, collenchyma and sclerenchyma based on their function. Parenchyma- they are live cells. They are usually loosely packed. This tissue provides support to plants and also stores food. In some situations it contains chlorophyll and performs photosynthesis and then it is called chlorenchyma. Parenchyma which contains large air cavities in aquatic plants is called aerenchyma. The aerenchyma helps in buoyancy. Collenchyma – These are elongated living cells with small intercellular spaces. Their cell walls
are made up of cellulose and pectin. Collenchyma occurs in the peripheral regions of stems and
leaves to provide mechanical support and flexibility in plants. Sclerenchyma – These are long, dead cells with a deposit of lignin in their cell wall. They have no intercellular spaces. Sclerenchyma occurs around the vascular tissues in stems, in the veins of leaves, and in the hard covering of seeds and nuts. They provide strength to the plant.
Epidermis aids in protection against loss of water, mechanical injury and invasion by parasitic
fungi. Since it has a protective role to play, cells of epidermal tissue form a continuous layer
without intercellular spaces. Epidermis of the leaf contains small pores called stomata. These are
necessary for gases exchange and transpiration. Cork – This is the outer protective tissue which replaces the epidermal cells in older roots and stems. Cork cells are dead and lack intercellular spaces. Their cell walls are thickened by suberin
which makes them impermeable to water and gas molecules.
Complex permanent tissue:
Complex permanent tissue comprises of conducting tissues called xylem and phloem. Xylem is useful in transport of water and soluble substances. Xylem consists of tracheids, vessels, fibres and xylem parenchyma. Transport of minerals and water is unidirectional in xylem. Phloem is useful in transport of food molecules. Phloem comprises of sieve tubes, sieve cells, companion cells, phloem fibres and phloem parenchyma. Phloem is unlike xylem in that materials can move in both directions in it.
Animal Tissues:
These are the tissues present only in animals. Different types of animal tissues are epithelial tissue, connective tissue, muscle tissue and nervous tissue.
Epithelial Tissue:
Epithelial tissue forms a lining all over the body of the organism. It protects the inner lying
parts.
It is also secretory in function to secrete sebum and excrete wastes along with sweat.
Sometimes it is absorptive in nature. Epithelial tissues act like a barrier to keep the different body systems separate. These are tightly packed and form a continuous sheet without intercellular spaces.
Squamous epithelium has flat and thin cells with no intercellular spaces.
Squamous epithelium provides is found in the outer layer of the skin, lining the cavities of blood vessels, lung alveoli, lining of oesophagus and the lining of the mouth. Stratified epithelium has epithelial cells lined up one over another. It is found in the epidermis of the skin.
It helps to prevent wear and tear of tissue. Columnar epithelium consists of cylindrical cells. It is found in the lining of the stomach and intestines, and facilitates the movement across the epithelial barrier.
Columnar epithelial tissue with cilia is known as ciliated epithelium. These cilia push the mucus forward into the nasal tract to clear it. Cuboidal epithelium consists of cubical cells. It is found in the lining of the kidney tubules, salivary glands and thyroid glands, where it provides mechanical support. Glandular epithelium consists of modified columnar cells, and is found in the sweat glands and tear glands to produce secretions.
Connective tissue :
Connective tissues are fibrous in nature.
They include blood, bone, ligament, cartilage, areolar and adipose tissues.
These help in binding other tissues together. They also provide support to other tissues.
Blood has plasma and blood cells.
The blood cells suspended in the plasma include RBC’s, WBC’s and platelets.
Blood flows within blood vessels, and transports gases, digested food, hormones and waste materials to different parts of the body. Bone cells are embedded in a hard matrix composed of calcium and phosphorus compounds.
Bones anchor the muscles and support the main organs of the body. Two bones can be connected to each other by another type of connective tissue called ligament. Ligaments are tough and elastic. They provide strength and flexibility. Tendons connect muscles to bones and are another type of connective tissue. Tendons are tough and non-elastic, and provide great strength and limited flexibility. Cartilage has widely spaced cells suspended in a matrix of proteins and sugars. It is found in the nose, ears, and the rings of the trachea to give flexibility. Areolar connective tissue is found between the skin and muscles, around blood vessels and nerves
and in the bone marrow. It helps in repair of tissues. Adipose tissue contains cells filled with fat globules. It is found below the skin and acts as an
insulator.
Muscular Tissue:
Muscle tissues consists of elongated cells also called muscle fibres.
This tissue is responsible for movement.
Muscles contain special proteins called contractile proteins which contract and relax to cause movement.
These are elastic in nature they have tensile strength.
These muscles can be
voluntary or involuntary in function. Muscular tissues are of three kinds namely striated muscles, unstriated muscles and cardiac muscles. Striated muscle cells are long, cylindrical, unbranched and multinucleate.
These are voluntary muscles.
Smooth muscles or involuntary muscles are found in the iris of the eye, in ureters and in the bronchi of the lungs.
These are also called unstriated muscles. The cells are long with pointed ends and uninucleate.
Hear muscles or cardiac muscles are cylindrical, branched and uninucleate.
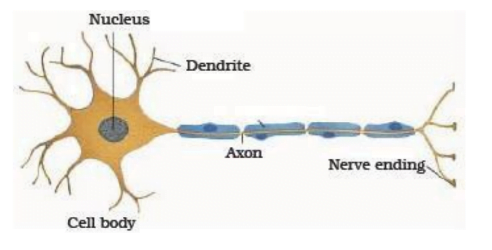
Nervous Tissue
Nervous tissues are found in the brain, spinal cord and nerves.
Nervous tissue is the tissue which works in coordinating the organs of the body by generating impulses.
It is made up of special cells called as neurons.
Each neuron consists of a cell body, which contains a nucleus, cytoplasm, called cyton, from which long thin hair like parts arise.
Usually each neuron has a single long part, called the axon, and many short branched parts called dendrites.

Hindi Version
Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments
Assess
November 5, 2023
THE FUNDAMENTAL UNIT OF LIFE | Study
Assess
Interact
Pre-Requisires
Test & Enrich
Pre – Requisite

English Version
The Fundamental Unit of Life | Speed Notes
Notes For Quick Recap
What are Living organisms made up of?
All living organisms are made up of cells. Cell is the basic structural and functional unit of complex organisms.
History of cell:
Cells were first discovered by Robert Hooke in 1665 with the help of a primitive microscope. Leeuwenhoek, in 1674, with the improved microscope, discovered free-living cells in pond water for the first time. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
Robert Brown in 1831 discovered the nucleus in the cell.
Purkinje in 1839 coined the term ‘protoplasm‘ for the fluid part of the cell.
Schleiden in 1838 and Schwann in 1839 proposed the cell theory which stated that all plants and animals are composed of cells.
Rudolf Virchow in 1855 further expanded the cell theory by suggesting that all cells arise from pre-existing cells.
The invention of magnifying lenses led to the discovery of the microscopic world.
Unicellular organisms are the organisms in which a single cell performs all the functions like nutrition, respiration, excretion and reproduction.
Example: Amoeba, Chlamydomonas, Paramecium and Bacteria possess single cells constituting the whole organism. Multicellular organisms are the organisms which possess many cells to perform different functions.
Multicellular organisms represent themselves as a member of a group of cells or as an individual.
individual.
Example: Fungi, plants and animals have many cells that group together to form tissues.
Every multi cellular organism has come from a single cell. All cells thus come from pre existing cell.
Some organisms can also have cells of different kinds.
The shape and size of cell are related to the specific function they perform.
Some cells change their shapes.
Example: Amoeba. In some cases the cell shape could be more or less fixed and the peculiar for a particular type of cell.
Example: Nerve cells.
Each living cell has the capacity to perform certain basic functions that are characteristic of all living forms.
There is a division of labour in multicellular organism such as human beings.
This means that different parts of the human body perform different functions.
Similarly division of labour is also seen within a single cell. In fact each such cell has got certain specific components
within it known as cell organelles. Each kind of cell organelle performs a special function.
A cell is able to live and perform all its functions because of these organelles.
These organelles together constitute the basic unit called the cell. What is a cell made up of? What is the structural organization of a cell?
Every cell would have three features- plasma membrane, nucleus and cytoplasm.
All activities inside the cell and interactions of the cell with its environment are possible due to these features. Plasma membrane or cell membrane:
This is the outermost covering of the cell that separates the contents of the cell from its external
environment. It is flexible and made up of organic molecules called lipids and proteins.
The flexibility of the cell membrane also enables the cell to engulf in food and other material from its external environment. Such processes are known as endocytosis.
Example: Amoeba It allows the movement of some substances into and out of the cell.
It also prevents movement of
some other materials.
Therefore it is called a selectively permeable membrane. Movement of substances through this semi-permeable membrane can be by the process of diffusion, osmosis etc.
Difference between diffusion and osmosis
If we put an animal cell or a plant cell into a hypotonic solution the cell is likely to swell up.
The cell will stay in the same size if it kept it in isotonic solution.
If the solution is hypertonic then the cell will shrink. Unicellular fresh water organism and most plants tend to gain water through osmosis.
Cell wall: It is present only in plant cells. The cell wall is composed of cellulose and is permeable. It
separates the contents of the cell from the surroundings. It gives shape and protection to the cell. Cell walls permit the cells of plants, fungi and bacteria to withstand very dilute external media without bursting.
Plasmolysis: It is the process in which cells lose water in a hypertonic solution.
Nucleus:
The nucleus has a double layered covering called nuclear membrane. The nuclear membrane has
pores which allow the transfer of material from inside to outside. The nucleus contains
chromosomes which are composed of Deoxyribonucleic acid (DNA) and proteins. Nucleus
controls all the activities of the cell. As the nucleus carries genetic information in the form of DNA, it plays a major role in cell division and cell development. The functional segments of DNA are called genes. Nucleus plays
an important role in protein synthesis and transmission of characters from one generation to
another generation. It plays a central role in cellular reproduction. In some organisms nuclear
membrane is absent and nuclear region contains only nucleic acids called nucleoid. Such
organisms called prokaryotes. Eg. Bacteria. are called eukaryotes. Organisms with cells having a nuclear membrane
Cytoplasm:
The cytoplasm is the fluid content inside the plasma membrane. It is a jelly like viscous substance occupying entire cell except the nucleus. It also contains many specialized cell organelles that perform a specific function for the cell.
Cell organelles:
Cell organelles include endoplasmic reticulum, Ribosomes, Golgi apparatus, Mitochondria, Plastids, Lysosomes, and Vacuoles. They are important because they carry out some very crucial functions in cells.
Endoplasmic reticulum (ER):
The ER is a large network of membrane bound tubes and sheets. It serves as channels for the transport of materials especially proteins between various organs of the cytoplasm or between the cytoplasm and nucleus. It also functions as a cytoplasmic framework providing a surface for some of the biochemical activities of the cell. There are two types of ER- Rough endoplasmic reticulum and smooth endoplasmic reticulum.
RER: These are rough at surface and are associated with ribosomes. These are responsible for the synthesis of proteins. SER: These are smooth at surface and are not associated with ribosomes. It helps in the manufacture of fat molecules or lipids. It also plays a crucial role in detoxifying many poisons and drugs.
Membrane biogenesis: Some of the proteins and lipids synthesized by EF help in building the cell membrane. This process is known as membrane biogenesis.
Golgi Apparatus:
These cell organelles are named after the biologist, Camillo Golgi, who first described it. The Golgi consists of a stack of membrane-bound cisternae. These membranes often have connections with the membranes of ER and therefore constitute another portion of a complex cellular membrane system. Its functions include the storage, modification and packaging of products in vesicles. It is also involved in the formation of lysosomes.
Lysososmes:
Lysosomes are membranous sacs filled with enzymes. These enzymes are made by RER. They are a kind of waste disposal system of the cell. They help to keep the cell clean by digesting any foreign material as well as worn out cell organelles. Lysosomes contain hydrolytic enzymes which are capable of digesting cellular macromolecules. When the cell gets damaged, the lysosome may burst and its enzymes may digest thecell itself. Hence, lysosomes are called as
‘suicidal bags’.
Mitochondria:
These are cellular organelles termed as ‘power houses of the cells’. These are bounded by a double membrane. The outer membrane is smooth while the inner membrane is thrown into folds called as cristae. The cristae increase the area of cellular respiration. Mitochondria releases energy in the form of ATP molecules. ATP is known as the “energy currency of the cell”. Mitochondria have its own DNA DNA ribosomes and are able to make some of their own proteins.
Plastids:
Plastids are present only in plant cells. These are of two types- chromoplasts (coloured plastids) and leucoplasts (white or colourless plastids). Plastid contains pigment called chlorophyll are known as chloroplasts. These are important for photosynthesis in plants. Chromoplasts are the organelles which provide bright colours to the plant structures like buds, flowers etc.
Leucoplasts: are the organelles which store starch, oils and protein granules. Plastids consist of numerous
membrane layers embedded in a material called the stroma. Plastids also have their own DNA
and ribosomes.
Vacuoles: Vacuoles are membrane bound compartments present in both plant and animal cells. These are
storage sacs for solid or liquid contents. These are small sized in animal cells while bigger in plant cell. In plant cells vacuoles are full of sap and provide turgidity and rigidity to the cell. These organelles store water, waste products, and substances like amino acids, sugars and proteins. In some unicellular organisms specialized vacuoles also play important roles in expelling excess water and some wastes from the cell. Difference between plant cells and animal cells
Difference between Plant cells and Animal cells.

Hindi Version
Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments
Assess
November 5, 2023

MATTER IN OUR SURROUNDINGS | Study

Pre-Requisires

Test & Enrich

English Version

Matter In Our Surroundings | Speed Notes

Notes For Quick Recap

Matter:

1. Characteristics of Matter Particles

Anything (Physical Material not emotions, feelings etc.) which has mass and volume (occupy space) is called matter.

We feel the presence of matter by one or more of our five sense organs.

Matter is made up of particles. (Scroll down to ontinue …)

Study Tools

Audio, Visual & Digital Content

Particles:

Particles are very small in size. Therefore we cannot see particles with our naked eye.

Characteristics of the particles of matter:

(1) All matter (elements or compounds) consists of very small particles which can exist independently and are called particles.

(ii) The particles of matter are in a state of continuous motion and possess kinetic energy.

(iii) There are intermolecular spaces in between the particles (molecules) of matter.

(iv) The particles (molecules) of matter attract each other with a force called intermolecular force.

Intermolecular force is maximum in solids and least in the gases.

These material particles can be touched, moved by changing temperature or attracted by decreasing or increasing forces of attraction or repulsion.

2. States of Matter

Matter exists in three different physical states namely solid, liquid and gas.

One substance such as water can exist in all the three states such as, ice in solid state, water in liquid state and steam or vapours in gaseous state.

The state of matter depends on temperature, forces of attraction between their constituent particles etc.

3. Interconversion of Matter

All these three different states of matter are interconvertible depending upon temperature and pressure.

The state of matter can be changed by changing temperature or pressure.

Due to change in temperature and pressure there will be a change in inter-particle space as well as force between them, resulting in change in physical state.

Examples:

Applying pressure and reducing temperature can liquefy gases.
Solid CO₂ gets converted directly to a gaseous state on decrease of pressure to 1 atmosphere without changing into a liquid state. Due to this fact solid CO₂ is also known as DRY ICE.

4. Plasma: It is the fourth state of matter consisting of super energetic and super excited particles. These particles are in the form of ionised gases.

Examples:

The plasma in stars is formed due to high temperature.
Glowing plasma formed in fluorescent tubes and neon sign bulbs.

These devices contain inert gases which get ionised due to the passage of electric current. The colour of the glowing plasma depends upon the nature of the gas.

5. Sublimation: The process in which a solid state directly changes into a gaseous state on heating or vice-versa on cooling.

6. Melting or Fusion: The process of changing a solid into a liquid state by absorbing heat at a constant temperature is known as Melting or Fusion.

7. Freezing or Solidification: The process of changing a liquid into solid state by losing heat at a constant temperature is known as Freezing or Solidification.

8. Condensation: The process of changing a gas into a liquid state by giving out heat at constant temperature is known as Condensation .

Boiling or Vaporisation : The process of changing a liquid into a gaseous state by absorbing heat at constant temperature is known as Boiling or Vaporisation .

Boiling is a bulk phenomenon. Particles from the bulk (whole) of the liquid change into a vapour state.

Evaporation: The phenomenon of changing the physical state from liquid to vapour, at any temperature is called evaporation.

Evaporation is a surface phenomenon. Particles from the surface gain required energy to overcome the forces of attraction present in the liquid and change into the vapour state.

The rate of evaporation depends upon the surface area exposed to the atmosphere, the temperature, the humidity and the wind speed.

Evaporation causes cooling.

Evaporation takes place at all temperatures, below the boiling point of a liquid

Factors affecting evaporation:

• Rate of evaporation increases with increase in surface area.

• Rate of evaporation increases with increase in temperature.

• Rate of evaporation increases with decrease in Humidity.

• Rate of evaporation increases with increase in wind speed.

Latent heat of boiling or Latent heat of Vaporisation: Latent heat of boiling or Latent heat of Vaporisation is the heat energy required to change 1 kg of a liquid to gas at atmospheric pressure at its boiling point.

Kelvin is the SI unit of temperature.

0°C = 273.16 K.

For convenience, we take 0°C = 273 K after rounding off the decimal.

To change a temperature on the Kelvin scale to the Celsius scale you have to subtract 273 from the given temperature, and to convert a temperature on the Celsius scale to the Kelvin scale you have to add 273 to the given temperature.

Conversion Formula: t°C = (t+273) K

Boiling point or Vaporisation point: Boiling point or Vaporisation point is the fixed temperature at which a liquid converts into a gaseous state at atmospheric pressure.

Melting point or Fusion point: Melting point or Fusion point is the temperature at which a solid starts converting into a liquid state at atmospheric pressure.

Evaporation Causes cooling: During evaporation the particles at the surface of the liquid gain energy from the surroundings and change into vapour.. Therefore Evaporation Causes cooling effect.

Sponge can be compressed although it is solid: Sponge contains minute holes in which air is trapped.So when it is pressed, the air gets expelled and the sponge gets compressed. Also,the material of the sponge is not rigid.

Temperature does not change during change of state: The temperature remains constant at its melting and boiling points (during change of state) until all the substance melts or boils.

Because the heat supplied is continuously used up in changing the state of the substance by overcoming the force of attraction between the particles.

There is no increase in the kinetic energy of the particles and thus, temperature does not change.

This heat energy absorbed without showing any rise in temperature is given the name latent heat of fusion/latent heat of vaporisation.

Effect of pressure on physical state of a substance:

If pressure is applied, melting point decreases and boiling point increases

When pressure is increased, the particles come closer and the force of attraction increases between them and this results in a change of state.

Example: When high pressure is applied to a gas by reducing its temperature, the particles of gas come close and get converted to a liquid. This is also known as liquefaction.

The amount of heat energy required in changing a 1 kg of solid into liquid at atmospheric pressure and its melting point is known as the latent heat of fusion.

[ Lice = 80 cal/g = 3.34 × 105 J/kg].

• The amount of heat which is required to convert 1 kg of the liquid (at its boiling point) to vapours of gas without any change in temperature is known as latent heat of vaporisation.

[Lwater =540 cal/g= 22.5 × 105 J/kg].

• The amount of heat absorbed or liberated , Q = mL.

• The specific heat is the amount of heat per unit mass required to raise the temperature by one degree Celsius.

• Q = m.s. t, where m = mass of the body, s = specific heat of the body and t is temperature difference and m.s is called thermal capacity.

• Change of liquid into vapours at any temperature below the boiling point.

Takes the latent heat from the body. Thus, the body cools when evaporation takes place.

Evaporation:

(1) Evaporation is a slow process.

(ii) Evaporation takes place at the surface mass of the liquid.

(iii) Evaporation takes place at all temperatures.

(iv) The substance becomes cool due to evapora- tion process.

(v) Heat is absorbed from the surroundings due to Evaporation. Absorption of heat from the surroundings causes cooling effect.

Boiling:

(1) Boiling is a rapid process.

(ii) Boiling takes place throughout the mass of a liquid.

(iii) Boiling takes place at a definite temperature called the boil- ing point.

(iv) The substance remains hot during the boiling process.

(v) Heat is required from an external source such as a burner for boiling to take place.

Scales of temperature

• Three scales are commonly used for measuring temperature, namely, the Celsius scale, the Fahrenheit scale and the Kelvin scale.

• The relation between the Celsius and the Kelvin scale can be expressed as:

C + 273 = K

• The relation between the Celsius and the Fahrenheit scale can be expressed as follows.

Property	Solid	Liquid	Gas
Inter particle space	Very less	Larger than solid butlesser than gas	Very large
Inter particle force	Very strong	Weaker than solidbut stronger than gas	Very weak
Nature (Rigidity)	Very hard and rigid	Fluid	Highly fluid
Compressibility	Negligible	Very small	Highly compressible
Shape	Definite shape	Indefiniteshape	Indefinite Shape
Volume	Definite Volume	Indefinite shape	Indefinite volume
Density	high	Less than solid	Very low
Kinetic energy	low	high	Very high
Diffusion	Negligible	Slow	Very high

Specific Heat

11.8 NATURAL PHENOMENA AND CONSEQUENCES OF HIGH SPECIFIC HEAT CAPACITY OF WATER

Some consequences of high specific heat capacity of water are given below.

(i) The climate near the seashore is moderate :

The specific heat capacity of water is very high (= 1000 cal kg-1 °C-1 or 4200 J kg-1 K-¹). It is about five times as high as that of sand. Hence the heat energy required for the same rise in temperature by a certain mass of water will be nearly five times that required by the same mass of sand.

Similarly, a certain mass of water will give out nearly five times more heat energy than that given by sand of the same mass for the same fall in temperature.

As such, sand (or earth) gets heated or cooled more rapidly as compared to water under similar conditions.

Thus, a large difference in temperature is developed between the land and the sea due to which land and sea breezes are formed”. These breezes make the climate near the seashore moderate.

(ii) Hot water bottles are used for fomentation: The reason is that water does not cool quickly due to its large specific heat capacity, so a hot water bottle provides heat energy for fomentation for a long time.

(iii) Water is used as an effective coolant: By allowing water to flow in pipes around the heated parts of a machine, heat energy from such parts is removed (e.g. radiators in car and generator are filled with water). Water in pipes extracts more heat from surroundings without much rise in its temperature because of its large specific heat capacity.

(iv) In cold countries, water is used as a heat reservoir for wine and juice bottles to avoid their freezing: The reason is that water due to its high specific heat capacity can impart a large amount of heat before reaching up to the freezing temperature. Hence bottles kept in water remain warm and they do not freeze even when the surrounding temperature falls considerably.

(v) Farmers fill their fields with water to protect the crops from frost: In the absence of water, if on a cold winter night, the atmospheric temperature falls below 0°C, the water in the fine capillaries of plants will freeze, so the veins will burst due to the increase in volume of water on freezing. As a result, plants will die and the crop will be destroyed. In order to save crop on such cold nights, farmers fill their fields with water because water has a high specific heat capacity, so it does not allow the temperature in the surrounding area of plants to fall up to 0°C.

(vi) All plants and animals have a high content of water in their bodies: All plants and animals have nearly 80% to 90% of water in their bodies so it helps in maintaining the body temperature nearly same in all seasons due to high specific heat capacity of water.

SOME EXAMPLES OF HIGH AND LOW THERMAL CAPACITY

(1) The base of a cooking pan is made thick : By making the base of the cooking pan thick, its thermal capacity becomes large and it imparts sufficient heat energy at a low temperature to the food for its proper cooking. Further it keeps the food warm for a long time, after cooking.

(2) The base of an electric iron is made thick and heavy: By doing so, the thermal capacity of the base becomes large and it remains hot for a long duration even after switching off the current.

(3) The vessel used for measurement of heat (i.e., calorimeter) is made of thin sheet of copper:

The reason is that the specific heat capacity of copper is low and by making the vessel thin, its thermal capacity becomes low so that it takes a negligible amount of heat from its contents to attain the temperature of the contents.

Hindi Version

Dig Deep

Topic Level Resources

Sub – Topics

Select A Topic

Topic:

Chapters Index

Select Another Chapter

Assessments

Personalised Assessments

IS MATTER AROUND US PURE | Study

Pre-Requisires

Test & Enrich

English Version

Speed Notes

Notes For Quick Recap

Matter: Anything that occupies space is called matter.

Example: Air, water, rock etc.,

Matter exists in our surroundings in both pure and impure forms. (Scroll down to continue …).

Study Tools

Audio, Visual & Digital Content

Mixture: A mixture is a matter that contains more than one pure substance in any ratio/proportion.

A mixture is an impure form of matter.

Example:

Water in milk, lemon juice, Ginger Garlic paste, etc.,

The mixture may or may not be separated into its constituent particles by physical processes.

Substance: A matter that cannot be separated into its constituent particles by any physical process is known as a substance.

Example:

Solution: A homogeneous mixture of two or more substances is called a solution.

Example:

Tea, sugar, and common salt are dissolved in water.

Alloy: A homogeneous mixture of metals is called an alloy.

Properties of the Solution:

A solution is a homogeneous mixture
Particles are extremely small, not visible to the naked eye
The light path is invisible in solution.
Solute particles cannot be separated by filtration

Concentration of solution: The concentration of a solution is the amount of solute present in a given quantity of the solution.

Unsaturated and Saturated Solutions: a solution in which a larger quantity of solute can be dissolved without raising its temperature, is called an unsaturated solution.

• A solution in which no more solute can be dissolved at a certain temperature, is called a saturated solution.

Solubility: The maximum amount of a solute that can be dissolved in 100 grams of a solvent at a specified temperature is known as the solubility of the solute in that solvent.

Suspension: a heterogeneous mixture of solids and liquids where the solid particles are suspended throughout the medium.

Example: Mixture of chalk powder and water

Properties of Suspension

• Particles are visible to the naked eye

• Light path in a suspension is visible

• Particles settle down

Colloidal Solution: Colloidal Solution Is a heterogeneous mixture, but appears to be homogeneous.

Examples: Milk, soap lather, soda water, pumice stone, rubber, bread, fog, cloud, insecticide spray, butter, etc.

Properties of colloidal solutions

• Heterogeneous mixture

• Particle size is small, not visible to the naked eye

• Light path can be visible;

• Particles do not settle down

• Substances cannot be separated by filtration

Tyndall Effect: Scattering of light beam by suspended particles in the solution.

Physical and Chemical changes:

Physical and change: The changes in which no new substances are formed are called physical changes.

Chemical change: The changes in which new substances are formed are called chemical changes.

SEPARATION OF MIXTURES

The method of separation depends on both the type of mixture and the physical properties of its constituents.

These are :

(i) The physical state of the constituents.

(ii) The differences in the physical properties

of the constituents, such as:

(a) boiling point

(b) melting point

(d) magnetic properties

(e) ability to sublime

(f) volatility

(g) solubility in various solvents.

• Evaporation: Used for separating mixtures of volatile solvents and non-volatile solutes.

Working Principle:

One component should be non-volatile. It may or may not be soluble in water.

Example: Separating salt from its solution

• Centrifugation used for separating components based on the difference in their weights.

Working Principle:

Difference in the densities of two liquids.

Example: Separating mixtures of cream from milk

• Separating Funnel: Used for separating two or more immiscible liquids.

Working Principle:

Immiscible liquids with different densities get separated into different layers if they are in the same container.

Example: Separating oil and water

Sublimation:

Sublimation is the process of converting a solid into vapour and returning it to the solid state without passing through the liquid state.

Sublimation is used to separate sublimable solids from their mixtures.

Working Principle:

One of the components can be sublime.

Example: Separating ammonium chloride from a mixture

Chromatography:

The process of separating the different dissolved constituents of a mixture by their adsorption (adsorption refers to the collection of one substance on the surface of another substance.) over an appropriate adsorbing material is called chromatography.

Chromatography is used to separate those solutes that dissolve in the same solvent.

Working Principle:

Adsorption/partition

Example: Separating the components of a dye

Distillation:

Distillation is the process of heating a liquid to convert it into vapours and then condensing the vapours back into a liquid.

Distillation is used to separate two miscible liquids that boil without decomposition.

Working Principle:

One component should be a soluble solid in a liquid.

Example: Separating a mixture of acetone and water

Fractional distillation

Fractional distillation is a process that involves the distillation and collection of fractions or different liquids boiling at different temperatures.

Fractional distillation is used to separate a mixture of liquids when their boiling temperatures differ by less than 25 K.

Example: Separating different components of petroleum

Crystallization: Used to separate pure solids from a solution by forming crystals.

Working Principle:

A solid dissolved in a liquid is separated by evaporating the solvent completely by heating the mixture.

Example: Obtaining pure crystals of copper sulphate from an impure sample.

Differences Between Mixture And Compound

Property	Mixture	Compound
Nature	When two or more elements or compounds or both are mixed together, such that they do not combine chemically, a mixture is formed.	When two or more elements unitechemically, a compound is formed.
Structure	Mixtures are generally heterogeneous. However, some mixtures can be homogeneous.	Compounds are always homogeneous.
Composition	In case of mixtures their constituents can be present in any ratio, i.e., mixtures havevariable composition.	In case of compounds, the constituents arepresent in a fixed ratio by weight.
Properties	The constituents of a mixture retain theirindividual chemical and physical properties.	The properties of a compound are entirelydifferent from the properties of itsconstituents
Separation of constituents	The constituents of a mixture can beseparated by applying physical methods likesolubility, filtration, evaporation, distillation,use of magnet, etc.	The constituents of a compound cannot beseparated by applying physical methods.However, constituents of a compound can beseparated by chemical means.
Energy change	There may or may not be energy changeduring the formation of mixture.	During the formation of a compound eitherthe energy is absorbed or given out.

Type of Mixture	Nature of Mixture	Example	Separation Method
Solid – solid	Heterogeneous	Iron + Sand;	Magnetic separation
Solid – solid	Heterogeneous	Iodine + Sand	Sublimation
Solid – solid	Heterogeneous	Iron + Sulphur	Solvent extraction
Solid – solid	Heterogeneous	Nitre + Common salt	Fractional crystallisation
Solid – liquid	Heterogeneous	Sand+Water; Clay + Water	Sedimentation-decantation
Solid – liquid	Heterogeneous	Chalk + Water; PbCl₂ + Water	Filtration
Solid – liquid	Homogeneous	Common salt in seawater	Evaporation
Solid – liquid	Homogeneous	Iodine + Methyl alcohol	Distillation
Liquid – liquid	Homogeneous	Methyl alcohol + Ethyl alcohol	Fractional distillation
Liquid – liquid	Homogeneous	Oil + Water; Mercury + Water	Separating funnel
Liquid – gas	Homogeneous	Ammonia + Water	Boiling of liquid
Complex Mixture	Homogeneous	Colouring matter in ink	Chromatography

Video	Link	Remarks
	TRM Link
	TRM Link
	TRM Link
	TRM Link

Hindi Version

Dig Deep

Topic Level Resources

Sub – Topics

Select A Topic

Topic:

Chapters Index

Select Another Chapter

Assessments

Personalised Assessments

ATOMS AND MOLECULES | Study
Assess
Interact
Pre-Requisires
Test & Enrich
Pre – Requisite

English Version
Speed Notes
Notes For Quick Recap
Atoms are the basic building blocks of matter.
Different kinds of matter contain different kinds of atoms present in them.
Protons were discovered by Ernest Rutherford, in his famous gold foil experiment.
Electrons were discovered by J.J. Thomson, in his cathode ray tube experiment.
Neutrons were discovered by James Chadwick. (Scroll down to continue …).
Study Tools
Audio, Visual & Digital Content
Laws of Chemical Combination: Antoine Laurent Lavoisier, is known as ‘Father of Modern Chemistry.
Lavoisier put forward the law of conservation of mass, which laid the foundation of chemical sciences.
Law of Conservation of Mass:Law of Conservation of Mass states that, “mass is neither created nor destroyed in a chemical reaction.
In other words, the mass of the reactants must be equal to the mass of products.
Law of Constant Proportions or Definite Composition: Law of Constant Proportions or Definite Composition states that, in a pure chemical substance, the elements are always present in definite proportions by mass.
Dalton’s Atomic Theory:
(i) Every element is composed of extremely small particles called atoms.
(ii) Atoms of a given element are identical, both in mass and properties.
(iiii) Different chemical elements have different kinds of atoms; in particular, their atoms have different masses.
(iv) The atoms neither be created nor be destroyed or transformed into atoms of other elements.
(v) Compounds are formed when atoms of different elements combine with each other in small whole number ratios.
(vi) The relative number and kinds of atoms in a given compound are constant.
Drawbacks of Dalton’s Atomic Theory:
(i) According to modern theory, an atom is not the ultimate indivisible particle of matter. Today, we know that atoms are divisible, they are themselves made-up of particles (protons, electrons, neutrons,etc.).
(i) In the case of isotopes of an element, the assumption that the atoms of the same element have the same mass does not hold good.
Atom: It is the smallest particle of an element that maintains its chemical identity throughout all chemical and physical changes.
The smallest unit of a substance which can exist independently is called a molecule.
Atomicity: It is defined as the number of atoms present in a molecule of an element or a compound.
Mono atomic: Molecule having only one atom is called mono atomic,
e.g., He, Ne, Ar.
Diatomic: Molecules made-up of two atoms are called diatomic, e.g., H₂, Cl₂, O₂, N₂.
Triatomic: Molecules made-up of three atoms, called triatomic.
e.g., O₃, H_₂O, NO₂.
Tetraatomic : Molecules made-up of four atoms, called tetra atomic.
e.g., P₄, NH₃, SO₃
Polyatomic: Molecules made-up of five or more atoms, called polyatomic/
e.g., CH₄.
Polyatomic: Any molecule which is made-up of more than four atoms is called polyatomic,
e.g., Sg.
Relative Atomic Mass: It is defined as the number of times one atom of an element is heavier than
(1/12)th of the mass of an atom of Carbon – 12.
Relative Atomic Mass (RAM) = Mass of an atom of an element/
¹/12 th mass of C-12
Molecular Mass: The molecular mass of a substance is the sum of the atomic masses of all atoms in a molecule of a substance,
e.g., molecular mass of water is 18 u.
The mole (or mol) is the SI unit of the amount of a substance. One mole is equal to the amount of substance that contains as many elementary units as there are atoms in 12 g of the carbon-12 isotope.
The elementary units may be atoms, molecules, ions, radicals, electrons, etc., and must be specified.
This number is called Avogadro’s number (No) or Avogadro’s constant
[NA = 6.0221367 x 10²³]. Generally,
Avogadro’s Number is rounded to 6.022 x 10²³.
For better understanding we can compare avogadro number with a dozen as:
One dozen oranges contain 12 oranges, similarly, 1 mole of hydrogen atoms contain 6.022 x 10²³ H atoms.
H₂O = 2 x H + 1 × O
= 2 x 1+1 x 16 = 2+16
= 18 amu or u.
By : 1 mole of a compound has a mass equal to its relative molecular mass expressed in grams.
1 mole = 6.022 × 10²³ number
= Relative mass in grams.
A molecule is the smallest particle of an element or a compound capable of independent existence under ordinary conditions. It shows all the properties of the substance.
A chemical formula of a compound shows its constituent elements and the number of atoms of each combining element.
Clusters of atoms that act as an ion are called polyatomic ions. They carry a fixed charge on them.
The chemical formula of a molecular compound is determined by the valency of each element.
In ionic compounds, the charge on each ion is used to determine the chemical formula of the compound.
Scientists use the relative atomic mass scale to compare the masses of different atoms of elements. Atoms of carbon-12 isotopes are assigned a relative atomic mass of 12 and the relative masses of all other atoms are obtained by comparison with the mass of a carbon-12 atom.
The Avogadro constant 6.022 × 10²³ is defined as the number of atoms in exactly 12 g of carbon-12.
The mole is the amount of substance that contains the same number of particles (atoms/ions/ molecules/formula units, etc.) as there are atoms in exactly 12g of carbon-12. Mass of 1 mole of a substance is called its molar mass.
The relative atomic mass of the atom of an element is the average mass of the atom as compared to 1/12th mass of one carbon-12 atom.
Hint: We know that chemical formulas can also be written using a criss-cross method. In the criss-cross method, the numerical value of the ion charge of the two atoms is crossed over, which becomes the subscript of the other ion. Using this technique, we will write the chemical formula of the given compounds.
Complete step by step answer:
Let’s us discuss about the given compound as,
A.Magnesium chloride
We have to remember that the atomic number of Magnesium is 12 and has a valency of 2.
It means it has two electrons in the outermost shell for bonding.
The atomic number of chlorine is 17 and has 7 electrons in the outermost shell.
It means it just needs one more atom for bonding.
Hence, we will use atoms of chlorine to bond with one atom of magnesium.
We can apply the criss-cross method for this compound as,
Therefore, the chemical formula of magnesium chloride is MgCl2
B.Calcium oxide
We have to know that the atomic number of calcium is 20 and has a valency of 2, it means it has 2 two atoms in the outermost shell for bonding.
The atomic number of Oxygen is 8
8 and has a valency of 2, it has 6 atoms in the outermost shell, it needs 2 more to complete the octet.
Hence, we need one calcium atom to bond with one oxygen atom.
We can apply the criss-cross method for this compound as,
Therefore, the chemical formula of magnesium chloride is CaO
C. Copper nitrate
We have to know that the atomic number of copper is 29 and has two atoms in the outermost shell for bonding. While a nitrate molecule has only one valence electron.
We need 2 nitrate molecules to satisfy the valency of 1 copper atom.
We can apply the criss-cross method for this compound as,
Therefore, the chemical formula of magnesium chloride is
Cu(NO3)2
D.Aluminium chloride
We have to know that the atomic number of aluminium is 13 and has a valency of 3 atoms and chlorine atom has a valency of 1. Since it has 7 electrons in the outermost shell.
Thus, we need 3 chlorine atoms to satisfy the valency of 1 aluminium atom.
We can apply the criss-cross method for this compound as,
Therefore, the chemical formula of magnesium chloride is AlCl3.
E.Potassium nitrate
We have to remember that the atomic number of potassium is 19 and has a valency of 1 and nitrate also has a valency of 1, since it needs one more atom to complete its octet. Hence, we need only one molecule of nitrate for one atom of potassium.
We can apply the criss-cross method for this compound as,
Therefore, the chemical formula of magnesium chloride is KNO3.
Note: As we know that the criss-cross method is the most efficient way to write the correct chemical formula of the molecule. It is generally used for finding out the formula of a bonding of a metal with a non-metal to form ionic bonds. Signs of the two ions are dropped, the ion value is crossed which becomes the subscript of the crossed atoms.
Hindi Version
Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments
Assess
November 5, 2023

STRUCTURE OF THE ATOM | Study

Pre-Requisires

Test & Enrich

English Version

Speed Notes

Notes For Quick Recap

Atoms are the basic building blocks of matter.
Different kinds of matter contain different kinds of atoms present in them.
Protons were discovered by Ernest Rutherford, in his famous gold foil experiment.
Electrons were discovered by J.J. Thomson, in his cathode ray tube experiment.
Neutrons were discovered by James Chadwick. (Scroll down to continue …)

Study Tools

Audio, Visual & Digital Content

Charged Particles in Matter

Whenever we rub two objects together, they become electrically charged.
This is because atoms contain charged particles in them.
Therefore, atoms can be divided further into particles i.e proton, electron and neutron.

Atoms consist of an equal number of protons and electrons.

Protons exist in the interiors of the atom and electrons exist in the exteriors of the atom. Therefore, electrons can be removed from an atom.

Since electrons exist in the exteriors of the atom they can be removed from an atom.

Dalton’s Atomic Theory

The postulates of the atomic theory by John Dalton

The matter is made up of tiny particles called Atoms that cannot be divided.
Atoms are never formed or destroyed during a chemical reaction.
Atoms of an element exhibit the same nature.
Atoms of the same element have equal size, mass and they exhibit similar chemical properties.
Atoms of different elements exhibit variant chemical properties.
Atoms form compounds by combining in a ratio of whole numbers.
A compound contains molecules in which a constant number and types of atoms are present.

Failure of Dalton’s Atomic Theory

Dalton suggested that atoms can neither be created nor destroyed and are indivisible.

But the discovery of electrons and protons in atoms disproved this aspect of Dalton’s theory.

Thomson’s Model of an Atom

According to J.J. Thomson, the structure of an atom can be compared to Christmas pudding.

According to this model the electrons are present inside a positive sphere. Thomson’s Model of an Atom

An atom is composed of a positively charged sphere in which electrons are embedded.

Atoms are neutral as the positive and negative charges are equal in number.

Rutherford’s Model of an Atom

Rutherford’s Experiment

Rutherford experimented by passing alpha rays through a thin gold foil.

He expected that the gold atoms would deflect the Alpha particles.

Observations	Inferences
Alpha particles which had high speed moved straight through the gold foil	Atom contains a lot of empty space
Some particles got diverted a by small angles	Positive charges in the atom are not occupying much of its space
Only one out of 12000 particles bounced back	The positive charges are concentrated over a particular area of the atom.

Based on his experiment Rutherford gave the nuclear model of an atom as the following.

Rutherford’s Atomic Model

Rutherford’s Atomic Model is known as Planetary Atomic Model and Nuclear Atomic Model.

According to Rutherford’s Atomic Model:

Atoms contain a lot of unoccupied space
The center of the atom is highly positive , Rutherford named it as nucleus
The atom contains an equal amount of positive and negative charges.

Nucleus of Atom

The nucleus is located at the center of the atom.

All the mass of the atom is because of the nucleus.

The electrons revolve around the nucleus in circular parts which called Orbits

The size of an atomic nucleus is much smaller than its atom.

Drawbacks of the Nuclear Atomic Model

The Rutherford’s Atomic Model failed to explain how an atom remains stable despite having positive and negative charges present in it.

Maxwell’s theory of radiation if any charged particle moves in a circular motion it radiates energy.

So, if electrons move in a circular motion around the nucleus they should radiate some energy as a result this decreases at the speed of the electrons. As a result, they would fall into the nucleus and the nucleus should collapse because of its high positive charge.

But it is not happening because the matter is not collapsing.

Nucleons: The subatomic particles present in the nucleus are collectively called Nucleons. Protons and Neutrons are nucleons.

Bohr’s Model of an Atom

Bohr Atomic Model states as the following: Bohr's Model of an Atom

Electrons revolve around the nucleus in particular circular paths, called orbits.
The electrons do not emit any energy while moving in their orbits.
The orbits are also called Energy Levels.
Energy Levels or Orbits are represented by using letters or numbers as shown in the figure.

Neutron:

J. Chadwick discovered Neutron, a subatomic particle of an atom.

Neutron carries no charge.

Subatomic Particles of Atom

Electrons	Electron carry a negative charge
Protons	Protons carry a positive charge
Neutrons	Neutrons are neutral

Electronic Configuration: The distribution of electrons in different shells or orbits is called Electronic Configuration.

If Orbit number = n
Then number of electrons present in an Orbit = 2n²
So, for n =1
Maximum electrons present in shell – K = 2 * (1)² = 2
The outermost shell can contain at most 8 electrons.
The shells in an atom are filled in sequence.
Thus, until the inner shells of an atom are filled completely the outer shells cannot contain any electrons.

Valency

Valence Electrons – Electrons existing in the outermost orbit of an atom are called Valence Electrons.
The atoms which have completely filled the outermost shell are not very active chemically.
The valency of an atom or the combining capacity of an atom is given by the number of elements present in the outermost shell.
For Example, Helium contains two electrons in its outermost shell which means its valency is two. In other words, it can share two electrons to form a chemical bond with another element.
What happens when the outermost shell contains a number of electrons that are close to its maximum capacity?

Valency in such cases is generated by subtracting the number of electrons present in the outermost orbit from octet (8). For example, oxygen contains 6 electrons in its outermost shell. Its valency is calculated as: 8 – 6 = 2. This means oxygen needs two electrons to form a bond with another element.

Representation Element: Atomic symbol Notation

Atomic Number of an Element

Atomic Number (Z) = Number of protons in an atom

Mass Number of an Element

Mass Number = Number of protons + Number of neutrons

Isotopes

The atoms of an element can exist in several forms having similar atomic numbers but varying mass numbers.
Isotopes are pure substances.
Isotopes have a similar chemical nature.
Isotopes have distinct physical characteristics.

Use of Isotopes:

1. The fuel of Nuclear Reactor – Isotope of Uranium

2. Treatment of Cancer – Isotope of Cobalt

3. Treatment of Goiter – Isotope of Iodine

Example: Consider two atomic species namely U and V. Are they isotopes?

	U	V
Protons	5	5
Neutrons	5	6
Mass Number	5 + 5 = 10	5 + 6 = 11
Atomic Number	5	5

From the above example, we can infer that U and V are isotopes because their atomic number is the same.

Isobars

The atoms of several elements can have a similar mass number but distinct atomic masses. Such elements are called Isobars. Isobars

Hindi Version

Dig Deep

Topic Level Resources

Sub – Topics

Select A Topic

Topic:

Chapters Index

Select Another Chapter

Assessments

Personalised Assessments

CBSE 9 | Mathematics – Study – Premium
Educational Tools | Full Course
Study Tools
Audio, Visual & Digital Content
Assessment Tools
Assign, Assess & Analyse
November 5, 2023

LINES AND ANGLES | Study

Pre-Requisires

Test & Enrich

English Version

Lines And Angles | Speed Notes

Notes For Quick Recap

Basic Terms And Definitions

1. Point – A Point is that which has no component. It is represented by a dot.

A point is shown with a capital letter.

Examples: A, B, C …..

Colinear And Non-colinear Points

Collinear and Non-collinear points

5. Collinear points: Points lying on the same line are called Collinear Points.

Non-collinear points: Points which do not lie on the same line are called Non-Collinear Points.

Line – When we join two distinct points then we get a line. A line has no endpoints; it can be extended on both sides infinitely.

Line Segment Line – Segment is the part of the line which has two endpoints.

Ray – Ray is also a part of the line that has only one endpoint and has no end on the other side. (Scroll to continue …)

Study Tools

Audio, Visual & Digital Content

Lines And Their Types

Ray

A Ray is a straight path that stars at a point and extends infinitely in one direction.

Note: A ray is a portion of line starting at a point and extends in one direction endlessly. A ray has only one endpoint (Initial point).

Line or Straight Line

A line is a straight path that extends infinitely in two opposite directions. It can be treated as a combination of two rays starting from the same point but extending in the opposite directions.

Note: A line has no end points.

Line Segment:

A line segment is the part of a line between two points. (Segment means part).

The length of a line segment is the shortest length between two endpoints.

The line segment has two endpoints. Note: A line Segment has two endpoints. (both Initial and end points).

Intersecting Lines and Non-intersecting Lines

Intersecting Lines

Lines that meet or cross at a point with each other are called intersecting lines Or Non-parallel lines.

Intersecting lines meet at a point.

Parallel Lines

Lines that are always the same distance apart from each other and that never meet are called Parallel lines or Non-intersecting lines.

Note: Parallel lines do not have any common point.

Angles

When two rays begin from the same endpoint then they form an Angle. The two rays are the arms of the angle and the endpoint is the vertex of the angle.

Types of Angles By Measure

Complementary and Supplementary Angles

Complementary Angles are the different angles whose sum is 90°.

Suplementary Angles are the different angles whose sum is 180°

The sum of two adjacent angles formed by that ray is 180°

Angles Based On Position

Adjacent Angles: Two angles that share a common sideare called Adjacent Angles.

Linear Pair: A pair of adjacent angles whose non-common sides form a straight line (i.e., they are supplementary and add up to 180°).

Vertical (Opposite) Angles:

Angles opposite each other when two lines intersect. They are always equal.

Angles:

An angle is formed by two rays(called the sides or arms of the angle) with a common endpoint called the vertex.

Angles are measured in degrees(°) or radians, with a full rotation being 360°.

Special Angle Pairs:

Complementary Angles:

Two angles whose measures add up to 90°.

Supplementary Angles: Two angles whose measures add up to 180°

Angles in Polygons:

Interior Angles: Angles on the inside of a polygon. The sum of the interior angles of an n-sided polygon is \((n-2) \times 180°\).

Exterior Angles: Angles on the outside of a polygon. The sum of the exterior angles of any polygon is always 360°.

Angles Formed by Parallel Lines and Transversals:

When a transversal intersects two parallel lines, several angle pairs are formed:

Corresponding Angles: Angles in the same position relative to the two lines and the transversal. They are equal.

Alternate Interior Angles: Angles on opposite sides of the transversal and inside the parallel lines. They are equal.

Alternate Exterior Angles: Angles on opposite sides of the transversal and outside the parallel lines. They are equal.

– **Consecutive (Same-Side) Interior Angles:** Angles on the same side of the transversal and inside the parallel lines. They are supplementary.

Measuring Angles:

Protractor: A tool used to measure angles in degrees.

Radians: Another unit of angle measure. One full revolution (360°) is equal to \(2\pi\) radians.

Angle Relationships in Circles:

Central Angle: An angle whose vertex is the centre of the circle. The measure of a central angle is equal to the measure of the arc it intercepts.

Inscribed Angle: An angle whose vertex is on the circle and whose sides contain chords of the circle. The measure of an inscribed angle is half the measure of the intercepted arc.

Angles Formed by Tangents and Chords: The measure of the angle formed by a tangent and a chord through the point of contact is half the measure of the intercepted arc.

Angles Inside the Circle (but not at the centre): The measure of an angle formed by two intersecting chords is half the sum of the measures of the arcs intercepted by the angle and its vertical angle.

Trigonometric Angles:

Standard Position: An angle with its vertex at the origin and one side on the positive x-axis.

Reference Angle: The acute angle formed by the terminal side of an angle and the x-axis.

Quadrantal Angles: Angles that are multiples of 90° (0°, 90°, 180°, 270°, 360°).

Angle Conversions:

Degrees to Radians: Multiply the number of degrees by \(\frac{\pi}{180}\).

Radians to Degrees: Multiply the number of radians by \(\frac{180}{\pi}\).

Key Properties and Theorems:

Angle Sum Property of a Triangle:

The sum of the interior angles of a triangle is always 180°.

Exterior Angle Theorem:

The measure of an exterior angle of a triangle is equal to the sum of the measures of the two non-adjacent interior angles.

Polygon Interior Angles Theorem:

The sum of the interior angles of an n-sided polygon is \((n-2) \times 180°\).

Understanding these fundamental aspects of angles will enhance your comprehension of geometric principles and their applications.

Pairs of Angles Axioms

1. If a ray stands on a line, then the sum of two adjacent angles formed by that ray is 180°.

This shows that the common arm of the two angles is the ray which is standing on a line and the two adjacent angles are the linear pair of the angles. As the sum of two angles is 180° so these are supplementary angles too.

2. If the sum of two adjacent angles is 180°, then the arms which are not common of the angles form a line.

This is the reverse of the first axiom which says that the opposite is also true.

Vertically opposite Angles Theorem

When two lines intersect each other, then the vertically opposite angles so formed will be equal.

AC and BD are intersecting each other so ∠AOD = ∠BOC and ∠AOB = DOC.

Parallel Lines and a Transversal

If a line passes through two distinct lines and intersects them at distant points then this line is called Transversal Line.

Here line “l” is transversal of line m and n.

Exterior Angles – ∠1, ∠2, ∠7 and ∠8

Interior Angles – ∠3, ∠4, ∠5 and ∠6

Pairs of angles formed when a transversal intersects two lines-

1. Corresponding Angles:

∠ 1 and ∠ 5
∠ 2 and ∠ 6
∠ 4 and ∠ 8
∠ 3 and ∠ 7

2. Alternate Interior Angles:

∠ 4 and ∠ 6
∠ 3 and ∠ 5

3. Alternate Exterior Angles:

∠ 1 and ∠ 7
∠ 2 and ∠ 8

4. Interior Angles on the same side of the transversal:

∠ 4 and ∠ 5
∠ 3 and ∠ 6

Transversal Axioms

1. If a transversal intersects two parallel lines, then

Each pair of corresponding angles will be equal.
Each pair of alternate interior angles will be equal.
Each pair of interior angles on the same side of the transversal will be supplementary.

2. If a transversal intersects two lines in such a way that

Corresponding angles are equal then these two lines will be parallel to each other.
Alternate interior angles are equal then the two lines will be parallel.
Interior angles on the same side of the transversal are supplementary then the two lines will be parallel.

Lines Parallel To The Same Line

If two lines are parallel with a common line then these two lines will also be parallel to each other.

As in the above figure if AB ∥ CD and EF ∥ CD then AB ∥ EF.

Angle Sum Property of a Triangle

1. The sum of the angles of a triangle is 180º.

∠A + ∠B + ∠C = 180°

2. If we produce any side of a triangle, then the exterior angle formed is equal to the sum of the two interior opposite angles.

∠BCD = ∠BAC + ∠ABC.

Hindi Version

Dig Deep

Topic Level Resources

Sub – Topics

Select A Topic

Topic:

Chapters Index

Select Another Chapter

Assessments

Personalised Assessments

QUADRILATERALS | Study

Pre-Requisires

Test & Enrich

English Version

Quadrilaterals | Speed Notes

Notes For Quick Recap

Quadrilateral

Any closed polygon with four sides, four angles and four vertices are called Quadrilateral. It could be regular or irregular. (Sroll down to continute …)

Study Tools

Audio, Visual & Digital Content

Revision Notes – CBSE 09 Math – Quadrilaterals

Angle Sum Property of a Quadrilateral

The sum of the four angles of a quadrilateral is 360°

If we draw a diagonal in the quadrilateral, it divides it into two triangles.

And we know the angle sum property of a triangle i.e. the sum of all the three angles of a triangle is 180°.

The sum of angles of ∆ADC = 180°.

The sum of angles of ∆ABC = 180°.

By adding both we get ∠A + ∠B + ∠C + ∠D = 360°

Hence, the sum of the four angles of a quadrilateral is 360°.

Example

Find ∠A and ∠D, if BC∥ AD and ∠B = 52° and ∠C = 60° in the quadrilateral ABCD.

Solution:

Given BC ∥ AD, so ∠A and ∠B are consecutive interior angles.

So ∠A + ∠B = 180° (Sum of consecutive interior angles is 180°).

∠B = 52°

∠A = 180°- 52° = 128°

∠A + ∠B + ∠C + ∠D = 360° (Sum of the four angles of a quadrilateral is 360°).

∠C = 60°

128° + 52° + 60° + ∠D = 360°

∠D = 120°

∴ ∠A = 128° and ∠D = 120 °.

Types of Quadrilaterals

Remark: A square, Rectangle and Rhombus are also a parallelogram.

Properties of a Parallelogram

Theorem 1: When we divide a parallelogram into two parts diagonally then it divides it into two congruent triangles.

∆ABD ≅ ∆CDB

Theorem 2: In a parallelogram, opposite sides will always be equal.

Theorem 3: A quadrilateral will be a parallelogram if each pair of its opposite sides will be equal.

Here, AD = BC and AB = DC

Then ABCD is a parallelogram.

Theorem 4: In a parallelogram, opposite angles are equal.

In ABCD, ∠A = ∠C and ∠B = ∠D

Theorem 5: In a quadrilateral, if each pair of opposite angles is equal, then it is said to be a parallelogram. This is the reverse of Theorem 4.

Theorem 6: The diagonals of a parallelogram bisect each other.

Here, AC and BD are the diagonals of the parallelogram ABCD.

So the bisect each other at the centre.

DE = EB and AE = EC

Theorem 7: When the diagonals of the given quadrilateral bisect each other, then it is a parallelogram.

This is the reverse of the theorem 6.

The Mid-point Theorem

1. If a line segment joins the midpoints of the two sides of the triangle then it will be parallel to the third side of the triangle.

If AB = BC and CD = DE then BD ∥ AE.

2. If a line starts from the midpoint of one line and that line is parallel to the third line then it will intersect the midpoint of the third line.

If D is the midpoint of AB and DE∥ BC then E is the midpoint of AC.

Example

Prove that C is the midpoint of BF if ABFE is a trapezium and AB ∥ EF.D is the midpoint of AE and EF∥ DC.

Solution:

Let BE cut DC at a point G.

Now in ∆AEB, D is the midpoint of AE and DG ∥ AB.

By midpoint theorem, G is the midpoint of EB.

Again in ∆BEF, G is the midpoint of BE and GC∥ EF.

So, by midpoint theorem C is the midpoint of BF.

Hence proved.

Hindi Version

Dig Deep

Topic Level Resources

Sub – Topics

Select A Topic

Topic:

Chapters Index

Select Another Chapter

Assessments

Personalised Assessments

HERON’S FORMULA | Study
Assess
Interact
Pre-Requisires
Test & Enrich
Pre – Requisite

English Version
Heron’s Formula | Speed Notes
Notes For Quick Recap
Perimeter
Perimeter is defined as the outside boundary of any closed shape.
To calculate the perimeter of a given shape we need to add all the sides of the shape.
Example: The perimeter of a rectangle is the sum of its all four sides. The unit of the perimeter is the same as its length.
Perimeter of the Given rectangle = 3 + 7 + 3 + 7 cm
Perimeter of rectangle = 20 cm. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
Area
Area of any closed figure is the surface enclosed by the perimeter. Unit of Area is the square of the unit of length.
Area of a triangle
The general formula to find the area of a triangle, if the height is given, is
Area of a Right Angled Triangle
To find the area of a right-angled triangle, we use the formula:
right-angled triangle, we take the two sides having the right angle, one as the base and one as height.
Example: Calculate area of a triangle of the Figure.
Data: base = 3 cm and height = 4 cm
Formula: Area of triangle = 1/2 × 3 × 4= 6 cm²
Result: Area of a triangle of the Figure is 6 cm² .
Remark: If you take base as 4 cm and height as 3 cm then also the area of the triangle will remain the same.
Area of Equilateral Triangle
Equilateral Triangle: Equilateral Triangle is defined as a triangle having three equal sides.
To calculate the area of the Equilateral Triangle ABC,
We calculate the height (altitude), AD by making the median of the triangle.
In the given example, the Height (altitude), AD touches Base of the equilateral triangle at the midpoint of BC, Say point, D.
Here the equilateral triangle ABC has three equal sides, such as:
AB = BC = AC = 10 cm.
Since, midpoint of BC divides the triangle into two right angle triangles.
The height, AD, is calculated using Pythagoras theorem.
According to Pythagoras theorem, AB² = AD² + BD²
On substituting the values we get,
(10)² = AD² + (5)²
AD² = (10)² – (5)²
AD² = 100 – 25 = 75
AD = 5√3
Now we can find the area of the triangle using the formula:
Area of triangle = 1/2 × base × height
On substituting the values we get,
Area of triangle = 1/2 × 10 × 5√3
25√3 cm²
Area of Isosceles Triangle
In the isosceles triangle also we need to find the height of the triangle then calculate the area of the triangle.
Here,
Area of a Triangle — by Heron’s Formula
The formula of the area of a triangle given by herons is called Heron’s Formula.
Heron’s Formula:
where a, b and c are the sides of the triangle and s is the semiperimeter
Generally, this formula is used when the height of the triangle is not possible to find or you can say if the triangle is a scalene triangle.
Here the sides of triangle are
AB = 12 cm
BC = 14 cm
AC = 6 cm
Application of Heron’s Formula in Finding Areas of Quadrilaterals
If we know the sides and one diagonal of the quadrilateral then we can find its area by using the Heron’s formula.
Find the area of the quadrilateral if its sides and the diagonal are given as follows.
Given, the sides of the quadrilateral
AB = 9 cm
BC = 40 cm
DC = 28 cm
AD = 15 cm
Diagonal is AC = 41 cm
Here, ∆ABC is a right angle triangle, so its area will be
Area of Quadrilateral ABCD = Area of ∆ABC + Area of ∆ADC
= 180 cm² + 126 cm²
= 306 cm²

Hindi Version
Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments
Assess
November 5, 2023
INTRODUCTION TO EUCLID’S GEOMETRY | Study
Assess
Interact
Pre-Requisires
Test & Enrich
Pre – Requisite

English Version
Speed Notes
Notes For Quick Recap
Introduction to Euclid Geometry The necessity of geometry had been felt from ancient times in different parts of the world.
The practical problems faced by people of ancient civilization had developed this branch of mathematics.
Let us cite few examples.
With floods in the river, the demarcations of land owners on the river-side land were used to wipe out. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
In order to redraw the boundaries, the idea of area was introduced, the idea of area was introduced.
The volumes of granaries could be measured by using geometry.
The existence of Egyptian pyramids indicates the use of geometry from olden times.
In Vedic period, there was a manual of geometrical construction, known as Sulbasutra’s.
Different geometrical shapes were constructed as altars to perform various Vedic rites.
The word Geometry originates from the green word ‘Geo’ (earth) and metrein (to measure) Through Geometry was developed and applied from ancient time in various part the world, it was not presented in a systematic manner.
Later in 300 BC, the Egyptian mathematician Euclid, collected all the known work and arranged it in a systematic manner.
‘Elements’ is a classic treatise in geometry which was written by Euclid.
This was the most influential book. The ‘element’ was used as a text book for several years in western Europe.
The ‘elements’ started with 28 definitions, five postulates and five common notions and systematically built the rest of plane and solid geometry.
The geometrical approach given by Euclid is known as Euclid method.
The Euclid method consists of making a small set of assumptions and then proving many other proposition from these assumptions.
The assumptions, made were obvious universal truth. The two types of assumption, made were ‘axioms’ and ‘postulates’.
Euclid’s Definitions Euclid listed 23 definitions in book 1 of the ‘elements’.
We list a few of them: 1) 2) 3) 4) 5) 6) 7) A point is that which has no part A line is a breadth less length The ends of a line are points A straight line is a line which lies evenly with the points on itself. A surface is that which has length and breadth only.
The edges of a surface are lines A plane surface is surface which lies evenly with straight lines on its self. Starting with these definitions, Euclid assumed certain assumptions, known as axioms and postulates.
Euclid’s Axioms Axioms were assumptions which were used throughout mathematics and are not specifically linked to geometry.
Few of Euclid’s axioms are:
1) Things which are equal to the same thing are equal to one another.
2) It equals are added to equals; the wholes are equal.
3) 4) 5) 6) 7) If equals are subtracted from equals, the remainders are equal.
Things which coincide one another are equal to one another.
The whole is greater than the part Things which are double of the same thing are equal to one another.
Things which are half of the same things are equal to one another.
All these axioms refer to magnitude of same kind.
Axiom – 1 can be written as follows: If x = Z and y = Z, then x = y
Axiom – 2 explains the following: If x = y, then x + Z = y + Z According to axiom – 3, If x = y, then x – Z = y – Z Axiom – 4 justifies the principle of superposition that every thing equals itself.
Axiom – 5, gives us the concept of comparison. If x is a part of y, then there is a quantity Z such that x = y + Z or x > y Note that magnitudes of the same kind can be added, subtracted or compared.
Euclid’s Postulates Euclid used the term postulate for the assumptions that were specific to geometry. Euclid’s five postulates are as follows: Postulate 1: A straight line may be drawn from any one point to any other point. Same may be stated as axiom 5.1 Given two distinct points, there is a unique line that passes through them.
Postulate 2: A terminated line can be produced indefinitely. Postulate 3: A circle can be drawn with any centre and any radius. Postulate 4: All right angles are equal to one another. Postulate 5: If a straight line falling on two straight lines makes the interior angle on the same side of it taken together less than two right angles, then two straight lines, if produced indefinitely, meet on that side on which the sum of the angles is less than two right angles. Postulates 1 to Postulates 4 are very simple and obvious and therefore they are taken a ‘self evident truths’. Postulates 5 is complex and it needs to be discussed. Suppose the line XY falls on two lines AB and CD such that ∠1 + ∠2 < 180°, then the lines AB and CD will intersect at a point. In the given figure, they intersect on left side of PQ, if both are produced. Note: In mathematics the words axiom and postulate may be used interchangeably, though they have distinct meaning according to Euclid. System of Consistent Axioms A system of axioms is said to be consistent, if it is impossible to deduce a statement from these axioms, which contradicts any of the given axioms or proposition. Proposition or Theorem The statement or results which were proved by using Euclid’s axioms and postulates are called propositions or Theorems. Theorem: Two distinct lines cannot have more than one point in common. Proof: Given: AB and CD are two lines. To prove: They intersect at one point or they do not intersect. Proof: Suppose the lines AB and CD intersect at two points P and Q. This implies the line AB passes through the points P and Q. Also the line CD passes through the points P and Q. This implies there are two lines which pass through two distinct point P and Q. But we know that one and only one line can pass through two distinct points. This axiom contradicts out assumption that two distinct lines can have more than one point in common. The lines AB and CD cannot pass through two distinct point P and Q. Equivalent Versions of Euclid’s Fifth Postulate The two different version of fifth postulate a) For every line l and for every point P not lying on l, there exist a unique line m passing through P and parallel to l. b) Two distinct intersecting lines cannot be parallel to the same line.

Hindi Version
Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments
Assess
November 5, 2023
NUMBER SYSTEMS | Study
Assess
Interact
Pre – Requisites
Test & Enrich
Pre – Requisite

English Version
Speed Notes
Notes For Quick Coverage
Introduction to Natural Numbers
Non-negative counting numbers excluding zero are called Natural Numbers.
N = 1, 2, 3, 4, 5, ……….
Whole Numbers
All natural numbers including zero are called Whole Numbers.
W = 0, 1, 2, 3, 4, 5, ……………. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
Integers
All natural numbers, negative numbers and 0, together are called Integers.
Z = – 3, – 2, – 1, 0, 1, 2, 3, 4, …………..
Rational Numbers
The number ‘a’ is called Rational if it can be written in the form of r/s where ‘r’ and ‘s’ are integers and s ≠ 0,
Q = 2/3, 3/5, etc. all are rational numbers.
How to find a rational number between two given numbers?
To find the rational number between two given numbers ‘a’ and ‘b’.
Example:
Find 2 rational numbers between 4 and 5.
Solution:
To find the rational number between 4 and 5
To find another number we will follow the same process again.
Hence the two rational numbers between 4 and 5 are 9/2 and 17/4.
Remark: There could be unlimited rational numbers between any two rational numbers.
Irrational Numbers
The number ‘a’ which can’t be written in the form of p/q is called irrational. Here, p and q are integers and q ≠ 0. You can say that the numbers which are not rational are called Irrational Numbers.
Example – √7, √11 etc.
Real Numbers
All numbers including both rational and irrational numbers are called Real Numbers.
R = – 2, – (2/3), 0, 3 and √2
Real Numbers And Their Decimal Expansions
1. Rational Numbers
If the rational number is in the form of a/b, then we can get two situations by dividing a by b.
a. If the remainder becomes zero
While dividing if we get zero as the remainder after some steps then the decimal expansion of such a number is called terminating.
Example:
7/8 = 0.875
b. If the remainder does not become zero
While dividing if the decimal expansion continues and not becomes zero then it is called non-terminating or repeating expansion.
Example:
1/3 = 0.3333….
Hence, the decimal expansion of rational numbers could be terminating or non-terminating recurring and vice-versa.
2. Irrational Numbers
If we do the decimal expansion of an irrational number then it would be non –terminating non-recurring and vice-versa. i. e. the remainder does not become zero and also not repeated.
Example:
π = 3.141592653589793238……
Representing Real Numbers on the Number Line
To represent the real numbers on the number line, we use the process of successive magnification. We visualise the numbers through a magnifying glass on the number line.
Example:
Step 1: The number lies between 4 and 5, so we divide it into 10 equal parts. Now for the first decimal place, we will mark the number between 4.2 and 4.3.
Step 2: Now we will divide it into 10 equal parts again. The second decimal place will be between 4.26 and 4.27.
Step 3: Now we will again divide it into 10 equal parts. The third decimal place will be between 4.262 and 4.263.
Step 4: By doing the same process again we will mark the point at 4.2626.
Operations on Real Numbers
1. The sum, difference, product and quotient of two rational numbers will be rational.
Example:
2. If we add or subtract a rational number with an irrational number then the outcome will be irrational.
Example:
If 5 is a rational number. √7 is an irrational number. Then, 5 + √7 and 5 – √7 are irrational numbers.
3. If we multiply a non-zero rational number with an irrational number, the outcome will be irrational. If we divide a non-zero rational number with an irrational number, the outcome will also be irrational.
Example:
If 7 is a rational number and √5 is an irrational number then 7√7 and 7/√5 are irrational numbers.
4. The sum, difference, product and quotient of two irrational numbers could be rational or irrational.
Example:
Finding Roots of a Positive Real Number ‘x’ geometrically and mark it on the Number Line
To find √x geometrically
1. First, mark the distance x unit from point A on the line. This ensures that AB equals x unit.
2. From B mark a point C with the distance of 1 unit, so that BC = 1 unit.
3. Take the midpoint of AC and mark it as O. Then take OC as the radius and draw a semicircle.
4. From the point B draw a perpendicular BD which intersects the semicircle at point D.
The length of BD = √x.
To mark the position of √x on the number line, we will take AC as the number line. B will be zero. So C is point 1 on the number line.
Now we will take B as the centre and BD as the radius. We will draw the arc on the number line at point E.
Now E is √x on the number line.
Identities Related to Square Roots
If p and q are two positive real numbers
Examples:
1. Simplify
We will use the identity
2. Simplify
We will use the identity
Rationalising the Denominator
Rationalising the denominator means to convert the denominator containing a square root term into a rational number. This is done by finding the equivalent fraction of the given fraction.
For which we can use the identities of the real numbers.
Example:
Rationalise the denominator of 7/(7- √3).
Solution:
We will use the identityhere.
Laws of Exponents for Real Numbers
If we have a and b as the base and m and n as the exponents, then
1. a^m × aⁿ =a^m+n
2. (a^m)ⁿ = a^mn
4. a^mb^m = (ab)^m
5.a⁰ = 1
6. a¹ = a
7. 1/aⁿ = a^-n
Let a > 0 be a real number and n a positive integer.
Let a > 0 be a real number. Let m and n be integers. They have no common factors other than 1. Also, n > 0. Then,
Example:
Simplify the expression (2x³y⁴) (3xy⁵)².
Solution:
Here we will use the law of exponents
a^m × aⁿ =a^m+n and (a^m)ⁿ = a^mn
(2x³y⁴)(3xy⁵)²
(2x³y⁴)(3 ² x ² y¹⁰)
18. x³. x². y⁴. y¹⁰
18. x³⁺². y⁴⁺¹⁰
18x⁵y¹⁴
Here’s a simple outline for an eBook on Real Numbers:
Title: Understanding Real Numbers: A Comprehensive Guide
Table of Contents
Introduction to Real Numbers
What are Numbers?
Introduction to Real Numbers
Why Are Real Numbers Important?
Classification of Numbers
Natural Numbers
Whole Numbers
Integers
Rational Numbers
Irrational Numbers
Properties of Real Numbers
Closure Property
Commutative Property
Associative Property
Distributive Property
Identity and Inverse Elements
The Real Number Line
Concept of Number Line
Plotting Real Numbers on the Number Line
Understanding Density of Real Numbers
Rational and Irrational Numbers
Definition of Rational Numbers
Properties of Rational Numbers
Definition of Irrational Numbers
Examples of Irrational Numbers (like √2, π, e)
Proving √2 is Irrational
Decimals and Real Numbers
Finite and Infinite Decimals
Terminating and Non-Terminating Decimals
Relationship between Decimals and Fractions
Operations on Real Numbers
Addition and Subtraction
Multiplication and Division
Operations with Decimals
Operations with Irrational Numbers
Absolute Value and Real Numbers
Definition of Absolute Value
Geometric Representation on the Number Line
Properties of Absolute Value
The Concept of Infinity
Understanding Infinite Sets
Limits and Real Numbers
Approaching Infinity on the Number Line
Applications of Real Numbers
In Geometry (Pythagorean Theorem)
In Calculus (Limits, Derivatives, and Integrals)
In Daily Life (Measurements, Finance, etc.)
Advanced Topics on Real Numbers
Real Numbers in Algebra
Real Numbers and Functions
Real Numbers and Continuity
Conclusion
Summary of Key Concepts
Importance of Mastering Real Numbers
How Real Numbers Apply to Higher Mathematics
Chapter 1: Introduction to Real Numbers
What Are Numbers?
Numbers are abstract symbols used to represent quantities. Throughout history, different types of numbers have been developed to address various mathematical problems.
Introduction to Real Numbers
Real numbers are all the numbers that can be found on the number line. This includes rational numbers (such as 5, -3, and 0.75) and irrational numbers (such as √2 and π). Together, they form the building blocks of modern mathematics.
Real numbers are used to measure continuous quantities like distance, time, and weight. They are integral to the concepts of calculus, physics, engineering, and many other fields.
Why Are Real Numbers Important?
Real numbers play a critical role in mathematics. They allow us to describe the size of objects, calculate areas and volumes, and express very large or very small values. Without real numbers, much of modern science and technology would not exist.
Chapter 2: Classification of Numbers
Natural Numbers
The set of natural numbers consists of counting numbers, such as 1, 2, 3, and so on. These are the simplest type of numbers and do not include zero.
Whole Numbers
Whole numbers are like natural numbers but also include zero. Thus, the set is {0, 1, 2, 3,…}.
Integers
Integers expand on whole numbers by including negative numbers. The set of integers is {…, -3, -2, -1, 0, 1, 2, 3,…}.
Rational Numbers
Rational numbers are numbers that can be expressed as a fraction of two integers (a/b), where b ≠ 0. Examples of rational numbers include 1/2, -4, and 0.75.
Irrational Numbers
Irrational numbers cannot be expressed as a fraction of two integers. Examples include √2, π, and e. These numbers have non-repeating, non-terminating decimal expansions.
Chapter 3: Properties of Real Numbers
Closure Property
The set of real numbers is closed under addition, subtraction, multiplication, and division (except division by zero). This means that the result of any of these operations on two real numbers will always yield another real number.
Commutative Property
For any two real numbers a and b:
Addition: a + b = b + a
Multiplication: a × b = b × a
Associative Property
For any three real numbers a, b, and c:
Addition: (a + b) + c = a + (b + c)
Multiplication: (a × b) × c = a × (b × c)
Distributive Property
The distributive property connects addition and multiplication:
a × (b + c) = (a × b) + (a × c)
Hindi Version
Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments
Assess
November 5, 2023
LINEAR EQUATIONS IN TWO VARIABLES | Study
Assess
Interact
Pre-Requisires
Test & Enrich
Pre – Requisite

English Version
Linear Equations in Two Variables | Speed Notes
Notes For Quick Recap
Linear Equations
The equation of a straight line is the linear equation. It could be in one variable or two variables.
Linear Equation in One Variable
The equation with one variable in it is known as a Linear Equation in One Variable. (Scroll down to continue …)
Study Tools
Audio, Visual & Digital Content
The general form for Linear Equation in One Variable is px + q = s, where p, q and s are real numbers and p ≠ 0.
Example:
x + 5 = 10
y – 3 = 19
These are called Linear Equations in One Variable because the highest degree of the variable is one.
Graph of the Linear Equation in One Variable
We can mark the point of the linear equation in one variable on the number line.
x = 2 can be marked on the number line as follows –
Graph of the Linear Equation in One Variable
Linear Equation in Two Variables
An equation with two variables is known as a Linear Equation in Two Variables. The general form of the linear equation in two variables is
ax + by + c = 0
where a and b are coefficients and c is the constant. a ≠ 0 and b ≠ 0.
Example
6x + 2y + 5 = 0, etc.
Slope Intercept form
Generally, the linear equation in two variables is written in the slope-intercept form as this is the easiest way to find the slope of the straight line while drawing the graph of it.
The slope-intercept form is y = mx+c
Where m represents the slope of the line.
and c tells the point of intersection of the line with the y-axis.
Remark: If b = 0 i.e. if the equation is y = mx then the line will pass through the origin as the y-intercept is zero.
Solution of a Linear Equation
There is only one solution in the linear equation in one variable but there are infinitely many solutions in the linear equation in two variables.
As there are two variables, the solution will be in the form of an ordered pair, i.e. (x, y).
The pair which satisfies the equation is the solution to that particular equation.
Example:
Find the solution for the equation 2x + y = 7.
Solution:
To calculate the solution of the given equation we will take x = 0
2(0) + y = 7
y = 7
Hence, one solution is (0, 7).
To find another solution we will take y = 0
2x + 0 = 7
x = 3.5
So another solution is (3.5, 0).
Graph of a Linear Equation in Two Variables
To draw the graph of a linear equation in two variables, we need to draw a table to write the solutions of the given equation, and then plot them on the Cartesian plane.
By joining these coordinates, we get the line of that equation.
The coordinates which satisfy the given Equation lie on the line of the equation.
Every point (x, y) on the line is the solution x = a, y = b of the given Equation.
Any point, which does not lie on the line AB, is not a solution of Equation.
Example:
Draw the graph of the equation 3x + 4y = 12.
Solution:
To draw the graph of the equation 3x + 4y = 12, we need to find the solutions of the equation.
Let x = 0
3(0) + 4y = 12
y = 3
Let y = 0
3x + 4(0) = 12
x = 4
Now draw a table to write the solutions.
x 0 4
y 3 0
Now we can draw the graph easily by plotting these points on the Cartesian plane.
Linear Equation in Two Variables
Equations of Lines Parallel to the x-axis and y-axis
When we draw the graph of the linear equation in one variable then it will be a point on the number line.
x – 5 = 0
x = 5
This shows that it has only one solution i.e. x = 5, so it can be plotted on the number line.
But if we treat this equation as the linear equation in two variables then it will have infinitely many solutions and the graph will be a straight line.
x – 5 = 0 or x + (0) y – 5 = 0
This shows that this is the linear equation in two variables where the value of y is always zero. So the line will not touch the y-axis at any point.
x = 5, x = number, then the graph will be the vertical line parallel to the y-axis.
All the points on the line will be the solution of the given equation.
Equations of Lines Parallel to the x-axis and y-axis
Similarly if y = – 3, y = number then the graph will be the horizontal line parallel to the x-axis.

Hindi Version
Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments
Assess
November 5, 2023
COORDINATE GEOMETRY | Study
Assess
Interact
Pre-Requisires
Test & Enrich
Pre – Requisite

English Version
Coordinate Geometry: Cartesian Plane | Speed Notes
Notes For Quick Recap
Cartesian System
A plane formed by two number lines, one horizontal
and the other vertical, such that they intersect each
other at their zeroes, and then they form a Cartesian
Plane.
Scroll Down To Continue …
Study Tools
Audio, Visual & Digital Content
● The horizontal line is known as the x-axis and the vertical line is known
as the y-axis.
● The point where these two lines intersect each other is called the origin.
It is represented as ‘O’.
● OX and OY are the positive directions as the positive numbers lie in the
right and upward direction.
● Similarly, the left and the downward directions are the negative directions
as all the negative numbers lie there.
Quadrants of the Cartesian Plane The Cartesian plane is divided into four quadrants namely Quadrant I, Quadrant II, Quadrant III, and Quadrant IV anticlockwise from OX.
Coordinates of a Point To write the coordinates of a point we need to follow the following rules. ● Thex – coordinate of a point is marked by drawing perpendicular from the y-axis measured a length of the x-axis .It is also called the Abscissa.
They – coordinate of a point is marked by drawing a perpendicular from the x-axis measured a length of the y-axis .It is also called the Ordinate. ● While writing the coordinates of a point in the coordinate plane, the x – coordinate comes first, and then the y – coordinate. We write the coordinates in brackets. In figure, OB = CA = x coordinate (Abscissa), and CO = AB = y coordinate (Ordinate). We write the coordinate as (x, y).
Remarks:
As the origin, O has zero distance from the x-axis and the y-axis so its abscissa and ordinate are zero. Hence the coordinate of the origin is (0, 0). The relationship between the signs of the coordinates of a point and the quadrant of a point in which it lies.
Plotting a Point in the Plane if its Coordinates are Given
Steps to plot the point (2, 3) on the Cartesian plane:
● First of all, we need to draw the Cartesian plane by drawing the coordinate axes with 1 unit = 1 cm.
● To mark the x coordinates, starting from 0 moves towards the positive x-axis and counts to 2.
● To mark the y coordinate, starting from 2 moves upwards in the positive direction and counts to 3.
● Now this point is the coordinate (2, 3). Likewise, we can plot all the other points, like (-3, 1) and (-1.5,-2.5) in the figure.
Question: Are the coordinates (x, y) = (y, x)? Let x = (-4) and y = (-2) So (x, y) = (- 4, – 2) (y, x) = (- 2, – 4)
Let’s mark these coordinates on the Cartesian plane. You can see that the positions of both the points are different in the Cartesian plane. So, If x ≠ y, then (x, y) ≠ (y, x), and (x, y) = (y, x), if x = y.
Example: Plot the points (6, 4), (- 6,- 4), (- 6, 4) and (6,- 4) on the Cartesian plane.
Solution: Since both numbers 6, 4 are positive the point (6, 4) lies in the first quadrant. For x coordinate, we will move towards the right and count to 6. Then from that point go upward and count to 4. Mark that point as the coordinate (6, 4). Similarly, we can plot all the other three points.

Hindi Version
Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
Assessments
Personalised Assessments
Assess
November 5, 2023

POLYNOMIALS | Study

Pre-Requisires

Test & Enrich

English Version

Speed Notes

Notes For Quick Recap

Study Tools

Audio, Visual & Digital Content

Revision Notes on Polynomials

Polynomial

A polynomial is an algebraic expression that includes constants, variables, and exponents. It is the expression in which the variables have only positive integral powers.

Example

1. 4x³ + 3x² + x +3 is a polynomial in variable x.

2. 4x² + 3x^-1 – 4 is not a polynomial as it has negative power.

3. 3x^3/2+ 2x – 3 is not a polynomial. Polynomials

Polynomials are denoted by p (x), q (x), etc.
In the above polynomials, 2x², 3y, and 2 are the terms of the polynomial.
2 and 3 are the coefficients of x² and y, respectively.
x and y are the variables.
2 is the constant term, which has no variable.

Polynomials in One Variable

If there is only one variable in the expression, then this is called the polynomial in one variable.

Example

x³ + x – 4 is polynomial in variable x and is denoted by p(x).
r² + 2 is polynomial in variable r and is denoted by p(r).

Types of polynomials on the basis of the number of terms

Types of polynomials on the basis of the number of degrees

The highest value of the power of the variable in the polynomial is the degree of the polynomial.

Zeros of a Polynomial

If p(x) is a polynomial, then the number ‘a’ will be the zero of the polynomial with p(a) = 0. We can find the zero of the polynomial by equating it to zero.

Example: 1

The given polynomial is p(x) = x – 4

To find the zero of the polynomial, we will equate it to zero.

x – 4 = 0

x = 4

p(4) = x – 4 = 4 – 4 = 0

This shows that if we place 4 in place of x, we get the value of the polynomial as zero. So 4 is the zero of this polynomial. And also, we are getting the value 4 by equating the polynomial with 0.

So 4 is the zero of the polynomial or the root of the polynomial.

The root of the polynomial is basically the x-intercept of the polynomial.

If the polynomial has one root, it will intersect the x-axis at one point only, and if it has two roots, it will intersect at two points, and so on.

Example: 2

Find p (1) for the polynomial p (t) = t² – t + 1

p (1) = (1)² – 1 + 1

= 1 – 1 + 1

= 1

Remainder Theorem

We know the property of division which follows in the basic division, i.e.

Dividend = (Divisor × Quotient) + Remainder

This follows the division of polynomials.

If p(x) and g(x) are two polynomials in which the degrees of p(x) ≥ degree of g(x) and g(x) ≠ 0 are given, then we can get the q(x) and r(x) so that:

P(x) = g(x) q(x) + r(x),

where r(x) = 0 or degree of r(x) < degree of g(x).

It says that p(x) divided by g(x), gives q(x) as a quotient and r(x) as a remainder.

Let’s understand it with an example

Division of a Polynomial with a Monomial

We can see that ‘x’ is common in the above polynomial, so we can write it as

Hence, 3x²+ x + 1 and x the factors of 3x³ + x² + x.

Steps of the Division of a Polynomial with a Non –Zero Polynomial

Divide x² – 3x -10 by 2 + x

Step 1: Write the dividend and divisor in descending order, i.e., in the standard form. x² – 3x -10 and x + 2

Divide the first term of the dividend with the first term of the divisor.

x²/x = x this will be the first term of the quotient.

Step 2: Now multiply the divisor by this term of the quotient and subtract it from the dividend.

Step 3: Now the remainder is our new dividend, so we will repeat the process again by dividing the dividend by the divisor.

Step 4: – (5x/x) = – 5

Step 5:

The remainder is zero.

Hence x² – 3x – 10 = (x + 2)(x – 5) + 0

Dividend = (Divisor × Quotient) + Remainder

The Remainder Theorem says that if p(x) is any polynomial of degree greater than or equal to one and let ‘t’ be any real number and p (x) is divided by the linear polynomial x – t, then the remainder is p(t).

As we know,

P(x) = g(x) q(x) + r(x)

If p(x) is divided by (x-t) then

If x = t

P (t) = (t – t). q (t) + r = 0

To find the remainder or to check the multiple of the polynomial, we can use the remainder theorem.

Example:

What is the remainder if a⁴ + a³ – 2a² + a + 1 is divided by a – 1.

Solution:

P(x) = a⁴ + a³ – 2a² + a + 1

To find the zero of (a – 1), we need to equate it to zero.

a -1 = 0

a = 1

p (1) = (1)⁴ + (1)³ – 2(1)² + (1) + 1

= 1 + 1 – 2 + 1 + 1

= 2

So by using the remainder theorem, we can easily find the remainder after the division of the polynomial.

Factor Theorem

The factor theorem says that if p(y) is a polynomial with degree n≥1 and t is a real number, then

(y – t) is a factor of p(y), if p(t) = 0, and
P (t) = 0 if (y – t) is a factor of p (y).

Example: 1

Check whether g(x) = x – 3 is the factor of p(x) = x³– 4x²+ x + 6 using the factor theorem.

Solution:

According to the factor theorem, if x – 3 is the factor of p(x), then p(3) = 0, as the root of x – 3 is 3.

P (3) = (3)³– 4(3)² + (3) + 6

= 27 – 36 + 3 + 6 = 0

Hence, g (x) is the factor of p (x).

Example: 2

Find the value of k, if x – 1 is a factor of p(x) = kx²– √2x + 1

Solution:

As x -1 is the factor, p(1) = 0

Factorization of Polynomials

Factorization can be done by three methods

1. By taking out the common factor

If we have to factorise x² –x then we can do it by taking x common.

x(x – 1) so that x and x-1 are the factors of x² – x.

2. By grouping

ab + bc + ax + cx = (ab + bc) + (ax + cx)

= b(a + c) + x(a + c)

= (a + c)(b + x)

3. By splitting the middle term

x² + bx + c = x² + (p + q) + pq

= (x + p)(x + q)

This shows that we have to split the middle term in such a way that the sum of the two terms is equal to ‘b’ and the product is equal to ‘c’.

Example: 1

Factorize 6x² + 17x + 5 by splitting the middle term.

Solution:

If we can find two numbers p and q such that p + q = 17 and pq = 6 × 5 = 30, then we can get the factors.

Some of the factors of 30 are 1 and 30, 2 and 15, 3 and 10, 5 and 6, out of which 2 and 15 is the pair which gives p + q = 17.

6x² + 17x + 5 =6 x² + (2 + 15) x + 5

= 6 x² + 2x + 15x + 5

= 2 x (3x + 1) + 5(3x + 1)

= (3x + 1) (2x + 5)

Algebraic Identities

1. (x + y)²= x²+ 2xy + y²

2. (x – y)² = x²– 2xy + y²

3. (x + y) (x – y) = x² – y²

4. (x + a) (x + b) = x² + (a + b)x + ab

5. (x + y + z)²= x²+ y²+ z²+ 2xy + 2yz + 2zx

6. (x + y)³ = x³ + y³ + 3xy(x + y) = x³+ y³ + 3x²y + 3xy²

7. (x – y)³ = x³– y³ – 3xy(x – y) = x³– y³ – 3x²y + 3xy²

8. x³+ y³ = (x + y)(x² – xy + y²)

9. x³ – y³= (x – y)(x² + xy + y²)

10. x³+ y³ + z³– 3xyz = (x + y + z)(x² + y²+ z² – xy – yz – zx) x³ + y³ + z³ = 3xyz if x + y + z = 0

Example: 2

Factorize 8x³ + 27y³ + 36x²y + 54xy²

Solution:

The given expression can be written as

= (2x)³+ (3y)³ + 3(4x²) (3y) + 3(2x) (9y²)

= (2x)³ + (3y)³ + 3(2x)²(3y) + 3(2x)(3y)²

= (2x + 3y)³ (Using Identity VI)

= (2x + 3y) (2x + 3y) (2x + 3y) are the factors.

Example: 3

Factorize 4x² + y² + z² – 4xy – 2yz + 4xz.

Solution:

4x² + y² + z² – 4xy – 2yz + 4xz = (2x)²+ (–y)² + (z)² + 2(2x) (-y)+ 2(–y)(z) + 2(2x)(z)

= [2x + (- y) + z]² (Using Identity V)

= (2x – y + z)² = (2x – y + z) (2x – y + z)

Hindi Version

Dig Deep

Topic Level Resources

Sub – Topics

Select A Topic

Topic:

Chapters Index

Select Another Chapter

Assessments

Personalised Assessments