At The Right Mentor, we understand the importance of quality study material in shaping a student’s academic journey. That’s why we offer a comprehensive range of study material for Mathematics and Science for classes 6 to 10.
Chapter Summaries (Speed Notes)
Our study material includes concise and well-structured chapter summaries, also known as Speed Notes. These summaries provide a quick overview of the key concepts, formulas, and important points covered in each chapter. With Speed Notes, students can easily revise and reinforce their understanding of the subject matter.
Keywords
Our study material also includes a list of keywords for each chapter. These keywords help students in understanding the core concepts and facilitate better retention of information. By focusing on these keywords, students can grasp the fundamental ideas and build a strong foundation in Mathematics and Science.
Soft Copies of Textbooks
To make learning more accessible, we provide soft copies of the textbooks prescribed by various education boards such as CBSE, ICSE, Telangana State Board, and Andhra Pradesh State Board. These PDF versions of the textbooks can be easily downloaded and accessed on any device, allowing students to study anytime, anywhere.
Textbook Solutions
In addition to the textbooks, we offer comprehensive solutions for the exercises and problems present in the textbooks. Our experts have carefully crafted these solutions to provide step-by-step explanations and help students understand the concepts effectively. These solutions serve as a valuable resource for self-study and exam preparation.
NCERT Exemplar Solutions
We also provide NCERT Exemplar Solutions, which are specifically designed to enhance problem-solving skills. These solutions cover a wide range of challenging problems and offer detailed explanations, enabling students to develop a deeper understanding of the subject.
With our study material, including Speed Notes, chapter videos, NCERT Exemplar Solutions, NCERT Solutions, and PDFs of NCERT textbooks, students can strengthen their knowledge, improve their performance, and excel in their Mathematics and Science studies.
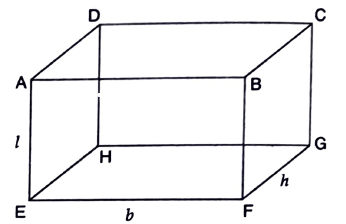
A cuboid is a three-dimensional geometric shape that resembles a rectangular box or a rectangular prism. A cuboid has 3 Pairs of opposite, congruent and parallel rectangular faces, 12 edges, and 8 vertices.
Note 1: All squares are rectangles.
Note 2: Cuboid may have one, or Three equal pairs of squares. (Square is a special type of Rectangle.
Note 3: If All three pairs of faces of a cuboid are squares then it it becomes a Cube.
Note 4: A cube is a special case of cuboid.
Parts And Their Alignment Of A Cuboid
Faces
The flat surfaces of a cuboid are known as its faces.
A cuboid has six faces, and each face is a rectangle.
These faces are arranged such that three pairs of opposite faces are parallel to each other.
The adjacent faces are perpendicular to each other (i.e., the angle between any two touching faces of a cube is right angle, 90°.
Note 1: All squares are rectangles.
Note 2: Rectangle may have one or two pairs of squares.
Note 3: If All three pairs of faces of a rectangle are squares then it it becomes a Cube.
Note 4: A cube is a special case of cuboid.
Edges
An edge is a line segment where the two surfaces of a cuboid meet.
There are 12 edges in a cuboid, where three edges meet at each vertex.
All edges form right angles with the adjacent edges and faces.
Vertices
A vertex is a point where the three edges meet. Vertices is the plural of vertex.
Cuboid has eight vertices.
Diagonals
Diagonal of a cuboid is a line segment that joins two opposite vertices.
The cuboid has four space diagonals.
Length of the diagonal of cuboid = √(length2 + breadth2 + height2) units.
Symmetry
Cuboids exhibit high symmetry.
They have rotational symmetry of order 4, meaning that you can rotate them by 90 degrees about their centre and they will look the same.
Features of a Cuboid
It is a three-dimensional, Rectangular figure.
It has 6 faces, 12 edges, and 8 vertices.
All 6 faces are rectangles.
Each vertex meets three faces and three edges.
The edges run parallel to those parallel to it.
All angles of a cuboid are right angles.
Mensuration of Cuboid
Surface Area of a Cuboid
The total surface area of a cuboid is defined as the area of its surface (Appearing face).
The Lateral Surface Area of a Cube.
Imagine yourself sitting in a cuboid shaped room. You can then see the four walls around you. This denotes the lateral surface area of that room.
That is, the lateral surface area of a cuboid shaped room is the area of its four walls, excluding the ceiling and the floor.
The lateral surface area of the cuboid is the sum of areas of its square faces, excluding the area of the top and the bottom face.
So the lateral surface area of a cube = sum of areas of 4 faces = (Length ✕ Height) + (Length ✕ Height) + (Length ✕ Height) + (Breadth ✕ Height) + (Breadth✕ Height)
Derivation of Total Surface Area of a Cuboid
Since the total surface area of a cuboid (TSA) is the area of its surface.
Total surface area of a cuboid = Lateral Surface Area + Area Of Bottom Surface + Area Of Top Surface
Total surface area of a cuboid = Area Of Front Surface + Area Of Back Surface + Area Of Left Srface + Area Of Right Surface + Area Of Bottom Surface + Area Of Top Surface
Total surface area of a cuboid = Lateral Surface Area 2[Area Of Bottom Surface]
Since Area Of Top Surface = + Area Of Bottom Surface We get, Total surface area of a cuboid = Lateral Surface Area + 2[Area Of Top Surface]
The volume of a three-dimensional object can be defined as the space required for it.
Similarly, Volume of a cuboid is defined as the space required for the cuboid or the Space occupied by the cuboid.
The volume of a cuboid can be calculated using the formula, V = lbh, where,
l = length, b = breadth or width, h = height
This formula shows that the volume of a cuboid is directly proportional to its length, breadth and height.
The volume is calculated by multiplying the object’s length, breadth, and height.
Hence the volume of the cube = lbh = lenth ✕ breadth ✕ height
Cuboids in Our Daily Life
Cuboids are commonly used in everyday objects, such as boxes, books, and building blocks.
They are used in architectural and engineering designs for modeling rooms, buildings, and structures.
In mathematics and geometry, cuboids serve as fundamental examples for teaching and understanding concepts related to three-dimensional shapes.
Similar Shapes:
A cube is a special type of cuboid where all sides are equal in length, making it a regular hexahedron.
Real-world Examples:
A shoebox is an example of a cuboid.
Most refrigerators, ovens, and TV screens have cuboidal shapes.
Buildings and houses often have cuboidal rooms.
Fun Fact:
Cuboids are among the simplest and most familiar three-dimensional shapes, making them a fundamental concept in geometry.
Remember that these notes provide an overview of cuboids, and there are more advanced topics and applications related to this shape in various fields of study.
What is a cube?
A cube is a three-dimensional regular polyhedron characterised by its 6 Identical (Congruent) Squares in which 3 Pairs of them parallel.
Parts And Their Alignment Of In A Cube
Faces
The flat surfaces of a cube are known as its faces.
A cube has six faces, and each face is a perfect square. These faces are arranged such that three pairs of faces are parallel to each other.
The adjacent faces are perpendicular to each other (the angle between any two touching faces of a cube is right angle, 90°.
All the edges have the same length.
A cube also has 8 vertices and 12 edges.
Edges
An edge is a line segment where the two surfaces of a cube meet.
There are twelve edges in a cube, where three edges meet at each vertex.
All edges have equal length and form right angles with the adjacent edges and faces.
Vertices
A vertex is a point where the three edges meet. Vertices is the plural of vertex.
Cube has eight vertices.
Diagonals
The cube has four space diagonals that connect opposite vertices, each of which has a length of √3 times the length of an edge.
Symmetry
Cubes exhibit high symmetry.
They have rotational symmetry of order 4, meaning that you can rotate them by 90 degrees about their centre and they will look the same.
Features of a Cube
It is a three-dimensional, square-shaped figure.
It has 6 faces, 12 edges, and 8 vertices.
All 6 faces are squares with equal area.
All sides have the same length.
Each vertex meets three faces and three edges.
The edges run parallel to those parallel to it.
All angles of a cube are right angles.
Mensuration of Cube
Surface Area of a Cube
The total surface area of a cube is defined as the area of its outer surface.
Derivation of Total Surface Area of a Cube
Since the total surface area of a cube is the area of its outer surface.
total surface area of a cube = 6 ✕ area of one face.
We know that the cube has six square faces and each of the square faces is of the same size, the total surface area of a cube = 6 ✕ area of one face.
Let the length of each edge is “s”.
Area of one square face = length of edge ✕ length of edge
Area of one square face == s ✕ s = s²
Therefore, the total surface area of the cube = 6s²
The total surface area of the cube will be equal to the sum of all six faces of the cube.
The Lateral Surface Area of a Cube.
Imagine yourself sitting in a cube shaped room. You can then see the four walls around you. This denotes the lateral surface area of that room.
That is, the lateral surface area of a cube shaped room is the area of its four walls, excluding the ceiling and the floor.
The lateral surface area of the cube is the sum of areas of its square faces, excluding the area of the top and the bottom face.
So the lateral surface area of a cube = sum of areas of 4 faces = 4a²
The Volume of a Cube
Volume
The volume of a three-dimensional object can be defined as the space required for it.
Similarly, Volume of a cube is defined as the space required for the cube or the Space occupied by the cube.
The volume of a cube can be calculated using the formula V = s3, where “s” represents the length of one side of the cube.
This formula shows that the volume of a cube is directly proportional to the cube of its side length.
The volume is calculated by multiplying the object’s length, breadth, and height. In the case of a cube shape, the length, width, and height are all of the same length. Let us refer to it as “s”.
Hence the volume of the cube is s ✕ s ✕ s = s³
Cubes in Our Daily Life
We encounter many cubes in our daily life such as Ice cubes, sugar cubes, dice and the building blocks used in games.
Cubes play a fundamental role in the study of geometry and serve as a basis for understanding three-dimensional space and concepts such as volume and surface area.
Also, Cubes have many applications in mathematics, engineering, architecture and art etc.
Study Tools
Audio, Visual & Digital Content
Cylinder is an important topic in Mathematics. It is a three-dimensional solid shape that has two parallel circular bases connected by a curved surface.
In this post, we will explore the properties of a cylinder and how to calculate its volume and surface area.
Let’s start with the basic definition of a right circular cylinder.
A cylinder is a solid shape that has two parallel circular bases of equal size and shape.
The curved surface that connects the two bases is called the lateral surface.
The axis of the cylinder is a line passing through the center of both bases.
Types Of Cylinders
(i). Solid Cylinder
(ii). Hollow Cylinder
Area Of A Solid Cylinder
Total Surface Area of Right Circular Cylinder = Curved Surface Area + Cicular Base Area + Circular Top Surface Area.
Since the Cicular Base Area And The Circular Top Surface Area of a cylinder are equal
Total Surface Area of Right Circular Cylinder = Curved Surface Area + 2(Cicular Top Surface Area)
Or
Total Surface Area of Right Circular Cylinder = Curved Surface Area + 2(Cicular Base Surface Area)
Therefore the formula to calculate the surface area of a cylinder is expressed as the following:
$$SA = 2\pi r h + 2\pi r^2$$
where SA is the surface area, r is the radius of the base, and h is the height of the cylinder.
Here, Curved Surface Area, $$CSA = 2\pi r h$$ and
Cicular Base Area = Top Surface Area = $$\pi r^2$$
Volume Of A Solid Cylinder
The formula to calculate the volume of a cylinder is given by:
$$V = \pi r^2 h$$
where V is the volume, r is the radius of the base, and h is the height of the cylinder.
Now let’s take an example to understand how to use these formulas. Suppose we have a cylinder with a radius of 4 cm and a height of 10 cm. To calculate its volume, we can use the formula:
$$V = \pi (4)^2 (10) = 160\pi$$
Therefore, the volume of the cylinder is 160π cubic cm.
To calculate its surface area, we can use the formula:
$$SA = 2\pi (4) (10) + 2\pi (4)^2 = 120\pi$$
Therefore, the surface area of the cylinder is 120π square cm.
In conclusion, understanding the properties of a cylinder and how to calculate its volume and surface area is important in CBSE Class 10 Mathematics. By using the formulas mentioned above, you can easily solve problems related to cylinders.
Hollow Cylinder
A hollow cylinder is a three-dimensional object with a circular base and a cylindrical shape. It is also known as a cylindrical shell. The cylinder has two circular faces and a curved surface. The thickness of the cylinder is uniform and it is hollow from inside.
Volume Of A Hollow Cylinder
The volume of a hollow cylinder can be calculated using the formula V = πh(R2-r2), where h is the height of the cylinder, R is the radius of the outer circle, and r is the radius of the inner circle.
Surface Area Of A Hollow Cylinder
The surface area of a hollow cylinder can be calculated using the formula A = 2πh(R+r), where h is the height of the cylinder, R is the radius of the outer circle, and r is the radius of the inner circle.
Hollow cylinders are used in various applications such as pipes, drums, and containers. They are also used in engineering structures such as bridges and towers.
In conclusion, a hollow cylinder is a useful shape in various fields and can be easily calculated using mathematical equations.
Cone
A cone is a three-dimensional geometric shape that has a circular base and a single vertex. It can be visualized as a pyramid with a circular base. In this note, we will cover the basic concepts and formulas related to cones.
Surface Area of a Cone
The surface area of a cone is the sum of the areas of its base and lateral surface. The formula to calculate the surface area of a cone is:
$$A = \pi r (r + l)$$
Where:
( A ) is the surface area of the cone
$$\pi = 3.14159$$
( r ) is the radius of the base of the cone
( l ) is the slant height of the cone
Volume of a Cone
The volume of a cone is the amount of space enclosed by it. The formula to calculate the volume of a cone is:
$$V = \frac{1}{3} \pi r^2 h$$
Where:
( V ) is the volume of the cone
( \pi ) is a mathematical constant approximately equal to 3.14159
( r ) is the radius of the base of the cone
( h ) is the height of the cone
Example Equations
Here are a few example equations related to cones:
Equation for calculating the slant height of a cone: $$l = \sqrt{r^2 + h^2}$$
Equation for calculating the radius of a cone given its slant height and height: $$r = \sqrt{l^2 – h^2}$$
Equation for calculating the height of a cone given its volume and radius: $$h = \frac{3V}{\pi r^2}$$
Sphere
Solid Sphere
Introduction – A solid sphere is a three-dimensional geometric figure in which all points inside the sphere are at the same distance from its center. – It is a type of 3D shape known as a “sphere” with a uniform density throughout.
Characteristics – The solid sphere has a well-defined volume, surface area, and mass. – It is completely filled with matter.
Volume of Solid Sphere The formula to calculate the volume (\(V_s\)) of a solid sphere is given by: \[ V_s = \frac{4}{3} \pi r^3 \] Where: \(V_s\) = Volume of the solid sphere, \(\pi\) (\(\pi\)) ≈ 3.14159, \(r\) = Radius of the sphere.
Surface Area of Solid Sphere The formula to calculate the surface area (\(A_s\)) of a solid sphere is given by: \[ A_s = 4 \pi r^2 \]
Where: \(A_s\) = Surface area of the solid sphere, \(\pi\) (\(\pi\)) ≈ 3.14159, \(r\) = Radius of the sphere
Mass of Solid Sphere The mass (\(m_s\)) of a solid sphere can be calculated using the formula: \[ m_s = \text{Density} \times V_s \] Where: \(m_s\) = Mass of the solid sphere, \(\text{Density}\) = Density of the material making up the sphere (usually in \(kg/m^3\)), \(V_s\) = Volume of the solid sphere (calculated using the previous formula)
Hollow Sphere
Introduction – A hollow sphere is also a three-dimensional geometric figure, but unlike a solid sphere, it has an empty space inside. – It consists of an outer shell or surface with a certain thickness and an inner empty region.
Characteristics – The hollow sphere has a well-defined outer radius (\(R\)), inner radius (\(r\)), volume, surface area, and mass. – It is partially filled with matter, mainly in the form of the outer shell.
Volume of Hollow Sphere The formula to calculate the volume (\(V_h\)) of a hollow sphere is given by: \[ V_h = \frac{4}{3} \pi (R^3 – r^3) \] Where: – \(V_h\) = Volume of the hollow sphere, \(\pi\) (\(\pi\)) ≈ 3.14159, \(R\) = Outer radius of the sphere, \(r\) = Inner radius of the sphere
Surface Area of Hollow Sphere The formula to calculate the surface area (\(A_h\)) of a hollow sphere is given by: \[ A_h = 4 \pi (R^2 – r^2) \] Where: \(A_h\) = Surface area of the hollow sphere, \(\pi\) (\(\pi\)) ≈ 3.14159, \(R\) = Outer radius of the sphere, \(r\) = Inner radius of the sphere
Mass of Hollow Sphere The mass (\(m_h\)) of a hollow sphere can be calculated using the formula: \[ m_h = \text{Density} \times V_h \] Where: \(m_h\) = Mass of the hollow sphere, \(\text{Density}\) = Density of the material making up the sphere (usually in \(kg/m^3\)), \(V_h\) = Volume of the hollow sphere (calculated using the previous formula)
Hindi Version
Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
[display-posts category=”CBSE 10 – Mathematics – Study – Premium” posts_per_page=”25″]
A cuboid is a three-dimensional geometric shape that resembles a rectangular box or a rectangular prism. A cuboid has 3 Pairs of opposite, congruent and parallel rectangular faces, 12 edges, and 8 vertices.
Note 1: All squares are rectangles, But All rectangles need not to be squares.
Note 2: Cuboid may have one, or three equal pairs of squares. (Square is a special type of Rectangle.
Note 3: If All one pair of opposite faces of a cuboid are squares then it it becomes an Equilateral Cuboid.
Note 3: If All three pairs of faces of a cuboid are squares then it it becomes a Cube.
Note 4: A Equilateral Cuboid and Cube is a special types of cuboid.
The flat surfaces of a cuboid are known as its faces.
A cuboid has six faces, and each face is a rectangle.
These faces are arranged such that three pairs of opposite faces are parallel to each other.
The adjacent faces are perpendicular to each other (i.e., the angle between any two touching faces of a cube is right angle, 90°.
Note 1: All squares are rectangles.
Note 2: Rectangle may have one or two pairs of squares.
Note 3: If All three pairs of faces of a rectangle are squares then it it becomes a Cube.
Note 4: A cube is a special case of cuboid.
Edges
An edge is a line segment where the two surfaces of a cuboid meet.
There are 12 edges in a cuboid, where three edges meet at each vertex.
All edges form right angles with the adjacent edges and faces.
Vertices
A vertex is a point where the three edges meet. Vertices is the plural of vertex.
Cuboid has eight vertices.
Diagonals
Diagonal of a cuboid is a line segment that joins two opposite vertices.
The cuboid has four space diagonals.
Length of the diagonal of cuboid = √(length2 + breadth2 + height2) units.
Symmetry
Cuboids exhibit high symmetry.
They have rotational symmetry of order 4, meaning that you can rotate them by 90 degrees about their centre and they will look the same.
Features of a Cuboid
It is a three-dimensional, Rectangular figure.
It has 6 faces, 12 edges, and 8 vertices.
All 6 faces are rectangles.
Each vertex meets three faces and three edges.
The edges run parallel to those parallel to it.
All angles of a cuboid are right angles.
Mensuration of Cuboid
What Is Diagonal Of A Cuboid?
The diagonal of a cuboid is the line segment that connects two opposite vertices of the cuboid. In other words, it is the longest line that can be drawn inside the cuboid.
Clculating The Length Of Diagonal Of A Cuboid?
To calculate the length of the diagonal, we need to know the lengths of all three edges of the cuboid. Let’s call these edges l, w and h. The length of the diagonal is then given by the following formula:
d = √(l² + w² + h²)
For example, let’s say that we have a cuboid with edges that are all equal to two meters. In this case, the length of the diagonal would be:
d = √(l² + w² + h²)
Example: if l = 3 cm, b = 2cm, h = 1cm, Find is longest diagonal
The longest diagonal of a cuboid = d = √(l² + w² + h²)
On substituting the values we get
d = √(3² + 2² + 1²) = √(9 + 4 +1) = √14 cm
Therefore, the length of the diagonal is fourteen meters.
Surface Area of a Cuboid
The total surface area of a cuboid is defined as the area of its surface (Appearing face).
The Lateral Surface Area of a Cube.
Imagine yourself sitting in a cuboid shaped room. You can then see the four walls around you. This denotes the lateral surface area of that room.
That is, the lateral surface area of a cuboid shaped room is the area of its four walls, excluding the ceiling and the floor.
The lateral surface area of the cuboid is the sum of areas of its square faces, excluding the area of the top and the bottom face.
So the lateral surface area of a cube = sum of areas of 4 faces = (Length ✕ Height) + (Length ✕ Height) + (Length ✕ Height) + (Breadth ✕ Height) + (Breadth✕ Height)
Derivation of Total Surface Area of a Cuboid
Since the total surface area of a cuboid (TSA) is the area of its surface.
Total surface area of a cuboid = Lateral Surface Area + Area Of Bottom Surface + Area Of Top Surface
Total surface area of a cuboid = Area Of Front Surface + Area Of Back Surface + Area Of Left Srface + Area Of Right Surface + Area Of Bottom Surface + Area Of Top Surface
Total surface area of a cuboid = Lateral Surface Area 2[Area Of Bottom Surface]
Since Area Of Top Surface = + Area Of Bottom Surface We get, Total surface area of a cuboid = Lateral Surface Area + 2[Area Of Top Surface]
The volume of a three-dimensional object can be defined as the space required for it.
Similarly, Volume of a cuboid is defined as the space required for the cuboid or the Space occupied by the cuboid.
The volume of a cuboid can be calculated using the formula, V = lbh, where,
l = length, b = breadth or width, h = height
This formula shows that the volume of a cuboid is directly proportional to its length, breadth and height.
The volume is calculated by multiplying the object’s length, breadth, and height.
Hence the volume of the cube = lbh = lenth ✕ breadth ✕ height
Cuboids in Our Daily Life
Cuboids are commonly used in everyday objects, such as boxes, books, and building blocks.
They are used in architectural and engineering designs for modeling rooms, buildings, and structures.
In mathematics and geometry, cuboids serve as fundamental examples for teaching and understanding concepts related to three-dimensional shapes.
Similar Shapes:
A cube is a special type of cuboid where all sides are equal in length, making it a regular hexahedron.
Real-world Examples:
A shoebox is an example of a cuboid.
Most refrigerators, ovens, and TV screens have cuboidal shapes.
Buildings and houses often have cuboidal rooms.
Fun Fact:
Cuboids are among the simplest and most familiar three-dimensional shapes, making them a fundamental concept in geometry.
Remember that these notes provide an overview of cuboids, and there are more advanced topics and applications related to this shape in various fields of study.
What is a cube?
A cube is a three-dimensional regular polyhedron characterised by its 6 Identical (Congruent) Squares in which 3 Pairs of them parallel.
Parts And Their Alignment Of In A Cube
Faces
The flat surfaces of a cube are known as its faces.
A cube has six faces, and each face is a perfect square. These faces are arranged such that three pairs of faces are parallel to each other.
The adjacent faces are perpendicular to each other (the angle between any two touching faces of a cube is right angle, 90°.
All the edges have the same length.
A cube also has 8 vertices and 12 edges.
Edges
An edge is a line segment where the two surfaces of a cube meet.
There are twelve edges in a cube, where three edges meet at each vertex.
All edges have equal length and form right angles with the adjacent edges and faces.
Vertices
A vertex is a point where the three edges meet. Vertices is the plural of vertex.
Cube has eight vertices.
Diagonals
The cube has four space diagonals that connect opposite vertices, each of which has a length of √3 times the length of an edge.
Symmetry
Cubes exhibit high symmetry.
They have rotational symmetry of order 4, meaning that you can rotate them by 90 degrees about their centre and they will look the same.
Features of a Cube
It is a three-dimensional, square-shaped figure.
It has 6 faces, 12 edges, and 8 vertices.
All 6 faces are squares with equal area.
All sides have the same length.
Each vertex meets three faces and three edges.
The edges run parallel to those parallel to it.
All angles of a cube are right angles.
Mensuration of Cube
Surface Area of a Cube
The total surface area of a cube is defined as the area of its outer surface.
Derivation of Total Surface Area of a Cube
Since the total surface area of a cube is the area of its outer surface.
total surface area of a cube = 6 ✕ area of one face.
We know that the cube has six square faces and each of the square faces is of the same size, the total surface area of a cube = 6 ✕ area of one face.
Let the length of each edge is “s”.
Area of one square face = length of edge ✕ length of edge
Area of one square face == s ✕ s = s²
Therefore, the total surface area of the cube = 6s²
The total surface area of the cube will be equal to the sum of all six faces of the cube.
The Lateral Surface Area of a Cube.
Imagine yourself sitting in a cube shaped room. You can then see the four walls around you. This denotes the lateral surface area of that room.
That is, the lateral surface area of a cube shaped room is the area of its four walls, excluding the ceiling and the floor.
The lateral surface area of the cube is the sum of areas of its square faces, excluding the area of the top and the bottom face.
So the lateral surface area of a cube = sum of areas of 4 faces = 4a²
The Volume of a Cube
Volume
The volume of a three-dimensional object can be defined as the space required for it.
Similarly, Volume of a cube is defined as the space required for the cube or the Space occupied by the cube.
The volume of a cube can be calculated using the formula V = s3, where “s” represents the length of one side of the cube.
This formula shows that the volume of a cube is directly proportional to the cube of its side length.
The volume is calculated by multiplying the object’s length, breadth, and height. In the case of a cube shape, the length, width, and height are all of the same length. Let us refer to it as “s”.
Hence the volume of the cube is s ✕ s ✕ s = s³
Cubes in Our Daily Life
We encounter many cubes in our daily life such as Ice cubes, sugar cubes, dice and the building blocks used in games.
Cubes play a fundamental role in the study of geometry and serve as a basis for understanding three-dimensional space and concepts such as volume and surface area.
Also, Cubes have many applications in mathematics, engineering, architecture and art etc.
A cuboid is a three-dimensional geometric shape that resembles a rectangular box or a rectangular prism. A cuboid has 3 Pairs of opposite, congruent and parallel rectangular faces, 12 edges, and 8 vertices.
Note 1: All squares are rectangles.
Note 2: Cuboid may have one, or Three equal pairs of squares. (Square is a special type of Rectangle.
Note 3: If All three pairs of faces of a cuboid are squares then it it becomes a Cube.
Note 4: A cube is a special case of cuboid.
Parts And Their Alignment Of A Cuboid
Faces
The flat surfaces of a cuboid are known as its faces.
A cuboid has six faces, and each face is a rectangle.
These faces are arranged such that three pairs of opposite faces are parallel to each other.
The adjacent faces are perpendicular to each other (i.e., the angle between any two touching faces of a cube is right angle, 90°.
Note 1: All squares are rectangles.
Note 2: Rectangle may have one or two pairs of squares.
Note 3: If All three pairs of faces of a rectangle are squares then it it becomes a Cube.
Note 4: A cube is a special case of cuboid.
Edges
An edge is a line segment where the two surfaces of a cuboid meet.
There are 12 edges in a cuboid, where three edges meet at each vertex.
All edges form right angles with the adjacent edges and faces.
Vertices
A vertex is a point where the three edges meet. Vertices is the plural of vertex.
Cuboid has eight vertices.
Diagonals
Diagonal of a cuboid is a line segment that joins two opposite vertices.
The cuboid has four space diagonals.
Length of the diagonal of cuboid = √(length2 + breadth2 + height2) units.
Symmetry
Cuboids exhibit high symmetry.
They have rotational symmetry of order 4, meaning that you can rotate them by 90 degrees about their centre and they will look the same.
Features of a Cuboid
It is a three-dimensional, Rectangular figure.
It has 6 faces, 12 edges, and 8 vertices.
All 6 faces are rectangles.
Each vertex meets three faces and three edges.
The edges run parallel to those parallel to it.
All angles of a cuboid are right angles.
Mensuration of Cuboid
Surface Area of a Cuboid
The total surface area of a cuboid is defined as the area of its surface (Appearing face).
The Lateral Surface Area of a Cube.
Imagine yourself sitting in a cuboid shaped room. You can then see the four walls around you. This denotes the lateral surface area of that room.
That is, the lateral surface area of a cuboid shaped room is the area of its four walls, excluding the ceiling and the floor.
The lateral surface area of the cuboid is the sum of areas of its square faces, excluding the area of the top and the bottom face.
So the lateral surface area of a cube = sum of areas of 4 faces = (Length ✕ Height) + (Length ✕ Height) + (Length ✕ Height) + (Breadth ✕ Height) + (Breadth✕ Height)
Derivation of Total Surface Area of a Cuboid
Since the total surface area of a cuboid (TSA) is the area of its surface.
Total surface area of a cuboid = Lateral Surface Area + Area Of Bottom Surface + Area Of Top Surface
Total surface area of a cuboid = Area Of Front Surface + Area Of Back Surface + Area Of Left Srface + Area Of Right Surface + Area Of Bottom Surface + Area Of Top Surface
Total surface area of a cuboid = Lateral Surface Area 2[Area Of Bottom Surface]
Since Area Of Top Surface = + Area Of Bottom Surface We get, Total surface area of a cuboid = Lateral Surface Area + 2[Area Of Top Surface]
The volume of a three-dimensional object can be defined as the space required for it.
Similarly, Volume of a cuboid is defined as the space required for the cuboid or the Space occupied by the cuboid.
The volume of a cuboid can be calculated using the formula, V = lbh, where,
l = length, b = breadth or width, h = height
This formula shows that the volume of a cuboid is directly proportional to its length, breadth and height.
The volume is calculated by multiplying the object’s length, breadth, and height.
Hence the volume of the cube = lbh = lenth ✕ breadth ✕ height
Cuboids in Our Daily Life
Cuboids are commonly used in everyday objects, such as boxes, books, and building blocks.
They are used in architectural and engineering designs for modeling rooms, buildings, and structures.
In mathematics and geometry, cuboids serve as fundamental examples for teaching and understanding concepts related to three-dimensional shapes.
Similar Shapes:
A cube is a special type of cuboid where all sides are equal in length, making it a regular hexahedron.
Real-world Examples:
A shoebox is an example of a cuboid.
Most refrigerators, ovens, and TV screens have cuboidal shapes.
Buildings and houses often have cuboidal rooms.
Fun Fact:
Cuboids are among the simplest and most familiar three-dimensional shapes, making them a fundamental concept in geometry.
Remember that these notes provide an overview of cuboids, and there are more advanced topics and applications related to this shape in various fields of study.
What is a cube?
A cube is a three-dimensional regular polyhedron characterised by its 6 Identical (Congruent) Squares in which 3 Pairs of them parallel.
Parts And Their Alignment Of In A Cube
Faces
The flat surfaces of a cube are known as its faces.
A cube has six faces, and each face is a perfect square. These faces are arranged such that three pairs of faces are parallel to each other.
The adjacent faces are perpendicular to each other (the angle between any two touching faces of a cube is right angle, 90°.
All the edges have the same length.
A cube also has 8 vertices and 12 edges.
Edges
An edge is a line segment where the two surfaces of a cube meet.
There are twelve edges in a cube, where three edges meet at each vertex.
All edges have equal length and form right angles with the adjacent edges and faces.
Vertices
A vertex is a point where the three edges meet. Vertices is the plural of vertex.
Cube has eight vertices.
Diagonals
The cube has four space diagonals that connect opposite vertices, each of which has a length of √3 times the length of an edge.
Symmetry
Cubes exhibit high symmetry.
They have rotational symmetry of order 4, meaning that you can rotate them by 90 degrees about their centre and they will look the same.
Features of a Cube
It is a three-dimensional, square-shaped figure.
It has 6 faces, 12 edges, and 8 vertices.
All 6 faces are squares with equal area.
All sides have the same length.
Each vertex meets three faces and three edges.
The edges run parallel to those parallel to it.
All angles of a cube are right angles.
Mensuration of Cube
Surface Area of a Cube
The total surface area of a cube is defined as the area of its outer surface.
Derivation of Total Surface Area of a Cube
Since the total surface area of a cube is the area of its outer surface.
total surface area of a cube = 6 ✕ area of one face.
We know that the cube has six square faces and each of the square faces is of the same size, the total surface area of a cube = 6 ✕ area of one face.
Let the length of each edge is “s”.
Area of one square face = length of edge ✕ length of edge
Area of one square face == s ✕ s = s²
Therefore, the total surface area of the cube = 6s²
The total surface area of the cube will be equal to the sum of all six faces of the cube.
The Lateral Surface Area of a Cube.
Imagine yourself sitting in a cube shaped room. You can then see the four walls around you. This denotes the lateral surface area of that room.
That is, the lateral surface area of a cube shaped room is the area of its four walls, excluding the ceiling and the floor.
The lateral surface area of the cube is the sum of areas of its square faces, excluding the area of the top and the bottom face.
So the lateral surface area of a cube = sum of areas of 4 faces = 4a²
The Volume of a Cube
Volume
The volume of a three-dimensional object can be defined as the space required for it.
Similarly, Volume of a cube is defined as the space required for the cube or the Space occupied by the cube.
The volume of a cube can be calculated using the formula V = s3, where “s” represents the length of one side of the cube.
This formula shows that the volume of a cube is directly proportional to the cube of its side length.
The volume is calculated by multiplying the object’s length, breadth, and height. In the case of a cube shape, the length, width, and height are all of the same length. Let us refer to it as “s”.
Hence the volume of the cube is s ✕ s ✕ s = s³
Cubes in Our Daily Life
We encounter many cubes in our daily life such as Ice cubes, sugar cubes, dice and the building blocks used in games.
Cubes play a fundamental role in the study of geometry and serve as a basis for understanding three-dimensional space and concepts such as volume and surface area.
Also, Cubes have many applications in mathematics, engineering, architecture and art etc.
Study Tools
Audio, Visual & Digital Content
Cylinder is an important topic in Mathematics. It is a three-dimensional solid shape that has two parallel circular bases connected by a curved surface.
In this post, we will explore the properties of a cylinder and how to calculate its volume and surface area.
Let’s start with the basic definition of a right circular cylinder.
A cylinder is a solid shape that has two parallel circular bases of equal size and shape.
The curved surface that connects the two bases is called the lateral surface.
The axis of the cylinder is a line passing through the center of both bases.
Types Of Cylinders
(i). Solid Cylinder
(ii). Hollow Cylinder
Area Of A Solid Cylinder
Total Surface Area of Right Circular Cylinder = Curved Surface Area + Cicular Base Area + Circular Top Surface Area.
Since the Cicular Base Area And The Circular Top Surface Area of a cylinder are equal
Total Surface Area of Right Circular Cylinder = Curved Surface Area + 2(Cicular Top Surface Area)
Or
Total Surface Area of Right Circular Cylinder = Curved Surface Area + 2(Cicular Base Surface Area)
Therefore the formula to calculate the surface area of a cylinder is expressed as the following:
$$SA = 2\pi r h + 2\pi r^2$$
where SA is the surface area, r is the radius of the base, and h is the height of the cylinder.
Here, Curved Surface Area, $$CSA = 2\pi r h$$ and
Cicular Base Area = Top Surface Area = $$\pi r^2$$
Volume Of A Solid Cylinder
The formula to calculate the volume of a cylinder is given by:
$$V = \pi r^2 h$$
where V is the volume, r is the radius of the base, and h is the height of the cylinder.
Now let’s take an example to understand how to use these formulas. Suppose we have a cylinder with a radius of 4 cm and a height of 10 cm. To calculate its volume, we can use the formula:
$$V = \pi (4)^2 (10) = 160\pi$$
Therefore, the volume of the cylinder is 160π cubic cm.
To calculate its surface area, we can use the formula:
$$SA = 2\pi (4) (10) + 2\pi (4)^2 = 120\pi$$
Therefore, the surface area of the cylinder is 120π square cm.
In conclusion, understanding the properties of a cylinder and how to calculate its volume and surface area is important in CBSE Class 10 Mathematics. By using the formulas mentioned above, you can easily solve problems related to cylinders.
Hollow Cylinder
A hollow cylinder is a three-dimensional object with a circular base and a cylindrical shape. It is also known as a cylindrical shell. The cylinder has two circular faces and a curved surface. The thickness of the cylinder is uniform and it is hollow from inside.
Volume Of A Hollow Cylinder
The volume of a hollow cylinder can be calculated using the formula V = πh(R2-r2), where h is the height of the cylinder, R is the radius of the outer circle, and r is the radius of the inner circle.
Surface Area Of A Hollow Cylinder
The surface area of a hollow cylinder can be calculated using the formula A = 2πh(R+r), where h is the height of the cylinder, R is the radius of the outer circle, and r is the radius of the inner circle.
Hollow cylinders are used in various applications such as pipes, drums, and containers. They are also used in engineering structures such as bridges and towers.
In conclusion, a hollow cylinder is a useful shape in various fields and can be easily calculated using mathematical equations.
Cone
A cone is a three-dimensional geometric shape that has a circular base and a single vertex. It can be visualized as a pyramid with a circular base. In this note, we will cover the basic concepts and formulas related to cones.
Surface Area of a Cone
The surface area of a cone is the sum of the areas of its base and lateral surface. The formula to calculate the surface area of a cone is:
$$A = \pi r (r + l)$$
Where:
( A ) is the surface area of the cone
$$\pi = 3.14159$$
( r ) is the radius of the base of the cone
( l ) is the slant height of the cone
Volume of a Cone
The volume of a cone is the amount of space enclosed by it. The formula to calculate the volume of a cone is:
$$V = \frac{1}{3} \pi r^2 h$$
Where:
( V ) is the volume of the cone
( \pi ) is a mathematical constant approximately equal to 3.14159
( r ) is the radius of the base of the cone
( h ) is the height of the cone
Example Equations
Here are a few example equations related to cones:
Equation for calculating the slant height of a cone: $$l = \sqrt{r^2 + h^2}$$
Equation for calculating the radius of a cone given its slant height and height: $$r = \sqrt{l^2 – h^2}$$
Equation for calculating the height of a cone given its volume and radius: $$h = \frac{3V}{\pi r^2}$$
Sphere
Solid Sphere
Introduction – A solid sphere is a three-dimensional geometric figure in which all points inside the sphere are at the same distance from its center. – It is a type of 3D shape known as a “sphere” with a uniform density throughout.
Characteristics – The solid sphere has a well-defined volume, surface area, and mass. – It is completely filled with matter.
Volume of Solid Sphere The formula to calculate the volume (\(V_s\)) of a solid sphere is given by: \[ V_s = \frac{4}{3} \pi r^3 \] Where: \(V_s\) = Volume of the solid sphere, \(\pi\) (\(\pi\)) ≈ 3.14159, \(r\) = Radius of the sphere.
Surface Area of Solid Sphere The formula to calculate the surface area (\(A_s\)) of a solid sphere is given by: \[ A_s = 4 \pi r^2 \]
Where: \(A_s\) = Surface area of the solid sphere, \(\pi\) (\(\pi\)) ≈ 3.14159, \(r\) = Radius of the sphere
Mass of Solid Sphere The mass (\(m_s\)) of a solid sphere can be calculated using the formula: \[ m_s = \text{Density} \times V_s \] Where: \(m_s\) = Mass of the solid sphere, \(\text{Density}\) = Density of the material making up the sphere (usually in \(kg/m^3\)), \(V_s\) = Volume of the solid sphere (calculated using the previous formula)
Hollow Sphere
Introduction – A hollow sphere is also a three-dimensional geometric figure, but unlike a solid sphere, it has an empty space inside. – It consists of an outer shell or surface with a certain thickness and an inner empty region.
Characteristics – The hollow sphere has a well-defined outer radius (\(R\)), inner radius (\(r\)), volume, surface area, and mass. – It is partially filled with matter, mainly in the form of the outer shell.
Volume of Hollow Sphere The formula to calculate the volume (\(V_h\)) of a hollow sphere is given by: \[ V_h = \frac{4}{3} \pi (R^3 – r^3) \] Where: – \(V_h\) = Volume of the hollow sphere, \(\pi\) (\(\pi\)) ≈ 3.14159, \(R\) = Outer radius of the sphere, \(r\) = Inner radius of the sphere
Surface Area of Hollow Sphere The formula to calculate the surface area (\(A_h\)) of a hollow sphere is given by: \[ A_h = 4 \pi (R^2 – r^2) \] Where: \(A_h\) = Surface area of the hollow sphere, \(\pi\) (\(\pi\)) ≈ 3.14159, \(R\) = Outer radius of the sphere, \(r\) = Inner radius of the sphere
Mass of Hollow Sphere The mass (\(m_h\)) of a hollow sphere can be calculated using the formula: \[ m_h = \text{Density} \times V_h \] Where: \(m_h\) = Mass of the hollow sphere, \(\text{Density}\) = Density of the material making up the sphere (usually in \(kg/m^3\)), \(V_h\) = Volume of the hollow sphere (calculated using the previous formula)
Hindi Version
Dig Deep
Topic Level Resources
Sub – Topics
Select A Topic
Topic:
Chapters Index
Select Another Chapter
[display-posts category=”CBSE 10 – Mathematics – Study – Premium” posts_per_page=”25″]
No Matter Where You Start Among Study, Assess & Interact. But Your Course Will Be Completed Successfully.
Welcome to The Right Mentor, your trusted source for educational insights. In this article, we delve into the transformative role of artificial intelligence (AI) in school education. Discover how AI is reshaping traditional classrooms, providing personalized learning experiences, and revolutionizing teaching methods. Read on to explore the incredible potential of AI in education and its impact on student engagement and academic outcomes.
Indian Political System
Notes On Indian Political System Useful for competitive Exams.
Welcome to The Right Mentor, your trusted source for exam preparation. In this article, we provide valuable notes on the Indian political system to help you excel in your Telangana TGT jobs exam. Gain a deep understanding of the structure, functions, and key features of the Indian political system, ensuring success in your exam. Read on to discover our comprehensive notes and ace your exam with confidence.
Introduction to the Indian Political System
Learn about the foundational aspects of the Indian political system. Understand the key principles of democracy, secularism, federalism, and social justice that underpin the Indian Constitution. Gain insights into the historical context and evolution of the Indian political system, including the framing of the Constitution.
Structure of the Indian Political System
Explore the structure of the Indian political system. Learn about the three branches of government: the Executive, the Legislative, and the Judiciary. Understand the roles and functions of the President, Prime Minister, Council of Ministers, Parliament, and the Supreme Court. Familiarize yourself with the hierarchy and powers of these institutions.
Functions of the Indian Political System
Delve into the functions of the Indian political system. Gain an understanding of the roles and responsibilities of the Executive, Legislative, and Judiciary branches. Explore how laws are made, implemented, and interpreted in the Indian context. Learn about the significance of checks and balances in maintaining the democratic fabric of the country.
Key Features of the Indian Political System
Discover the key features that define the Indian political system. Learn about universal adult suffrage, federalism, fundamental rights, and the role of political parties in the Indian democracy. Understand the importance of free and fair elections, independent institutions, and the representation of diverse communities in shaping the Indian political landscape.
Recent Developments and Current Affairs
Stay updated with recent developments and current affairs related to the Indian political system. Explore significant political events, government policies, and constitutional amendments that have shaped the country’s political landscape. Keep track of major political parties, their ideologies, and their roles in national and state-level politics.
Prepare effectively for your Telangana TGT jobs exam with our comprehensive notes on the Indian political system. Gain a solid understanding of the structure, functions, and key features of the Indian political system to excel in your exam. Visit The Right Mentor for more valuable exam preparation resources and stay ahead in your journey towards success.
No Matter Where You Start Among Study, Assess & Interact. But Your Course Will Be Completed Successfully.
Welcome to The Right Mentor, your trusted source for educational insights. In this article, we delve into the transformative role of artificial intelligence (AI) in school education. Discover how AI is reshaping traditional classrooms, providing personalized learning experiences, and revolutionizing teaching methods. Read on to explore the incredible potential of AI in education and its impact on student engagement and academic outcomes.
Indian Political System – Structure
Notes on the Indian political system to help you excel in Competitive exams.
Introduction to the Indian Political System:
The Indian political system is based on the principles of democracy,federalism, secularism, and social justice.
The Constitution of India is the supreme law of the land and provides the framework for the functioning of the political system.
Three Branches of Government:
The Indian political system consists of three branches of government: the Executive, the Legislative, and the Judiciary.
Each branch has distinct roles and functions, ensuring a system of checks and balances.
Executive Branch:
The Executive branch is responsible for implementing and enforcing laws.
It includes the President, who is the head of state, and the Prime Minister, who is the head of government.
The Council of Ministers assists the Prime Minister in the administration of the country.
Legislative Branch:
The Legislative branch is responsible for making laws.
It consists of the Parliament, which is further divided into two houses: the Rajya Sabha (Council of States) and the Lok Sabha (House of the People).
Members of Parliament (MPs) are elected by the people and represent their respective constituencies.
Judiciary:
The Judiciary is the guardian of the Constitution and ensures the rule of law.
The Supreme Court is the highest judicial authority in the country.
It interprets the Constitution, resolves disputes, and safeguards fundamental rights.
Hierarchy and Powers of Institutions:
The President is the head of the state and exercises ceremonial powers.
The Prime Minister is the head of the government and holds executive powers.
The Parliament has the power to make laws, approve budgets, and oversee the functioning of the government.
The Supreme Court has the authority to interpret the Constitution, protect fundamental rights, and provide justice.
Federal Structure:
India follows a federal system where powers are divided between the central government and the state governments.
The central government is responsible for national-level matters, while the state governments handle state-level affairs.
Local self-government bodies, such as Panchayats and Municipalities, exist at the grassroots level.
Significance of the Structure:
The structure of the Indian political system ensures a separation of powers, preventing the concentration of authority in a single entity.
It promotes accountability, transparency, and democratic governance.
The hierarchy and powers of institutions create a system where each branch has its own roles and functions.
Note: It is advisable to refer to official textbooks, previous question papers, and updated syllabus provided by the Telangana TGT jobs exam conducting authority for comprehensive and accurate information.
No Matter Where You Start Among Study, Assess & Interact. But Your Course Will Be Completed Successfully.
Welcome to The Right Mentor, your trusted source for educational insights. In this article, we delve into the transformative role of artificial intelligence (AI) in school education. Discover how AI is reshaping traditional classrooms, providing personalized learning experiences, and revolutionizing teaching methods. Read on to explore the incredible potential of AI in education and its impact on student engagement and academic outcomes.
Welcome to The Right Mentor, your trusted source for exam preparation. In this article, we provide valuable notes on the Indian political system to help you excel in your Telangana TGT jobs exam. Gain a deep understanding of the structure, functions, and key features of the Indian political system, ensuring success in your exam. Read on to discover our comprehensive notes and ace your exam with confidence.
Content
Prepare effectively for your Telangana TGT jobs exam with our comprehensive notes on the Indian political system. Gain a solid understanding of the structure, functions, and key features of the Indian political system to excel in your exam. Visit The Right Mentor for more valuable exam preparation resources and stay ahead in your journey towards success.
No Matter Where You Start Among Study, Assess & Interact. But Your Course Will Be Completed Successfully.
Welcome to The Right Mentor, your trusted source for exam preparation. In this article, we provide comprehensive notes on the functions of the Indian political system, designed to help you succeed in your Telangana TGT jobs exam. Gain a deep understanding of the roles and responsibilities of the Executive, Legislative, and Judicial branches of the Indian political system. Read on to discover our valuable notes and prepare with confidence for your exam.,
Indian Political System – Functions
Notes on the functions of Indian political system to help you excel in Competitive exams.
Familiarize yourself with the Indian political system and its core principles. Understand the foundational aspects of democracy, secularism, federalism, and social justice that shape the Indian Constitution. Gain insights into the historical background and the evolution of the Indian political system, including the framing of the Constitution.
Functions of the Executive Branch
Explore the functions of the Executive branch in the Indian political system. Learn about the roles and responsibilities of the President, who is the head of state, and the Prime Minister, who is the head of government. Understand how the Council of Ministers assists in the execution and administration of government policies.
Functions of the Legislative Branch
Delve into the functions of the Legislative branch in the Indian political system. Learn about the roles and responsibilities of the Parliament, which consists of the Rajya Sabha (Council of States) and the Lok Sabha (House of the People). Understand how laws are made, amended, and repealed through the legislative process.
Functions of the Judicial Branch
Understand the functions of the Judicial branch in the Indian political system. Explore the role of the Supreme Court as the highest court of the land. Learn about the judiciary’s responsibility in upholding the Constitution, interpreting laws, and safeguarding the rights and freedoms of citizens.
Checks and Balances in the Indian Political System
Discover the significance of checks and balances in the Indian political system. Understand how the Executive, Legislative, and Judicial branches work together to ensure accountability and prevent the concentration of power. Explore the role of independent institutions, such as the Election Commission and the Comptroller and Auditor General, in maintaining the democratic fabric of the country.
Conclusion: Prepare effectively for your Telangana TGT jobs exam with our comprehensive notes on the functions of the Indian political system. Gain a thorough understanding of the roles and responsibilities of the Executive, Legislative, and Judicial branches to excel in your exam. Visit The Right Mentor for more valuable exam preparation resources and confidently prepare for success.
The Constitution of India is the supreme law of the land and provides the framework for the functioning of the political system.
No Matter Where You Start Among Study, Assess & Interact. But Your Course Will Be Completed Successfully.
Welcome to The Right Mentor, your trusted source for educational insights. In this article, we delve into the transformative role of artificial intelligence (AI) in school education. Discover how AI is reshaping traditional classrooms, providing personalized learning experiences, and revolutionizing teaching methods. Read on to explore the incredible potential of AI in education and its impact on student engagement and academic outcomes.
Welcome to The Right Mentor, your trusted source for exam preparation. In this article, we provide valuable notes on the Indian political system to help you excel in your Telangana TGT jobs exam. Gain a deep understanding of the structure, functions, and key features of the Indian political system, ensuring success in your exam. Read on to discover our comprehensive notes and ace your exam with confidence.
Federal System
India follows a federal system of government where power is divided between the central government and the state governments. This division of power ensures a balance between the central authority and regional autonomy.
Parliamentary Democracy
India has a parliamentary form of government where the President is the head of state and the Prime Minister is the head of government. The Parliament consists of the President, the Rajya Sabha (Council of States), and the Lok Sabha (House of the People).
Written Constitution
India has a written constitution that lays down the fundamental rights, principles, and structure of the government. The Constitution of India is one of the lengthiest and most comprehensive constitutions in the world.
Fundamental Rights
The Indian Constitution guarantees fundamental rights to its citizens, such as the right to equality, freedom of speech and expression, and the right to protection of life and personal liberty. These rights are enforceable by the judiciary.
Independent Judiciary
The Indian political system has an independent judiciary that acts as the guardian of the Constitution and ensures the rule of law. The Supreme Court is the highest judicial authority in the country.
Universal Adult Suffrage
India follows the principle of universal adult suffrage, where every citizen above the age of 18 has the right to vote. Elections are held periodically at the national, state, and local levels to elect representatives to the Parliament and state legislatures.
Secularism
India is a secular country, which means that the state does not promote or favor any particular religion. It guarantees freedom of religion and ensures equal treatment of all religions.
Multi-Party System
India has a multi-party system where multiple political parties compete in elections. The diversity of political parties allows for a wide range of representation and promotes democratic participation.
Independent Election Commission
The Election Commission of India is an autonomous body responsible for conducting free and fair elections in the country. It ensures the integrity of the electoral process and regulates political parties.
Reserved Seats
The Indian political system includes reserved seats for scheduled castes and scheduled tribes in the Parliament and state legislatures to ensure representation and participation of marginalized communities.
Understanding the key features of the Indian political system is essential for a comprehensive understanding of the country’s governance structure and democratic processes. These features reflect the principles of federalism, democracy, rule of law, and inclusivity that form the foundation of the Indian political system.
Prepare effectively for your Telangana TGT jobs exam with our comprehensive notes on the Indian political system. Gain a solid understanding of the structure, functions, and key features of the Indian political system to excel in your exam. Visit The Right Mentor for more valuable exam preparation resources and stay ahead in your journey towards success.
